No CrossRef data available.
Published online by Cambridge University Press: 24 October 2008
The fundamental group G of a μ-component link has the properties:
(1) G is finitely presented, with deficiency 1;
(2) G/G′ is free abelian on μ distinguished generators, say {t1 …, tμ}.
Let ψ: G → G/G′ → 〈t〉 be the composition of the canonical projection G → G/G′ and the epimorphism  defined by
defined by 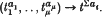 . Then the Z〈t〉-module M = Ker ψ/(Ker ψ)′ (the so-called module of the link) has a square (say n × n) relation matrix N. We write [N] for the Z〈t〉-equivalence class of N (Fox [3], p. 199) and N′̅ for the conjugate transpose of N.
. Then the Z〈t〉-module M = Ker ψ/(Ker ψ)′ (the so-called module of the link) has a square (say n × n) relation matrix N. We write [N] for the Z〈t〉-equivalence class of N (Fox [3], p. 199) and N′̅ for the conjugate transpose of N.