No CrossRef data available.
Article contents
RAMANUJAN SERIES WITH A SHIFT
Published online by Cambridge University Press: 23 October 2018
Abstract
We consider an extension of the Ramanujan series with a variable  $x$. If we let
$x$. If we let  $x=x_{0}$, we call the resulting series ‘Ramanujan series with the shift
$x=x_{0}$, we call the resulting series ‘Ramanujan series with the shift 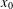 $x_{0}$’. Then we relate these shifted series to some
$x_{0}$’. Then we relate these shifted series to some  $q$-series and solve the case of level
$q$-series and solve the case of level 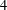 $4$ with the shift
$4$ with the shift  $x_{0}=1/2$. Finally, we indicate a possible way towards proving some patterns observed by the author corresponding to the levels
$x_{0}=1/2$. Finally, we indicate a possible way towards proving some patterns observed by the author corresponding to the levels  $\ell =1,2,3$ and the shift
$\ell =1,2,3$ and the shift 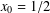 $x_{0}=1/2$.
$x_{0}=1/2$.
- Type
- Research Article
- Information
- Journal of the Australian Mathematical Society , Volume 107 , Issue 3 , December 2019 , pp. 367 - 380
- Copyright
- © 2018 Australian Mathematical Publishing Association Inc.
References
Borwein, J. M., Glasser, M. L., McPhedran, R. C., Wan, J. G. and Zucker, I. J., Lattice Sums Then and Now, Encyclopedia of Mathematics and its Applications, 150 (Cambridge University Press, Cambridge, 2013).Google Scholar
Chan, H. H., Chan, S. H. and Liu, Z., ‘Domb’s numbers and Ramanujan–Sato type series for 1/𝜋’, Adv. Math.
186 (2004), 396–410.Google Scholar
Glasser, M. L. and Zucker, I. J., ‘Lattice sums’, in: Theoretical Chemistry: Advances and Perspectives, Vol. 5 (eds. Eyring, H. and Henderson, D.) (Academic Press, New York, 1980), 67–139.Google Scholar
Guillera, J., ‘Series closely related to Ramanujan formulas for Pi’, unpublished paper (8 pages), 2003.Google Scholar
Guillera, J., ‘Series de Ramanujan: generalizaciones y conjeturas’, PhD Thesis, Universidad de Zaragoza, Spain, 2007.Google Scholar
Guillera, J., ‘A matrix form of Ramanujan-type series for 1/𝜋’, in: Gems in Experimental Mathematics, Contemporary Mathematics, 517 (eds. Amdeberhan, T., Medina, L. A. and Moll, V. H.) (American Mathematical Society, Washington, DC, 2010), 189–206.Google Scholar
Guillera, J., ‘WZ-proofs of ‘divergent’ Ramanujan-type series’, in: Waterloo Workshop in Computer Algebra in Memory of Herbert S. Wilf, Advances in Combinatorics (eds. Kotsireas, I. and Zima, E. V.) (Springer, Berlin–Heidelberg, 2013), 187–195.Google Scholar
Guillera, J. and Rogers, M., ‘Ramanujan series upside-down’, J. Aust. Math. Soc.
97 (2014), 78–106.Google Scholar
Petkovs̆ek, M., Wilf, H. S. and Zeilberger, D.,
A = B
(A.K. Peters, Natick, MA, 1996).Google Scholar
Sloane, N. J. A., The On-Line Encyclopedia of Integer Sequences (OEIS), founded in 1964,https://oeis.org.Google Scholar
Yang, Y., ‘Apèry limits and special values of L-functions’, J. Math. Anal. Appl.
343 (2008), 492–513.Google Scholar


