Published online by Cambridge University Press: 12 March 2014
Consider any interpreted theory Θ, formulated in the notation of quantification theory (or lower predicate calculus) with interpreted predicate letters. It will be proved that Θ is translatable into a theory, likewise formulated in the notation of quantification theory, in which there is only one predicate letter, and it a dyadic one.
Let us assume a fragment of set theory, adequate to assure the existence, for all x and y without regard to logical type, of the set {x, y) whose members are x and y, and to assure the distinctness of x from {x, y} and {{x}}. ({x} is explained as {x, x}.) Let us construe the ordered pair x; y in Kuratowski's fashion, viz. as {{x}, {x, y}}, and then construe x;y;z as x;(y;z), and x;y;z;w as x;(y;z;w), and so on. Let us refer to w, w;w, w;w;w, etc. as 1w, 2w, 3w, etc.
Suppose the predicates of Θ are ‘F1’, ‘F2’, …, finite or infinite in number, and respectively d1-adic, d2-adic, …. Now let Θ′ be a theory whose notation consists of that of quantification theory with just the single dyadic predicate ‘F’, interpreted thus:
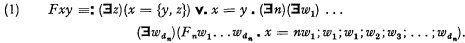
The universe of Θ′ is to comprise all objects of the universe of Θ and, in addition, {x, y) for every x and y in the universe of Θ′. (Of course the universe of Θ may happen already to comprise all this.)
Now I shall show how the familiar notations ‘x = y’, ‘x = {y, z}’, etc., and ultimately the desired ‘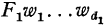 ’, ‘
’, ‘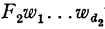 ’, etc. themselves can all be defined within Θ′.
’, etc. themselves can all be defined within Θ′.
1 This result is reminiscent of, but distinct from, two others in the literature. One is Kalmár's, which differs in that it has to do not with the translatability of an interpreted theory, but with the likeness of quantificational schemata in point of consistency or inconsistency. The other is that of Church, Craig, and Quine, which assures reducibility not merely to a dyadic predicate but to a symmetric one; it differs from the present result in supposing the universe to consist of natural numbers. See Kalmár, László, Zurückführung des Entscheidungsproblems auf den Fall von Formeln mit einer einzigen, binären, Funktionsvariablen, Compositio mathematica, vol. 4 (1936), pp. 137–144Google Scholar; Church, Alonzo and Quine, W. V., Some theorems on definability and decidability, this Journal, vol. 17 (1952), pp. 179–187Google Scholar; William Craig and W. V. Quine, On reduction to a symmetric relation, ibid., p. 188.
2 The preceding definition of ‘x = y’ applies only to simple variables, and thus does not overlap the present definition or succeeding ones.