Article contents
Model companions of distributive p-algebras
Published online by Cambridge University Press: 12 March 2014
Extract
Let Bn, 0 ≤ n ≤ ω, be the equational classes of distributive p-algebras (precise definitions are given in §1). It has been known for some time that the elementary theories Tn of Bn possess model companions 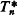 ; see, e.g., [6] and [14] and the references given there. However, no axiomatizations of
; see, e.g., [6] and [14] and the references given there. However, no axiomatizations of 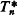 were given, with the exception of n = 0 (Boolean case) and n= 1 (Stonian case). While the first case belongs to the folklore of the subject (see [6], also [11]), the second case presented considerable difficulties (see Schmitt [13]). Schmitt's use of methods characteristic for Stone algebras seems to prevent a ready adaptation of his results to the cases n ≥ 2.
were given, with the exception of n = 0 (Boolean case) and n= 1 (Stonian case). While the first case belongs to the folklore of the subject (see [6], also [11]), the second case presented considerable difficulties (see Schmitt [13]). Schmitt's use of methods characteristic for Stone algebras seems to prevent a ready adaptation of his results to the cases n ≥ 2.
The natural way to get a hold on 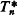 is to determine the class E(Bn) of existentially complete members of Bn: Since
is to determine the class E(Bn) of existentially complete members of Bn: Since 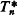 exists, it equals the elementary theory of E(Bn). The present author succeeded [12] in solving the simpler problem of determining the classes A(Bn) of algebraically closed algebras in Bn (exact definitions of A(Bn) and E(Bn) are given in §1) for all 0 > n < ω. A(Bn) is easier to handle since it contains sufficiently many “small” algebras-viz. finite direct products of certain subdirectly irreducibles-in terms of which the members of A(Bn) may be analyzed (in contrast, all members of E(Bn) are infinite and ℵ-homogeneous). As it turns out, A(Bn) is finitely axiomatizable for all n, and comparing the theories of A(B0), A(B1) with the explicitly known theories of E(B0), E(B1)-viz.
exists, it equals the elementary theory of E(Bn). The present author succeeded [12] in solving the simpler problem of determining the classes A(Bn) of algebraically closed algebras in Bn (exact definitions of A(Bn) and E(Bn) are given in §1) for all 0 > n < ω. A(Bn) is easier to handle since it contains sufficiently many “small” algebras-viz. finite direct products of certain subdirectly irreducibles-in terms of which the members of A(Bn) may be analyzed (in contrast, all members of E(Bn) are infinite and ℵ-homogeneous). As it turns out, A(Bn) is finitely axiomatizable for all n, and comparing the theories of A(B0), A(B1) with the explicitly known theories of E(B0), E(B1)-viz.  ,
, 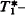 , a reasonable conjecture for
, a reasonable conjecture for 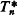 , 2 ≤ n ≤ ω, is immediate. The main part of this paper is concerned with verifying that the conditions formalized by
, 2 ≤ n ≤ ω, is immediate. The main part of this paper is concerned with verifying that the conditions formalized by 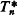 suffice to describe the algebras in E(Bn) (necessity is easy). This verification rests on the same combinatorial techniques as used in [12] to describe the members of A(Bn).
suffice to describe the algebras in E(Bn) (necessity is easy). This verification rests on the same combinatorial techniques as used in [12] to describe the members of A(Bn).
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1982
References
REFERENCES
- 9
- Cited by


