Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-26T19:11:03.889Z Has data issue: false hasContentIssue false
  • English
  • Français

Comparing the atomic and macroscopic aging dynamics in an amorphous and partially crystalline Zr44Ti11Ni10Cu10Be25 bulk metallic glass

Published online by Cambridge University Press:  22 May 2017

Zach Evenson Open the ORCID record for Zach Evenson [Opens in a new window] ,
Alba Payes-Playa ,
Yuriy Chushkin ,
Marco di Michiel ,
Eloi Pineda  and
Beatrice Ruta
Zach Evenson*
Affiliation:
Heinz Maier-Leibnitz Zentrum (MLZ) and Physik Department, Technische Universität München, Garching 85748, Germany
Alba Payes-Playa
Affiliation:
ESRF—The European Synchrotron, Grenoble 38043, France; and Universidad Autonoma de Madrid, Ciudad Universitaria de Cantoblanco, Madrid 28049, Spain
Yuriy Chushkin
Affiliation:
ESRF—The European Synchrotron, Grenoble 38043, France
Marco di Michiel
Affiliation:
ESRF—The European Synchrotron, Grenoble 38043, France
Eloi Pineda
Affiliation:
Departament de Física, Universitat Politècnica Catalunya—BarcelonaTech, ESAB, Castelldefels 08860, Spain
Beatrice Ruta*
Affiliation:
ESRF—The European Synchrotron, Grenoble 38043, France; and Institute of Light and Matter, UMR5306 Université Lyon 1-CNRS, Université de Lyon, Villeurbanne Cedex 69622, France
*
a)Address all correspondence to these authors. e-mail: [email protected]
b)e-mail: [email protected]
Get access

Abstract

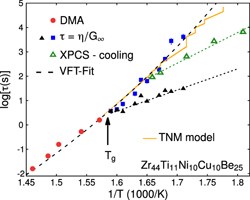
Several recent X-ray photon correlation spectroscopy works have reported an anomalous atomic dynamics in hyperquenched metallic glasses. Here, we compare and contrast these microscopic dynamics with that found in a Zr44Ti11Ni10Cu10Be25 bulk metallic glass, prepared with a cooling rate some 6 orders of magnitude lower. In both cases, structural relaxation in the glass is governed by internal stresses, giving rise to highly compressed density correlation functions. Differently from the fast aging reported in previous studies, here the atomic dynamics displays a slow linear atomic-level aging, while not affecting the shape parameter. Traditional macroscopic phenomenological models fail to capture the temperature dependence of the microscopic structural relaxation time, suggesting a length scale dependence of the aging. Interestingly, the dynamics does not seem to be affected by the presence of a low percentage of frozen nanocrystals and displays a temperature dependence similar to that observed in macroscopic viscosity measurements.

Keywords

amorphousannealingalloy
Type
Invited Papers
Information
Journal of Materials Research , Volume 32 , Issue 11 , 14 June 2017 , pp. 2014 - 2021
Check for updates
Copyright
Copyright © Materials Research Society 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Contributing Editor: Jürgen Eckert

References

REFERENCES

Lubchenko, V. and Wolynes, P.G.: Theory of aging in structural glasses. J. Chem. Phys. 121, 2852 (2004).Google Scholar
Berthier, L. and Biroli, G.: Theoretical perspective on the glass transition and amorphous materials. Rev. Mod. Phys. 83, 587 (2011).Google Scholar
Zhao, J., Simon, S.L., and McKenna, G.B.: Using 20-million-year-old amber to test the super-Arrhenius behaviour of glass-forming systems. Nat. Commun. 4, 1783 (2013).Google Scholar
Mauro, J.C., Allan, D., and Potuzak, M.: Nonequilibrium viscosity of glass. Phys. Rev. B: Condens. Matter Mater. Phys. 80, 1 (2009).Google Scholar
Launey, M.E., Busch, R., and Kruzic, J.J.: Influence of structural relaxation on the fatigue behavior of a ZrTiNiCuBe bulk amorphous alloy. Scr. Mater. 54, 483 (2006).Google Scholar
Evenson, Z., Koschine, T., Wei, S., Gross, O., Bednarcik, J., Gallino, I., Kruzic, J.J., Rätzke, K., Faupel, F., and Busch, R.: The effect of low-temperature structural relaxation on free volume and chemical short-range ordering in a Au49Cu26.9Si16.3Ag5.5Pd2.3 bulk metallic glass. Scr. Mater. 103, 14 (2015).Google Scholar
Evenson, Z., Naleway, S.E., Wei, S., Gross, O., Kruzic, J.J., Gallino, I., Possart, W., Stommel, M., and Busch, R.: β relaxation and low-temperature aging in a Au-based bulk metallic glass: From elastic properties to atomic-scale structure. Phys. Rev. B: Condens. Matter Mater. Phys. 89, 174204 (2014).Google Scholar
Ruta, B., Chushkin, Y., Monaco, G., Cipelletti, L., Pineda, E., Bruna, P., Giordano, V.M., and Gonzalez-Silveira, M.: Atomic-scale relaxation dynamics and aging in a metallic glass probed by X-ray photon correlation spectroscopy. Phys. Rev. Lett. 109, 165701 (2012).Google Scholar
Leitner, M., Sepiol, B., Stadler, L-M., and Pfau, B.: Time-resolved study of the crystallization dynamics in a metallic glass. Phys. Rev. B: Condens. Matter Mater. Phys. 86, 64202 (2012).Google Scholar
Ruta, B., Baldi, G., Monaco, G., and Chushkin, Y.: Compressed correlation functions and fast aging dynamics in metallic glasses. J. Chem. Phys. 138, 54508 (2013).Google Scholar
Evenson, Z., Ruta, B., Hechler, S., Stolpe, M., Pineda, E., Gallino, I., and Busch, R.: X-ray photon correlation spectroscopy reveals intermittent aging dynamics in a metallic glass. Phys. Rev. Lett. 115, 175701 (2015).Google Scholar
Wang, X.D., Ruta, B., Xiong, L.H., Zhang, D.W., Chushkin, Y., Sheng, H.W., Lou, H.B., Cao, Q.P., and Jiang, J.Z.: Free-volume dependent atomic dynamics in beta relaxation pronounced La-based metallic glasses. Acta Mater. 99, 290 (2015).Google Scholar
Giordano, V.M. and Ruta, B.: Unveiling the structural arrangements responsible for the atomic dynamics in metallic glasses during physical aging. Nat. Commun. 7, 1 (2015).Google Scholar
Radelaar, S. and van den Beukel, A.: On the kinetics of structural relaxation in metallic glasses. Acta Metall. 31, 419 (1983).Google Scholar
Slipenyuk, A. and Eckert, J.: Correlation between enthalpy change and free volume reduction during structural relaxation of Zr55Cu30Al10Ni5 metallic glass. Scr. Mater. 50, 39 (2004).Google Scholar
Evenson, Z. and Busch, R.: Equilibrium viscosity, enthalpy recovery and free volume relaxation in a Zr44Ti11Ni10Cu10Be25 bulk metallic glass. Acta Mater. 59, 4404 (2011).Google Scholar
Waniuk, T.A., Busch, R., Masuhr, A., and Johnson, W.L.: Viscosity of the Zr41.2Ti13.8Cu12.5Ni10Be22.5 bulk metallic glass-forming liquid and viscous flow during relaxation, phase separation, and primary crystallization. Acta Mater. 46, 5229 (1998).Google Scholar
Hays, C.C., Kim, C.P., and Johnson, W.L.: Large supercooled liquid region and phase separation in the Zr–Ti–Ni–Cu–Be bulk metallic glasses. Appl. Phys. Lett. 75, 1089 (1999).Google Scholar
Evenson, Z., Raedersdorf, S., Gallino, I., and Busch, R.: Equilibrium viscosity of Zr–Cu–Ni–Al–Nb bulk metallic glasses. Scr. Mater. 63, 573 (2010).Google Scholar
Waniuk, T.A.: Viscosity and crystallization in a series of Zr-Based bulk amorphous alloys. Ph.D. thesis, California Institute of Technology, 2004.Google Scholar
Chushkin, Y., Caronna, C., and Madsen, A.: A novel event correlation scheme for X-ray photon correlation spectroscopy. J. Appl. Crystallogr. 45, 807 (2012).Google Scholar
Madsen, A., Fluerasu, A., and Ruta, B.: Synchrotron Light Sources and Free-Electron Lasers (Springer, Cham, 2015); pp. 1–21.Google Scholar
Kohlrausch, R.: Theorie des elektrischen Rückstandes in der Leidener Flasche [Theory of electric residue in the Leyden jar]. Ann. Phys. 167, 56 (1854).Google Scholar
Williams, G. and Watts, C.: Non-symmetrical dielectric relaxation behaviour arising from a simple empirical decay function. Trans. Faraday Soc. 66, 80 (1970).Google Scholar
Ediger, M.D.: Spatially heterogeneous dynamics in supercooled liquids. Annu. Rev. Phys. Chem. 51, 99 (2000).Google Scholar
Bouchaud, J-P. and Pitard, E.: Anomalous dynamical light scattering in soft glassy gels. Eur. Phys. J. E: Soft Matter Biol. Phys. 6, 231 (2001).Google Scholar
Cipelletti, L., Ramos, L., Manley, S., Pitard, E., Weitz, D.A., Pashkovski, E.E., and Johansson, M.: Universal non-diffusive slow dynamics in aging soft matter. Faraday Discuss. 123, 237 (2003).Google Scholar
Ferrero, E.E., Martens, K., and Barrat, J-L.: Relaxation in yield stress systems through elastically interacting activated events. Phys. Rev. Lett. 113, 248301 (2014).Google Scholar
Chaudhuri, P. and Berthier, L.: Ultra-long-range dynamic correlations in a microscopic model for aging gels. arXiv:1605.09770 (2016).Google Scholar
Lemaître, A.: Structural relaxation is a scale-free process. Phys. Rev. Lett. 113, 245702 (2014).Google Scholar
Lemaître, A.: Tensorial analysis of Eshelby stresses in 3D supercooled liquids. J. Chem. Phys. 143, 164515 (2015).Google Scholar
Fan, Y., Iwashita, T., and Egami, T.: Crossover from localized to cascade relaxations in metallic glasses. Phys. Rev. Lett. 115, 45501 (2015).Google Scholar
Meyer, A., Wuttke, J., Petry, W., Peker, A., Bormann, R., Coddens, G., Kranich, L., Randl, O., and Schober, H.: Harmonic behavior of metallic glasses up to the metastable melt. Phys. Rev. B: Condens. Matter Mater. Phys. 53, 12107 (1996).Google Scholar
Meyer, A., Petry, W., Koza, M., and Macht, M-P.: Fast diffusion in ZrTiCuNiBe melts. Appl. Phys. Lett. 83, 3894 (2003).Google Scholar
Yang, F., Kordel, T., Holland-Moritz, D., Unruh, T., and Meyer, A.: Structural relaxation as seen by quasielastic neutron scattering on viscous Zr–Ti–Cu–Ni–Be droplets. J. Phys.: Condens. Matter 23, 254207 (2011).Google Scholar
Zhao, Z.F., Wen, P., Shek, C.H., and Wang, W.H.: Measurements of slow β-relaxations in metallic glasses and supercooled liquids. Phys. Rev. B: Condens. Matter Mater. Phys. 75, 174201 (2007).Google Scholar
Debenedetti, P.G. and Stillinger, F.H.: Supercooled liquids and the glass transition. Nature 410, 259 (2001).Google Scholar
Heuer, A.: Exploring the potential energy landscape of glass-forming systems: From inherent structures via metabasins to macroscopic transport. J. Phys.: Condens. Matter 20, 373101 (2008).Google Scholar
Tool, A.Q.: Relation between inelastic deformability and thermal expansion of glass in its annealing range. J. Am. Chem. Soc. 29, 240 (1946).Google Scholar
Narayanaswamy, O.S.: A model of structural relaxation in glass. J. Am. Ceram. Soc. 54, 491 (1971).Google Scholar
Moynihan, C.T., Macedo, P.B., Montrose, C.J., Gupta, P.K., DeBolt, M.A., Dill, J.F., Dom, B.E., Drake, P.W., Easteal, A.J., Elterman, P.B., Moeller, R.P., Sasabe, H., and Wilder, J.A.: Structural relaxation in vitreous materials. Ann. N. Y. Acad. Sci. 279, 15 (1976).Google Scholar
Maxwell, J.C.: On the dynamical theory of gases. Philos. Trans. R. Soc. London 157, 49 (1867).Google Scholar
Lind, M.L., Duan, G., and Johnson, W.L.: Isoconfigurational elastic constants and liquid fragility of a bulk metallic glass forming alloy. Phys. Rev. Lett. 97, 15501 (2006).Google Scholar
Johnson, W.L., Demetriou, M.D., Harmon, J.S., Lind, M.L., and Samwer, K.: Rheology and properties of metallic glass-forming liquids: A potential energy landscape perspective. MRS Bull. 32, 644 (2007).Google Scholar
Khonik, V.A., Mitrofanov, Y.P., Khonik, S.V., and Saltykov, S.N.: Unexpectedly large relaxation time determined by in situ high-frequency shear modulus measurements near the glass transition of bulk glassy Pd40Cu30Ni10P20 . J. Non-Cryst. Solids 356, 1191 (2010).Google Scholar
Vogel, H.: The law of the relationship between viscosity of liquids and the temperature. Phys. Z. 22, 645 (1921).Google Scholar
Fulcher, G.: Analysis of recent measurements of the viscosity of glasses. J. Am. Ceram. Soc. 8, 339 (1925).Google Scholar
Tammann, G. and Hesse, W.: Die Abhängigkeit der Viscosität von der Temperatur bei unterkühlten Flüssigkeiten [The dependence of viscosity on temperature in undercooled liquids]. Z. Anorg. Allg. Chem. 156, 245 (1926).Google Scholar
Vilgis, T.A.: Strong and fragile glasses: A powerful classification and its consequences. Phys. Rev. B: Condens. Matter Mater. Phys. 47, 2882 (1993).Google Scholar
Böhmer, R., Ngai, K.L., Angell, C.A., and Plazek, D.J.: Nonexponential relaxations in strong and fragile glass formers. J. Chem. Phys. 99, 4201 (1993).Google Scholar
Hodge, I.M.: Enthalpy relaxation and recovery in amorphous materials. J. Non-Cryst. Solids 169, 211 (1994).Google Scholar
Scherer, G.W.: Use of the Adam–Gibbs equation in the analysis of structural relaxation. J. Am. Ceram. Soc. 67, 504 (1984).Google Scholar
Hodge, I.M.: Adam–Gibbs formulation of enthalpy relaxation near the glass transition. J. Res. Natl. Inst. Stand. Technol. 102, 195 (1997).Google Scholar
Ruta, B., Baldi, G., Chushkin, Y., Rufflé, B., Cristofolini, L., Fontana, A., Zanatta, M., and Nazzani, F.: Revealing the fast atomic motion of network glasses. Nat. Commun. 5, 3939 (2014).Google Scholar
O’Reilly, J.M.: Review of structure and mobility in amorphous polymers. Crit. Rev. Solid State Mater. Sci. 13, 259 (1987).Google Scholar
Simon, S.L.: Enthalpy recovery of poly(ether imide): Experiment and model calculations incorporating thermal gradients. Macromolecules 9297, 4056 (1997).Google Scholar
Bernazzani, P. and Simon, S.L.: Volume recovery of polystyrene: Evolution of the characteristic relaxation time. J. Non-Cryst. Solids 307–310, 470 (2002).Google Scholar
Simon, S.L. and Bernazzani, P.: Structural relaxation in the glass: Evidence for a path dependence of the relaxation time. J. Non-Cryst. Solids 352, 4763 (2006).Google Scholar