Published online by Cambridge University Press: 09 January 2013
Using a slip-length-based level-set approach with adaptive mesh refinement, we have simulated axisymmetric droplet spreading for a dimensionless slip length down to  $O(1{0}^{\ensuremath{-} 4} )$. The main purpose is to validate, and where necessary improve, the asymptotic analysis of Cox (J. Fluid Mech., vol. 357, 1998, pp. 249–278) for rapid droplet spreading/dewetting, in terms of the detailed interface shape in various regions close to the moving contact line and the relation between the apparent angle and the capillary number based on the instantaneous contact-line speed,
$O(1{0}^{\ensuremath{-} 4} )$. The main purpose is to validate, and where necessary improve, the asymptotic analysis of Cox (J. Fluid Mech., vol. 357, 1998, pp. 249–278) for rapid droplet spreading/dewetting, in terms of the detailed interface shape in various regions close to the moving contact line and the relation between the apparent angle and the capillary number based on the instantaneous contact-line speed, 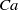 $\mathit{Ca}$. Before presenting results for inertial spreading, simulation results are compared in detail with the theory of Hocking & Rivers (J. Fluid Mech., vol. 121, 1982, pp. 425–442) for slow spreading, showing that these agree very well (and in detail) for such small slip-length values, although limitations in the theoretically predicted interface shape are identified; a simple extension of the theory to viscous exterior fluids is also proposed and shown to yield similar excellent agreement. For rapid droplet spreading, it is found that, in principle, the theory of Cox can predict accurately the interface shapes in the intermediate viscous sublayer, although the inviscid sublayer can only be well presented when capillary-type waves are outside the contact-line region. However,
$\mathit{Ca}$. Before presenting results for inertial spreading, simulation results are compared in detail with the theory of Hocking & Rivers (J. Fluid Mech., vol. 121, 1982, pp. 425–442) for slow spreading, showing that these agree very well (and in detail) for such small slip-length values, although limitations in the theoretically predicted interface shape are identified; a simple extension of the theory to viscous exterior fluids is also proposed and shown to yield similar excellent agreement. For rapid droplet spreading, it is found that, in principle, the theory of Cox can predict accurately the interface shapes in the intermediate viscous sublayer, although the inviscid sublayer can only be well presented when capillary-type waves are outside the contact-line region. However, 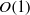 $O(1)$ parameters taken to be unity by Cox must be specified and terms be corrected to
$O(1)$ parameters taken to be unity by Cox must be specified and terms be corrected to 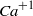 ${\mathit{Ca}}^{+ 1} $ in order to achieve good agreement between the theory and the simulation, both of which are undertaken here. We also find that the apparent angle from numerical simulation, obtained by extrapolating the interface shape from the macro region to the contact line, agrees reasonably well with the modified theory of Cox. A simplified version of the inertial theory is proposed in the limit of negligible viscosity of the external fluid. Building on these results, weinvestigate the flow structure near the contact line, the shear stress and pressure along the wall, and the use of the analysis for droplet impact and rapid dewetting. Finally, we compare the modified theory of Cox with a recent experiment for rapid droplet spreading, the results of which suggest a spreading-velocity-dependent dynamic contact angle in the experiments. The paper is closed with a discussion of the outlook regarding the potential of using the present results in large-scale simulations wherein the contact-line region is not resolved down to the slip length, especially for inertial spreading.
${\mathit{Ca}}^{+ 1} $ in order to achieve good agreement between the theory and the simulation, both of which are undertaken here. We also find that the apparent angle from numerical simulation, obtained by extrapolating the interface shape from the macro region to the contact line, agrees reasonably well with the modified theory of Cox. A simplified version of the inertial theory is proposed in the limit of negligible viscosity of the external fluid. Building on these results, weinvestigate the flow structure near the contact line, the shear stress and pressure along the wall, and the use of the analysis for droplet impact and rapid dewetting. Finally, we compare the modified theory of Cox with a recent experiment for rapid droplet spreading, the results of which suggest a spreading-velocity-dependent dynamic contact angle in the experiments. The paper is closed with a discussion of the outlook regarding the potential of using the present results in large-scale simulations wherein the contact-line region is not resolved down to the slip length, especially for inertial spreading.