Published online by Cambridge University Press: 22 June 2017
We analyse the low-frequency dynamics of a high Reynolds number impinging shock-wave/turbulent boundary-layer interaction (SWBLI) with strong mean-flow separation. The flow configuration for our grid-converged large-eddy simulations (LES) reproduces recent experiments for the interaction of a Mach 3 turbulent boundary layer with an impinging shock that nominally deflects the incoming flow by 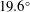 $19.6^{\circ }$. The Reynolds number based on the incoming boundary-layer thickness of
$19.6^{\circ }$. The Reynolds number based on the incoming boundary-layer thickness of  $Re_{\unicode[STIX]{x1D6FF}_{0}}\approx 203\times 10^{3}$ is considerably higher than in previous LES studies. The very long integration time of
$Re_{\unicode[STIX]{x1D6FF}_{0}}\approx 203\times 10^{3}$ is considerably higher than in previous LES studies. The very long integration time of  $3805\unicode[STIX]{x1D6FF}_{0}/U_{0}$ allows for an accurate analysis of low-frequency unsteady effects. Experimental wall-pressure measurements are in good agreement with the LES data. Both datasets exhibit the distinct plateau within the separated-flow region of a strong SWBLI. The filtered three-dimensional flow field shows clear evidence of counter-rotating streamwise vortices originating in the proximity of the bubble apex. Contrary to previous numerical results on compression ramp configurations, these Görtler-like vortices are not fixed at a specific spanwise position, but rather undergo a slow motion coupled to the separation-bubble dynamics. Consistent with experimental data, power spectral densities (PSD) of wall-pressure probes exhibit a broadband and very energetic low-frequency component associated with the separation-shock unsteadiness. Sparsity-promoting dynamic mode decompositions (SPDMD) for both spanwise-averaged data and wall-plane snapshots yield a classical and well-known low-frequency breathing mode of the separation bubble, as well as a medium-frequency shedding mode responsible for reflected and reattachment shock corrugation. SPDMD of the two-dimensional skin-friction coefficient further identifies streamwise streaks at low frequencies that cause large-scale flapping of the reattachment line. The PSD and SPDMD results of our impinging SWBLI support the theory that an intrinsic mechanism of the interaction zone is responsible for the low-frequency unsteadiness, in which Görtler-like vortices might be seen as a continuous (coherent) forcing for strong SWBLI.
$3805\unicode[STIX]{x1D6FF}_{0}/U_{0}$ allows for an accurate analysis of low-frequency unsteady effects. Experimental wall-pressure measurements are in good agreement with the LES data. Both datasets exhibit the distinct plateau within the separated-flow region of a strong SWBLI. The filtered three-dimensional flow field shows clear evidence of counter-rotating streamwise vortices originating in the proximity of the bubble apex. Contrary to previous numerical results on compression ramp configurations, these Görtler-like vortices are not fixed at a specific spanwise position, but rather undergo a slow motion coupled to the separation-bubble dynamics. Consistent with experimental data, power spectral densities (PSD) of wall-pressure probes exhibit a broadband and very energetic low-frequency component associated with the separation-shock unsteadiness. Sparsity-promoting dynamic mode decompositions (SPDMD) for both spanwise-averaged data and wall-plane snapshots yield a classical and well-known low-frequency breathing mode of the separation bubble, as well as a medium-frequency shedding mode responsible for reflected and reattachment shock corrugation. SPDMD of the two-dimensional skin-friction coefficient further identifies streamwise streaks at low frequencies that cause large-scale flapping of the reattachment line. The PSD and SPDMD results of our impinging SWBLI support the theory that an intrinsic mechanism of the interaction zone is responsible for the low-frequency unsteadiness, in which Görtler-like vortices might be seen as a continuous (coherent) forcing for strong SWBLI.
DMD analysis of spanwise-averaged snapshots. Flow modulation by means of mode Φ1. The animation shows contours of the pressure gradient magnitude in the range │∇p│δ0/p∞ = [0,10] at 8 equally spaced phase angles. The mean shock system together with the instantaneous separation bubble are highlighted by black solid lines.
DMD analysis of spanwise-averaged snapshots. Flow modulation by means of mode Φ2. The animation shows contours of the pressure gradient magnitude in the range │∇p│δ0/p∞ = [0,10] at 8 equally spaced phase angles. The mean shock system together with the instantaneous separation bubble are highlighted by black solid lines.
DMD analysis of spanwise-averaged snapshots. Flow modulation by means of mode Φ3. The animation shows contours of the pressure gradient magnitude in the range │∇p│δ0/p∞ = [0,10] at 8 equally spaced phase angles. The mean shock system together with the instantaneous separation bubble are highlighted by black solid lines.
MDMD analysis of spanwise-averaged snapshots. Flow modulation by means of mode Φ4. The animation shows contours of the pressure gradient magnitude in the range │∇p│δ0/p∞ = [0,10] at 8 equally spaced phase angles. The mean shock system together with the instantaneous separation bubble are highlighted by black solid lines.
DMD analysis of wall-plane snapshots. Flow modulation by means of mode Φ1. The animation shows contours of the two-dimensional skin-friction coefficient at 8 equally spaced phase angles. The instantaneous separation and reattachment locations are highlighted by black solid lines.
DMD analysis of wall-plane snapshots. Flow modulation by means of mode Φ2. The animation shows contours of the two-dimensional skin-friction coefficient at 8 equally spaced phase angles. The instantaneous separation and reattachment locations are highlighted by black solid lines.
DMD analysis of wall-plane snapshots. Flow modulation by means of mode Φ3. The animation shows contours of the two-dimensional skin-friction coefficient at 8 equally spaced phase angles. The instantaneous separation and reattachment locations are highlighted by black solid lines.
DMD analysis of wall-plane snapshots. Flow modulation by means of mode Φ3. The animation shows contours of the two-dimensional skin-friction coefficient at 8 equally spaced phase angles. The instantaneous separation and reattachment locations are highlighted by black solid lines.