Published online by Cambridge University Press: 10 May 2019
The influence of a cube-based canopy on coherent structures of the flow was investigated in a high Reynolds number boundary layer (thickness 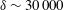 $\unicode[STIX]{x1D6FF}\sim 30\,000$ wall units). Wind tunnel experiments were conducted considering wall configurations that represent three idealised urban terrains. Stereoscopic particle image velocimetry was employed using a large field of view in a streamwise–spanwise plane (
$\unicode[STIX]{x1D6FF}\sim 30\,000$ wall units). Wind tunnel experiments were conducted considering wall configurations that represent three idealised urban terrains. Stereoscopic particle image velocimetry was employed using a large field of view in a streamwise–spanwise plane (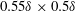 $0.55\unicode[STIX]{x1D6FF}\times 0.5\unicode[STIX]{x1D6FF}$) combined to two-point hot-wire measurements. The analysis of the flow within the inertial layer highlights the independence of its characteristics from the wall configuration. The population of coherent structures is in agreement with that of smooth-wall boundary layers, i.e. consisting of large- and very-large-scale motions, sweeps and ejections, as well as smaller-scale vortical structures. The characteristics of vortices appear to be independent of the roughness configuration while their spatial distribution is closely linked to large meandering motions of the boundary layer. The canopy geometry only significantly impacts the wall-normal exchanges within the roughness sublayer. Bi-dimensional spectral analysis demonstrates that wall-normal velocity fluctuations are constrained by the presence of the canopy for the densest investigated configurations. This threshold in plan area density above which large scales from the overlying boundary layer can penetrate the roughness sublayer is consistent with the change of the flow regime reported in the literature and constitutes a major difference with flows over vegetation canopies.
$0.55\unicode[STIX]{x1D6FF}\times 0.5\unicode[STIX]{x1D6FF}$) combined to two-point hot-wire measurements. The analysis of the flow within the inertial layer highlights the independence of its characteristics from the wall configuration. The population of coherent structures is in agreement with that of smooth-wall boundary layers, i.e. consisting of large- and very-large-scale motions, sweeps and ejections, as well as smaller-scale vortical structures. The characteristics of vortices appear to be independent of the roughness configuration while their spatial distribution is closely linked to large meandering motions of the boundary layer. The canopy geometry only significantly impacts the wall-normal exchanges within the roughness sublayer. Bi-dimensional spectral analysis demonstrates that wall-normal velocity fluctuations are constrained by the presence of the canopy for the densest investigated configurations. This threshold in plan area density above which large scales from the overlying boundary layer can penetrate the roughness sublayer is consistent with the change of the flow regime reported in the literature and constitutes a major difference with flows over vegetation canopies.