Hostname: page-component-745bb68f8f-kw2vx Total loading time: 0 Render date: 2025-01-10T01:13:48.287Z Has data issue: false hasContentIssue false
  • English
  • Français

Stability of a temporally evolving natural convection boundary layer on an isothermal wall

Published online by Cambridge University Press:  02 September 2019

Junhao Ke Open the ORCID record for Junhao Ke [Opens in a new window] ,
N. Williamson Open the ORCID record for N. Williamson [Opens in a new window] ,
S. W. Armfield Open the ORCID record for S. W. Armfield [Opens in a new window] ,
G. D. McBain Open the ORCID record for G. D. McBain [Opens in a new window]  and
S. E. Norris Open the ORCID record for S. E. Norris [Opens in a new window]
Junhao Ke*
Affiliation:
School of Aerospace, Mechanical and Mechatronic Engineering, The University of Sydney, New South Wales, 2006, Australia
N. Williamson
Affiliation:
School of Aerospace, Mechanical and Mechatronic Engineering, The University of Sydney, New South Wales, 2006, Australia
S. W. Armfield
Affiliation:
School of Aerospace, Mechanical and Mechatronic Engineering, The University of Sydney, New South Wales, 2006, Australia
G. D. McBain
Affiliation:
Memjet North Ryde Pty Ltd, Macquarie Park, New South Wales, 2113, Australia
S. E. Norris
Affiliation:
Department of Mechanical Engineering, The University of Auckland, Auckland, 1010, New Zealand
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The stability properties of a natural convection boundary layer adjacent to an isothermally heated vertical wall, with Prandtl number 0.71, are numerically investigated in the configuration of a temporally evolving parallel flow. The instantaneous linear stability of the flow is first investigated by solving the eigenvalue problem with a quasi-steady assumption, whereby the unsteady base flow is frozen in time. Temporal responses of the discrete perturbation modes are numerically obtained by solving the two-dimensional linearized disturbance equations using a ‘frozen’ base flow as an initial-value problem at various 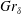$Gr_{\unicode[STIX]{x1D6FF}}$, where 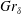$Gr_{\unicode[STIX]{x1D6FF}}$ is the Grashof number based on the velocity integral boundary layer thickness $\unicode[STIX]{x1D6FF}$. The resultant amplification rates of the discrete modes are compared with the quasi-steady eigenvalue analysis, and both two-dimensional and three-dimensional direct numerical simulations (DNS) of the temporally evolving flow. The amplification rate predicted by the linear theory compares well with the result of direct numerical simulation up to a transition point. The extent of the linear regime where the perturbations linearly interact with the base flow is thus identified. The value of the transition 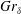$Gr_{\unicode[STIX]{x1D6FF}}$, according to the three-dimensional DNS results, is dependent on the initial perturbation amplitude. Beyond the transition point, the DNS results diverge from the linear stability predictions as nonlinear mechanisms become important.

JFM classification

Boundary Layers: Boundary layer stability Instability: Absolute/convective instability
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 877 , 25 October 2019 , pp. 1163 - 1185
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abedin, M. Z., Tsuji, T. & Hattori, Y. 2009 Direct numerical simulation for a time-developing natural-convection boundary layer along a vertical flat plate. Intl J. Heat Mass Transfer 52 (19-20), 4525–4534.10.1016/j.ijheatmasstransfer.2009.03.061Google Scholar
Aberra, T., Armfield, S. W., Behnia, M. & McBain, G. D. 2012 Boundary layer instability of the natural convection flow on a uniformly heated vertical plate. Intl J. Heat Mass Transfer 55 (21-22), 6097–6108.10.1016/j.ijheatmasstransfer.2012.06.023Google Scholar
Armfield, S. W. & Patterson, J. C. 1992 Wave properties of natural-convection boundary layers. J. Fluid Mech. 239, 195–211.10.1017/S0022112092004373Google Scholar
Brooker, A. M. H., Patterson, J. C., Graham, T. & Schöpf, W. 2000 Convective instability in a time-dependent buoyancy driven boundary layer. Intl J. Heat Mass Transfer 43 (2), 297–310.10.1016/S0017-9310(99)00127-1Google Scholar
Dring, R. P. & Gebhart, B. 1968 A theoretical investigation of disturbance amplification in external laminar natural convection. J. Fluid Mech. 34 (3), 551–564.10.1017/S0022112068002077Google Scholar
Dring, R. P. & Gebhart, B. 1969 An experimental investigation of disturbance amplification in external laminar natural convection flow. J. Fluid Mech. 36 (3), 447–464.10.1017/S0022112069001753Google Scholar
Eckert, E. R. G. 1951 Interferometric studies on the stability and transition to turbulence of a free convection boundary layer. In Proceedings of the General Discussion on Heat Transfer, pp. 321–323. Institute of Mechanical Engineers.Google Scholar
Eckert, E. R. G., Soehngen, E. & Schneider, F. J. 1955 Studien zum Umschlag laminar-turbulent der freien Konvektions-Strömung an einer senkrechten Platte. In Anniversary volume Fünfzig Jahre Grenzschichtforschung, pp. 407–418. Vieweg+Teubner Verlag.10.1007/978-3-663-20219-6_38Google Scholar
Gebhart, B. & Mahajan, R. L. 1982 Instability and transition in buoyancy-induced flows. In Adv. Appl. Mech., vol. 22, pp. 231–315. Elsevier.Google Scholar
Gill, A. E. & Davey, A. 1969 Instabilities of a buoyancy-driven system. J. Fluid Mech. 35 (4), 775–798.10.1017/S0022112069001431Google Scholar
Goldstein, R. J. & Briggs, D. G. 1964 Transient free convection about vertical plates and circular cylinders. Trans. ASME J. Heat Transfer 86 (4), 490–500.10.1115/1.3688728Google Scholar
Hieber, C. A. & Gebhart, B. 1971 Stability of vertical natural convection boundary layers: some numerical solutions. J. Fluid Mech. 48 (4), 625–646.10.1017/S0022112071001770Google Scholar
Illingworth, C. R. 1950 Unsteady laminar flow of gas near an infinite flat plate. In Math. Proc. Camb. Philos. Soc., vol. 46, pp. 603–613. Cambridge University Press.Google Scholar
Janssen, R. & Armfield, S. W. 1996 Stability properties of the vertical boundary layers in differentially heated cavities. Intl J. Heat Fluid Flow 17 (6), 547–556.10.1016/S0142-727X(96)00077-XGoogle Scholar
Joshi, Y. & Gebhart, B. 1987 Transition of transient vertical natural-convection flows in water. J. Fluid Mech. 179, 407–438.10.1017/S0022112087001599Google Scholar
Knowles, C. P. & Gebhart, B. 1968 The stability of the laminar natural convection boundary layer. J. Fluid Mech. 34 (4), 657–686.10.1017/S0022112068002156Google Scholar
Krane, M. J. M. & Gebhart, B. 1993 The hydrodynamic stability of a one-dimensional transient buoyancy-induced flow. Intl J. Heat Mass Transfer 36 (4), 977–988.10.1016/S0017-9310(05)80282-0Google Scholar
Kurtz, E. F.1961 A study of the stability of laminar parallel flows. PhD thesis, Massachusetts Institute of Technology, Cambridge, MA.Google Scholar
Kurtz, E. F. & Crandall, S. H. 1962 Computer-aided analysis of hydrodynamic stability. J. Math. Phys. 41 (1–4), 264–279.10.1002/sapm1962411264Google Scholar
Mahajan, R. L. & Gebhart, B. 1979 An experimental determination of transition limits in a vertical natural convection flow adjacent to a surface. J. Fluid Mech. 91 (1), 131–154.10.1017/S0022112079000070Google Scholar
McBain, G. D., Armfield, S. W. & Desrayaud, G. 2007 Instability of the buoyancy layer on an evenly heated vertical wall. J. Fluid Mech. 587, 453–469.10.1017/S0022112007007318Google Scholar
Morkovin, M. V. 1969 On the many faces of transition. In Viscous Drag Reduction, pp. 1–31. Springer.Google Scholar
Nachtsheim, P. R.1963 Stability of free-convection boundary-layer flows. NASA Tech. Note D-2089. NASA.Google Scholar
Nakao, K., Hattori, Y. & Suto, H. 2017 Numerical investigation of a spatially developing turbulent natural convection boundary layer along a vertical heated plate. Intl J. Heat Fluid Flow 63, 128–138.10.1016/j.ijheatfluidflow.2016.09.006Google Scholar
Ostrach, S.1952 An analysis of laminar free-convection flow and heat transfer about a flat plate parallel to the direction of the generating body force. NACA Tech. Rep. 1111.Google Scholar
Otto, S. R.1993 On the stability of a time dependent boundary layer. NASA Tech. Note CR-191542.Google Scholar
Patterson, J. C. & Imberger, J. 1980 Unsteady natural convection in a rectangular cavity. J. Fluid Mech. 100 (1), 65–86.10.1017/S0022112080001012Google Scholar
Plapp, J. E.1957 I. laminar boundary layer stability in free convection. PhD thesis, California Institute of Technology, Pasadena, CA.Google Scholar
Polymeropoulos, C. E. & Gebhart, B. 1967 Incipient instability in free convection laminar boundary layers. J. Fluid Mech. 30 (2), 225–239.10.1017/S0022112067001405Google Scholar
Qureshi, Z. H. & Gebhart, B. 1978 Transition and transport in a buoyancy driven flow in water adjacent to a vertical uniform flux surface. Intl J. Heat Mass Transfer 21 (12), 1467–1479.10.1016/0017-9310(78)90003-0Google Scholar
Reed, H. L., Saric, W. S. & Arnal, D. 1996 Linear stability theory applied to boundary layers. Annu. Rev. Fluid Mech. 28 (1), 389–428.10.1146/annurev.fl.28.010196.002133Google Scholar
Saric, W. S., Reed, H. L. & Kerschen, E. J. 2002 Boundary-layer receptivity to freestream disturbances. Annu. Rev. Fluid Mech. 34 (1), 291–319.10.1146/annurev.fluid.34.082701.161921Google Scholar
Schetz, J. A. & Eichhorn, R. 1962 Unsteady natural convection in the vicinity of a doubly infinite vertical plate. J. Heat Transfer 84 (4), 334–338.10.1115/1.3684386Google Scholar
Shen, S. F. 1961 Some considerations on the laminar stability of time-dependent basic flows. J. Aero. Sci. 28 (5), 397–404.Google Scholar
Squire, H. B. 1933 On the stability for three-dimensional disturbances of viscous fluid flow between parallel walls. Proc. R. Soc. Lond. A 142 (847), 621–628.10.1098/rspa.1933.0193Google Scholar
Szewczyk, A. A. 1962 Stability and transition of the free-convection layer along a vertical flat plate. Intl J. Heat Mass Transfer 5 (10), 903–914.10.1016/0017-9310(62)90071-6Google Scholar
Williamson, N., Armfield, S. W. & Kirkpatrick, M. P. 2012 Transition to oscillatory flow in a differentially heated cavity with a conducting partition. J. Fluid Mech. 693, 93–114.10.1017/jfm.2011.471Google Scholar
Williamson, N., Armfield, S. W., Lin, W. & Kirkpatrick, M. P. 2016 Stability and Nusselt number scaling for inclined differentially heated cavity flow. Intl J. Heat Mass Transfer 97, 787–793.10.1016/j.ijheatmasstransfer.2016.02.053Google Scholar
Wu, X. & Cowley, S. J. 1995 On the nonlinear evolution of instability modes in unsteady shear layers: the Stokes layer as a paradigm. Q. J. Mech. Appl. Math. 48 (2), 159–188.10.1093/qjmam/48.2.159Google Scholar
Xin, S. & Le Quéré, P. 2001 Linear stability analyses of natural convection flows in a differentially heated square cavity with conducting horizontal walls. Phys. Fluids 13 (9), 2529–2542.10.1063/1.1388054Google Scholar
Xin, S. & Le Quéré, P. 2012 Stability of two-dimensional (2D) natural convection flows in air-filled differentially heated cavities: 2D/3D disturbances. Fluid Dyn. Res. 44 (3), 031419.Google Scholar
Zhao, Y., Lei, C. & Patterson, J. C. 2017 The k-type and h-type transitions of natural convection boundary layers. J. Fluid Mech. 824, 352–387.10.1017/jfm.2017.354Google Scholar