Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Chen, Chang Hsin
Donzis, Diego A.
and
Bowersox, Rodney D.
2020.
Characteristic Locations in Shock-Turbulence Interactions.
Sethuraman, Yogesh Prasaad Madras
and
Sinha, Krishnendu
2020.
Effect of turbulent Mach number on the thermodynamic fluctuations in canonical shock-turbulence interaction.
Computers & Fluids,
Vol. 197,
Issue. ,
p.
104354.
Kumari, Komal
and
Donzis, Diego A.
2020.
Direct numerical simulations of turbulent flows using high-order asynchrony-tolerant schemes: Accuracy and performance.
Journal of Computational Physics,
Vol. 419,
Issue. ,
p.
109626.
Aruga, Kenta
Inokuma, Kento
Watanabe, Tomoaki
Nagata, Koji
and
Sakai, Yasuhiko
2020.
Experimental investigation of interactions between turbulent cylinder wake and spherical shock wave.
Physics of Fluids,
Vol. 32,
Issue. 1,
Tanaka, K.
Watanabe, T.
and
Nagata, K.
2020.
Statistical analysis of deformation of a shock wave propagating in a local turbulent region.
Physics of Fluids,
Vol. 32,
Issue. 9,
Gao, Xiangyu
Bermejo-Moreno, Ivan
and
Larsson, Johan
2020.
Parametric numerical study of passive scalar mixing in shock turbulence interaction.
Journal of Fluid Mechanics,
Vol. 895,
Issue. ,
Fukushima, Gaku
Ogawa, Shingo
Wei, Jiaxi
Hagiwara, Jun
Nakamura, Yusuke
and
Sasoh, Akihiro
2021.
Moderation of Shock Wave Profile in Grid Turbulence.
Fukushima, Gaku
Wei, Jiaxi
Ogawa, Shingo
Hagiwara, Jun
Nakamura, Yusuke
and
Sasoh, Akihiro
2021.
Losing the shock wave front profile due to interaction with turbulence.
Fluid Dynamics Research,
Vol. 53,
Issue. 2,
p.
025504.
Grube, Nathan E.
and
Martín, M. Pino
2021.
Reynolds stress anisotropy in shock/isotropic turbulence interactions.
Journal of Fluid Mechanics,
Vol. 913,
Issue. ,
Lacombe, Francis
Roy, Subhajit
Sinha, Krishnendu
Karl, Sebastian
and
Hickey, Jean-Pierre
2021.
Characteristic Scales in Shock–Turbulence Interaction.
AIAA Journal,
Vol. 59,
Issue. 2,
p.
526.
Hamzehloo, Arash
Lusher, David J.
Laizet, Sylvain
and
Sandham, Neil D.
2021.
On the performance of WENO/TENO schemes to resolve turbulence in DNS/LES of high‐speed compressible flows.
International Journal for Numerical Methods in Fluids,
Vol. 93,
Issue. 1,
p.
176.
Fukushima, G.
Ogawa, S.
Wei, J.
Nakamura, Y.
and
Sasoh, A.
2021.
Impacts of grid turbulence on the side projection of planar shock waves.
Shock Waves,
Vol. 31,
Issue. 2,
p.
101.
McManamen, B.
Donzis, D.A.
North, S.W.
and
Bowersox, R.D.W.
2021.
Velocity and temperature fluctuations in a high-speed shock–turbulence interaction.
Journal of Fluid Mechanics,
Vol. 913,
Issue. ,
Inokuma, Kento
Watanabe, Tomoaki
Nagata, Koji
and
Sakai, Yasuhiko
2021.
Experimental study of shock wave modulation caused by velocity and temperature fluctuations in cylinder wakes.
Physical Review Fluids,
Vol. 6,
Issue. 6,
Huete, C.
Cuadra, A.
Vera, M.
and
Urzay, J.
2021.
Thermochemical effects on hypersonic shock waves interacting with weak turbulence.
Physics of Fluids,
Vol. 33,
Issue. 8,
Davidovits, Seth
Federrath, Christoph
Teyssier, Romain
Raman, Kumar S.
Collins, David C.
and
Nagel, Sabrina R.
2022.
Turbulence generation by shock interaction with a highly nonuniform medium.
Physical Review E,
Vol. 105,
Issue. 6,
Huang, Zhiwei
Cleary, Matthew J.
Ren, Zhuyin
and
Zhang, Huangwei
2022.
Large eddy simulation of a supersonic lifted hydrogen flame with sparse-Lagrangian multiple mapping conditioning approach.
Combustion and Flame,
Vol. 238,
Issue. ,
p.
111756.
Chen, Chang Hsin
and
Donzis, Diego A.
2022.
Amplification of Transverse Reynolds Stresses in Shock–Turbulence Interactions.
AIAA Journal,
Vol. 60,
Issue. 11,
p.
6235.
Thakare, Pranav
Nair, Vineeth
and
Sinha, Krishnendu
2022.
A weakly nonlinear framework to study shock–vorticity interaction.
Journal of Fluid Mechanics,
Vol. 933,
Issue. ,
Thakare, Pranav B.
Sinha, Krishnendu
and
Nair, Vineeth
2022.
A weakly nonlinear analysis for pressure generation in shock vorticity interaction.
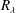 $R_{\unicode[STIX]{x1D706}}$,
$R_{\unicode[STIX]{x1D706}}$,  $M$ and
$M$ and 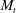 $M_{t}$, respectively). The simulations are shock and turbulence resolving with
$M_{t}$, respectively). The simulations are shock and turbulence resolving with 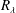 $R_{\unicode[STIX]{x1D706}}$ up to 65,
$R_{\unicode[STIX]{x1D706}}$ up to 65, 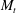 $M_{t}$ up to 0.54 and
$M_{t}$ up to 0.54 and 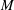 $M$ up to 1.4. The focus is on the effect of strong turbulence on the jumps of mean thermodynamic variables across the shock, the shock structure and the amplification of turbulence as it moves through the shock. Theoretical results under the so-called quasi-equilibrium (QE) assumption provide explicit laws for a number of statistics of interests which are in agreement with the new DNS data presented here as well as all the data available in the literature. While in previous studies turbulence was found to weaken jumps, it is shown here that stronger jumps are also observed depending on the regime of the interaction. Statistics of the dilatation at the shock are also investigated and found to be well represented by QE for weak turbulence but saturate at high turbulence intensities with a Reynolds number dependence also captured by the analysis. Finally, amplification factors are found to present a universal behaviour with two limiting asymptotic regimes governed by
$M$ up to 1.4. The focus is on the effect of strong turbulence on the jumps of mean thermodynamic variables across the shock, the shock structure and the amplification of turbulence as it moves through the shock. Theoretical results under the so-called quasi-equilibrium (QE) assumption provide explicit laws for a number of statistics of interests which are in agreement with the new DNS data presented here as well as all the data available in the literature. While in previous studies turbulence was found to weaken jumps, it is shown here that stronger jumps are also observed depending on the regime of the interaction. Statistics of the dilatation at the shock are also investigated and found to be well represented by QE for weak turbulence but saturate at high turbulence intensities with a Reynolds number dependence also captured by the analysis. Finally, amplification factors are found to present a universal behaviour with two limiting asymptotic regimes governed by  $(M-1)$ and
$(M-1)$ and 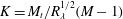 $K=M_{t}/R_{\unicode[STIX]{x1D706}}^{1/2}(M-1)$, for weak and strong turbulence, respectively. Effect of anisotropy in the incoming flow is also assessed by utilizing two different forcing mechanisms to generate turbulence.
$K=M_{t}/R_{\unicode[STIX]{x1D706}}^{1/2}(M-1)$, for weak and strong turbulence, respectively. Effect of anisotropy in the incoming flow is also assessed by utilizing two different forcing mechanisms to generate turbulence.