Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-05T02:31:17.371Z Has data issue: false hasContentIssue false
  • English
  • Français

Puffing in planar buoyant plumes: BiGlobal instability analysis and experiments

Published online by Cambridge University Press:  28 January 2019

Kuchimanchi K. Bharadwaj  and
Debopam Das Open the ORCID record for Debopam Das [Opens in a new window]
Kuchimanchi K. Bharadwaj
Affiliation:
Department of Aerospace Engineering, Indian Institute of Technology Kanpur, Kanpur, UP 208016, India
Debopam Das*
Affiliation:
Department of Aerospace Engineering, Indian Institute of Technology Kanpur, Kanpur, UP 208016, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The present study investigates the puffing behaviour of planar buoyant plumes by employing linear BiGlobal stability analysis and experiments. The BiGlobal instability characteristics of two-dimensional plumes have been explored using stability analysis and compared with the puffing behaviour of both rectangular plumes and square plumes obtained from experiments. In the parameter space investigated, which spans a Richardson number range 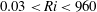$0.03<Ri<960$, instability analysis reveals that planar plumes exhibit BiGlobal instability only for varicose perturbations, while they remain stable for sinuous perturbations. The BiGlobal frequency and growth rates of the unstable varicose mode are used to obtain Strouhal number correlation and stability curves. An investigation into the effect of the spanwise wavenumber on BiGlobal instability indicates that planar plumes are more unstable to two-dimensional perturbations than to three-dimensional perturbations. An increase in the spanwise wavenumber tends to stabilize planar plumes without affecting their oscillation frequencies. Experiments suggest that the puffing frequencies in rectangular plumes closely follow the power law obtained from two-dimensional instability analysis while exhibiting a weaker dependence on inlet aspect ratio. To further explore the effect of aspect ratio on puffing behaviour, experiments have been carried out in plumes of aspect ratio 1, i.e. square plumes. Square plumes are found to be more stable and to exhibit higher puffing frequencies than rectangular plumes. The reasons for these differences in puffing dynamics between rectangular and square plumes have been explored from the phase-locked streamwise and spanwise flow visualizations. In addition to puffing, spanwise visualizations in both rectangular and square plumes show the presence of secondary flows at their corners, similar to their constant-density jet counterparts. Finally, from experiments, we deduced a new universal puffing frequency correlation with the hydraulic diameter as the length scale which eliminates the aspect ratio dependence, and is valid for both square and low-aspect-ratio rectangular plumes.

JFM classification

Convection: Buoyancy-driven instability Boundary Layers: Free shear layers Convection: Plumes/thermals
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 863 , 25 March 2019 , pp. 817 - 849
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

ANSYS, Inc.2009 ANSYS Fluent 12.0 user’s guide.Google Scholar
ANSYS, Inc.2013 Release 15.0 theory guide.Google Scholar
Bharadwaj, K. K. & Das, D. 2017 Global instability analysis and experiments on buoyant plumes. J. Fluid Mech. 832, 97–145.10.1017/jfm.2017.665Google Scholar
Cetegen, B. M. 1997 Behavior of naturally unstable and periodically forced axisymmetric buoyant plumes of helium and helium–air mixtures. Phys. Fluids 9 (12), 3742–3752.10.1063/1.869512Google Scholar
Cetegen, B. M. & Ahmed, T. A. 1993 Experiments on the periodic instability of buoyant plumes and pool fires. Combust. Flame 93 (1–2), 157–184.10.1016/0010-2180(93)90090-PGoogle Scholar
Cetegen, B. M., Dong, Y. & Soteriou, M. C. 1998 Experiments on stability and oscillatory behavior of planar buoyant plumes. Phys. Fluids 10 (7), 1658–1665.10.1063/1.869683Google Scholar
Cetegen, B. M. & Kasper, K. D. 1996 Experiments on the oscillatory behavior of buoyant plumes of helium and helium–air mixtures. Phys. Fluids 8 (11), 2974–2984.10.1063/1.869075Google Scholar
Chakravarthy, R. V. K., Lesshafft, L. & Huerre, P. 2018 Global stability of buoyant jets and plumes. J. Fluid Mech. 835, 654–673.10.1017/jfm.2017.764Google Scholar
Chandler, G. J., Juniper, M. P., Nichols, J. W. & Schmid, P. J. 2012 Adjoint algorithms for the Navier–Stokes equations in the low Mach number limit. J. Comput. Phys. 231 (4), 1900–1916.10.1016/j.jcp.2011.11.013Google Scholar
Chomaz, J.-M. 2003 Fully nonlinear dynamics of parallel wakes. J. Fluid Mech. 495, 57–75.10.1017/S0022112003006335Google Scholar
Coenen, W., Lesshafft, L., Garnaud, X. & Sevilla, A. 2017 Global instability of low-density jets. J. Fluid Mech. 820, 187–207.10.1017/jfm.2017.203Google Scholar
Garnaud, X., Lesshafft, L., Schmid, P. J. & Huerre, P. 2013 Modal and transient dynamics of jet flows. Phys. Fluids 25 (4), 044103.10.1063/1.4801751Google Scholar
Gebhart, B., Jaluria, Y., Mahajan, R. L. & Sammakia, B. 1988 Buoyancy-Induced Flows and Transport. Springer.Google Scholar
Gutmark, E. J. & Grinstein, F. F. 1999 Flow control with noncircular jets. Annu. Rev. Fluid Mech. 31 (1), 239–272.10.1146/annurev.fluid.31.1.239Google Scholar
Hattori, T., Bartos, N., Norris, S. E., Kirkpatrick, M. P. & Armfield, S. W. 2013a Experimental and numerical investigation of unsteady behaviour in the near-field of pure thermal planar plumes. Exp. Therm. Fluid Sci. 46, 139–150.10.1016/j.expthermflusci.2012.12.005Google Scholar
Hattori, T., Norris, S. E., Kirkpatrick, M. P. & Armfield, S. W. 2013b Prandtl number dependence and instability mechanism of the near-field flow in a planar thermal plume. J. Fluid Mech. 732, 105–127.10.1017/jfm.2013.392Google Scholar
Hattori, T., Norris, S. E., Kirkpatrick, M. P. & Armfield, S. W. 2013c Simulation and analysis of puffing instability in the near field of pure thermal planar plumes. Intl J. Therm. Sci. 69, 1–13.10.1016/j.ijthermalsci.2013.01.016Google Scholar
Huerre, P. & Monkewitz, P. A. 1990 Local and global instabilities in spatially developing flows. Annu. Rev. Fluid Mech. 22 (1), 473–537.10.1146/annurev.fl.22.010190.002353Google Scholar
Jiang, X. & Luo, K. H. 2000a Direct numerical simulation of the puffing phenomenon of an axisymmetric thermal plume. Theor. Comput. Fluid Dyn. 14 (1), 55–74.10.1007/s001620050125Google Scholar
Jiang, X. & Luo, K. H. 2000b Spatial direct numerical simulation of the large vortical structures in forced plumes. Flow Turbul. Combust. 64 (1), 43–69.10.1023/A:1009950127478Google Scholar
Juniper, M. P., Hanifi, A. & Theofilis, V. 2014 Modal stability theory: lecture notes from the FLOW-NORDITA summer school on advanced instability methods for complex flows, Stockholm, Sweden, 2013. Appl. Mech. Rev. 66 (2), 024804.10.1115/1.4026604Google Scholar
Lesshafft, L. 2018 Artificial eigenmodes in truncated flow domains. Theor. Comput. Fluid Dyn. 32, 245–262.10.1007/s00162-017-0449-6Google Scholar
Lesshafft, L. & Huerre, P. 2007 Linear impulse response in hot round jets. Phys. Fluids 19 (2), 024102.10.1063/1.2437238Google Scholar
Malalasekera, W. M. G., Versteeg, H. K. & Gilchrist, K. 1996 A review of research and an experimental study on the pulsation of buoyant diffusion flames and pool fires. Fire Mater. 20 (6), 261–271.10.1002/(SICI)1099-1018(199611)20:6<261::AID-FAM578>3.0.CO;2-M3.0.CO;2-M>Google Scholar
Nichols, J. W., Schmid, P. J. & Riley, J. J. 2007 Self-sustained oscillations in variable-density round jets. J. Fluid Mech. 582, 341–376.10.1017/S0022112007005903Google Scholar
Pera, L. & Gebhart, B. 1971 On the stability of laminar plumes: some numerical solutions and experiments. Intl J. Heat Mass Transfer 14 (7), 975–984.10.1016/0017-9310(71)90123-2Google Scholar
Quinn, W. R. 1992 Streamwise evolution of a square jet cross section. AIAA J. 30 (12), 2852–2857.10.2514/3.48973Google Scholar
Ravier, S., Abid, M., Amielh, M. & Anselmet, F. 2006 Direct numerical simulations of variable-density plane jets. J. Fluid Mech. 546, 153–191.10.1017/S0022112005006993Google Scholar
Raynal, L., Harion, J.-L., Favre-Marinet, M. & Binder, G. 1996 The oscillatory instability of plane variable-density jets. Phys. Fluids 8 (4), 993–1006.10.1063/1.868877Google Scholar
Sau, A. 1999 Three-dimensional simulation of flows through a rectangular sudden expansion. Phys. Fluids 11 (10), 3003–3016.10.1063/1.870159Google Scholar
Sau, A. 2002 Vortex dynamics and mass entrainment in a rectangular channel with a suddenly expanded and contracted part. Phys. Fluids 14 (9), 3280–3308.10.1063/1.1498839Google Scholar
Schmid, P. J. & Henningson, D. S. 2012 Stability and Transition in Shear Flows, vol. 142. Springer Science & Business Media.Google Scholar
Soteriou, M. C., Dong, Y. & Cetegen, B. M. 2002 Lagrangian simulation of the unsteady near field dynamics of planar buoyant plumes. Phys. Fluids 14 (9), 3118–3140.10.1063/1.1491248Google Scholar
Sreenivasan, K. R., Raghu, S. & Kyle, D. 1989 Absolute instability in variable density round jets. Exp. Fluids 7 (5), 309–317.10.1007/BF00198449Google Scholar
Subbarao, E. R. & Cantwell, B. J. 1992 Investigation of a co-flowing buoyant jet: experiments on the effect of Reynolds number and Richardson number. J. Fluid Mech. 245, 69–90.10.1017/S0022112092000351Google Scholar
Theofilis, V. 2003 Advances in global linear instability analysis of nonparallel and three-dimensional flows. Prog. Aerosp. Sci. 39 (4), 249–315.10.1016/S0376-0421(02)00030-1Google Scholar
Tieszen, S. R. 2001 On the fluid mechanics of fires. Annu. Rev. Fluid Mech. 33 (1), 67–92.10.1146/annurev.fluid.33.1.67Google Scholar
Versteeg, H. K. & Malalasekera, W. 2007 An Introduction to Computational Fluid Dynamics: The Finite Volume Method. Pearson Education.Google Scholar
Wilke, C. R. 1950 A viscosity equation for gas mixtures. J. Chem. Phys. 18 (4), 517–519.10.1063/1.1747673Google Scholar
Yang, H. Q. 1992 Buckling of a thermal plume. Intl J. Heat Mass Transfer 35 (6), 1527–1532.10.1016/0017-9310(92)90042-QGoogle Scholar
Yu, M.-H. & Monkewitz, P. A. 1993 Oscillations in the near field of a heated two-dimensional jet. J. Fluid Mech. 255, 323–347.10.1017/S0022112093002502Google Scholar
Zaman, K. B. M. Q. 1996 Axis switching and spreading of an asymmetric jet: the role of coherent structure dynamics. J. Fluid Mech. 316, 1–27.10.1017/S0022112096000420Google Scholar