Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-17T09:17:22.987Z Has data issue: false hasContentIssue false
  • English
  • Français

On the large difference between Benjamin’s and Hanratty’s formulations of perturbed flow over uneven terrain

Published online by Cambridge University Press:  24 May 2019

Paolo Luchini Open the ORCID record for Paolo Luchini [Opens in a new window]  and
François Charru
Paolo Luchini*
Affiliation:
Department of Industrial Engineering, University of Salerno, 84084 Fisciano, Italy
François Charru
Affiliation:
Institut de Mècanique des Fluides de Toulouse, 31400 Toulouse, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Flow over an uneven terrain is a complex phenomenon that requires a chain of approximations in order to be studied. In addition to modelling the intricacies of turbulence if present, the problem is classically first linearized about a flat bottom and a locally parallel flow, and then asymptotically approximated into an interactive representation that couples a boundary layer and an irrotational region through an intermediate inviscid but rotational layer. The first of these steps produces a stationary Orr–Sommerfeld equation; since this is a one-dimensional problem comparatively easy for any computer, it would seem appropriate today to forgo the second sweep of approximation and solve the Orr–Sommerfeld problem numerically. However, the results are inconsistent! It appears that the asymptotic approximation tacitly restores some of the original problem’s non-parallelism. In order to provide consistent results, Benjamin’s version of the Orr–Sommerfeld equation needs to be modified into Hanratty’s. The large difference between Benjamin’s and Hanratty’s formulations, which arises in some wavenumber ranges but not in others, is here explained through an asymptotic analysis based on the concept of admittance and on the symmetry transformations of the boundary layer. A compact and accurate analytical formula is provided for the wavenumber range of maximum laminar shear-stress response. We highlight that the maximum turbulent shear-stress response occurs in the quasi-laminar regime at a Reynolds-independent wavenumber, contrary to the maximum laminar shear-stress response whose wavenumber scales with a power of the boundary-layer thickness. A numerical computation involving an eddy-viscosity model provides a warning against the inaccuracy of such a model. We emphasize that the range 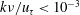$k\unicode[STIX]{x1D708}/u_{\unicode[STIX]{x1D70F}}<10^{-3}$ of the spectrum remains essentially unexplored, and that the question is still open whether a fully developed turbulent regime, similar to the one predicted by an eddy-viscosity model, ever exists for open flow even in the limit of infinite wavelength.

JFM classification

Boundary Layers: Boundary layer structure Geophysical and Geological Flows: Topographic effects
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 871 , 25 July 2019 , pp. 534 - 561
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abramowitz, M. & Stegun, I. A. 1972 Handbook of Mathematical Functions, 10th edn. Applied Mathematics Series, vol. 55. National Bureau of Standards.Google Scholar
Abrams, J. & Hanratty, T. J. 1985 Relaxation effects observed for turbulent flow over a wavy surface. J. Fluid Mech. 151, 443–455.Google Scholar
Belcher, S. E. & Hunt, J. C. R. 1993 Turbulent shear flow over slowly moving waves. J. Fluid Mech. 251, 109–148.Google Scholar
Belcher, S. E. & Hunt, J. C. R. 1998 Turbulent flow over hills and waves. Annu. Rev. Fluid Mech. 30, 507–538.Google Scholar
Benjamin, T. B. 1957 Wave formation in laminar flow down an inclined plane. J. Fluid Mech. 2, 554–574.Google Scholar
Benjamin, T. B. 1959 Shearing flow over a wavy boundary. J. Fluid Mech. 6, 161–205.Google Scholar
Charru, F., Andreotti, B. & Claudin, P. 2013 Sand ripples and dunes. Annu. Rev. Fluid Mech. 45, 469–493.Google Scholar
Charru, F. & Hinch, E. J. 2000 Phase diagram of interfacial instabilities in a two-layer Couette flow and mechanism of the long-wave instability. J. Fluid Mech. 414, 195–233.Google Scholar
Ern, P., Charru, F. & Luchini, P. 2003 Stability analysis of a shear flow with strongly stratified viscosity. J. Fluid Mech. 496, 295–312.Google Scholar
Jackson, P. S. & Hunt, J. C. R. 1975 Turbulent wind flow over a low hill. Q. J. R. Meteorol. Soc. 101, 929–955.Google Scholar
Luchini, P. 2016 Immersed-boundary simulations of turbulent flow past a sinusoidally undulated river bottom. Eur. J. Mech. B/Fluids 55, 340–347.Google Scholar
Luchini, P. 2017 Addendum to ‘Immersed-boundary simulations of turbulent flow past a sinusoidally undulated river bottom’. Eur. J. Mech. B/Fluids 62, 57–58.Google Scholar
Luchini, P. 2018a Structure and interpolation of the turbulent velocity profile in parallel flow. Eur. J. Mech. B/Fluids 71, 15–34.Google Scholar
Luchini, P. 2018b An elementary example of contrasting laminar and turbulent flow physics. Phys. Rev. Fluids (submitted), arXiv:1811.11877.Google Scholar
Luchini, P. & Charru, F. 2010 The phase lead of shear stress in shallow-water flow over a perturbed bottom. J. Fluid Mech. 665, 516–539.Google Scholar
Luchini, P. & Charru, F. 2017 Quasilaminar regime in the linear response of a turbulent flow to wall waviness. Phys. Rev. Fluids 2 (1), 012601.Google Scholar
Russo, S. & Luchini, P. 2016 The linear response of turbulent flow to a volume force: comparison between eddy-viscosity model and DNS. J. Fluid Mech. 790, 104–127.Google Scholar
Thorsness, C. B.1975 Transport phenomena associated with flow over a solid wavy surface. PhD thesis in Chemical Engineering, University of Illinois, Urbana.Google Scholar
Thorsness, C. B., Morrisroe, P. E. & Hanratty, T. J. 1978 A comparison of linear theory with measurements of the variation of shear stress along a solid wave. Chem. Engng Sci. 33, 579–592.Google Scholar
Tollmien, W.1929 Über die Entstehung der Turbulenz. Nachr. Ges. Wiss. Göttingen, Math.-phys. Kl. 21–44. Translated into English as ‘The production of turbulence’, Tech. Memo. Nat. Adv. Comm. Aero., Wash. No. 609 (1931).Google Scholar
Zilker, D. P., Cook, G. W. & Hanratty, T. J. 1977 Influence of the amplitude of a solid wavy wall on a turbulent flow. Part 1. Non-separated flows. J. Fluid Mech. 82, 29–51.Google Scholar