Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Lagrée, Pierre-Yves
Saingier, Guillaume
Deboeuf, Stéphanie
Staron, Lydie
Popinet, Stéphane
Radjai, F.
Nezamabadi, S.
Luding, S.
and
Delenne, J.Y.
2017.
Granular front for flow down a rough incline: about the value of the shape factor in depths averaged models.
EPJ Web of Conferences,
Vol. 140,
Issue. ,
p.
03046.
Saramito, Pierre
and
Wachs, Anthony
2017.
Progress in numerical simulation of yield stress fluid flows.
Rheologica Acta,
Vol. 56,
Issue. 3,
p.
211.
Lusso, Christelle
Bouchut, François
Ern, Alexandre
and
Mangeney, Anne
2017.
A Free Interface Model for Static/Flowing Dynamics in Thin-Layer Flows of Granular Materials with Yield: Simple Shear Simulations and Comparison with Experiments.
Applied Sciences,
Vol. 7,
Issue. 4,
p.
386.
Delannay, R
Valance, A
Mangeney, A
Roche, O
and
Richard, P
2017.
Granular and particle-laden flows: from laboratory experiments to field observations.
Journal of Physics D: Applied Physics,
Vol. 50,
Issue. 5,
p.
053001.
Bouchut, F.
Fernández-Nieto, E.D.
Koné, E.H.
Mangeney, A.
Narbona-Reina, G.
Radjai, F.
Nezamabadi, S.
Luding, S.
and
Delenne, J.Y.
2017.
A two-phase solid-fluid model for dense granular flows including dilatancy effects: comparison with submarine granular collapse experiments.
EPJ Web of Conferences,
Vol. 140,
Issue. ,
p.
09039.
Yamada, Masumi
Mangeney, Anne
Matsushi, Yuki
and
Matsuzawa, Takanori
2018.
Estimation of dynamic friction and movement history of large landslides.
Landslides,
Vol. 15,
Issue. 10,
p.
1963.
Fernández-Nieto, E.D.
Garres-Díaz, J.
Mangeney, A.
and
Narbona-Reina, G.
2018.
2D granular flows with the μ(I) rheology and side walls friction: A well-balanced multilayer discretization.
Journal of Computational Physics,
Vol. 356,
Issue. ,
p.
192.
Bonaventura, Luca
Fernández-Nieto, Enrique D.
Garres-Díaz, José
and
Narbona-Reina, Gladys
2018.
Multilayer shallow water models with locally variable number of layers and semi-implicit time discretization.
Journal of Computational Physics,
Vol. 364,
Issue. ,
p.
209.
Chacón Rebollo, Tomás
Franco Coronil, Daniel
and
Hecht, Frédéric
2019.
A Petrov–Galerkin multilayer discretization to second order elliptic boundary value problems.
Computers & Mathematics with Applications,
Vol. 77,
Issue. 12,
p.
3068.
Allgeyer, Sebastien
Bristeau, Marie-Odile
Froger, David
Hamouda, Raouf
Jauzein, V.
Mangeney, Anne
Sainte-Marie, Jacques
Souillé, Fabien
and
Vallée, Martin
2019.
Numerical approximation of the 3D hydrostatic Navier–Stokes system with free surface.
ESAIM: Mathematical Modelling and Numerical Analysis,
Vol. 53,
Issue. 6,
p.
1981.
Yu, Ming-Lan
and
Lee, Cheng-Hsien
2019.
Multi-phase-flow modeling of underwater landslides on an inclined plane and consequently generated waves.
Advances in Water Resources,
Vol. 133,
Issue. ,
p.
103421.
Garres-Díaz, J.
Bouchut, F.
Fernández-Nieto, E.D.
Mangeney, A.
and
Narbona-Reina, G.
2020.
Multilayer models for shallow two-phase debris flows with dilatancy effects.
Journal of Computational Physics,
Vol. 419,
Issue. ,
p.
109699.
De Vita, Francesco
Lagrée, Pierre-Yves
Chibbaro, Sergio
and
Popinet, Stéphane
2020.
Beyond Shallow Water: Appraisal of a numerical approach to hydraulic jumps based upon the Boundary Layer theory.
European Journal of Mechanics - B/Fluids,
Vol. 79,
Issue. ,
p.
233.
Duan, Zhao
Wu, Yan-Bin
Tang, Hao
Ma, Jian-Quan
Zhu, Xing-Hua
and
Shi, Haiyun
2020.
An Analysis of Factors Affecting Flowslide Deposit Morphology Using Taguchi Method.
Advances in Civil Engineering,
Vol. 2020,
Issue. 1,
Lin, Cheng-Chuan
and
Yang, Fu-Ling
2020.
Continuum simulation for regularized non-local μ(I) model of dense granular flows.
Journal of Computational Physics,
Vol. 420,
Issue. ,
p.
109708.
Bristeau, Marie-Odile
Di Martino, Bernard
Mangeney, Anne
Sainte-Marie, Jacques
and
Souillé, Fabien
2021.
Some analytical solutions for validation of free surface flow computational codes.
Journal of Fluid Mechanics,
Vol. 913,
Issue. ,
Sarno, L.
Wang, Y.
Tai, Y.-C.
Martino, R.
and
Carravetta, A.
2021.
Asymptotic analysis of the eigenstructure of the two-layer model and a new family of criteria for evaluating the model hyperbolicity.
Advances in Water Resources,
Vol. 154,
Issue. ,
p.
103966.
Guimpier, A.
Conway, S.J.
Mangeney, A.
Lucas, A.
Mangold, N.
Peruzzetto, M.
Pajola, M.
Lucchetti, A.
Munaretto, G.
Sæmundsson, T.
Johnsson, A.
Le Deit, L.
Grindrod, P.
Davis, J.
Thomas, N.
and
Cremonese, G.
2021.
Dynamics of recent landslides (<20 My) on Mars: Insights from high-resolution topography on Earth and Mars and numerical modelling.
Planetary and Space Science,
Vol. 206,
Issue. ,
p.
105303.
Choi, C.E.
and
Goodwin, G.R.
2021.
Effects of interactions between transient granular flows and macroscopically rough beds and their implications for bulk flow dynamics.
Canadian Geotechnical Journal,
Vol. 58,
Issue. 12,
p.
1943.
Bouchut, François
Fernández-Nieto, Enrique D.
Koné, El Hadji
Mangeney, Anne
and
Narbona-Reina, Gladys
2021.
Dilatancy in dry granular flows with a compressible μ(I) rheology.
Journal of Computational Physics,
Vol. 429,
Issue. ,
p.
110013.
 ${\it\mu}(I)$-rheology: application to granular collapse on erodible beds
${\it\mu}(I)$-rheology: application to granular collapse on erodible beds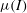 ${\it\mu}(I)$-rheology through an asymptotic analysis. The main advantages of this approximation are (i) the low cost associated with the numerical treatment of the free surface of the modelled flows, (ii) the exact conservation of mass and (iii) the ability to compute two-dimensional profiles of the velocities in the directions along and normal to the slope. The derivation of the model follows Fernández-Nieto et al. (J. Comput. Phys., vol. 60, 2014, pp. 408–437) and introduces a dimensional analysis based on the shallow flow hypothesis. The proposed first-order multilayer model fully satisfies a dissipative energy equation. A comparison with steady uniform Bagnold flow – with and without the sidewall friction effect – and laboratory experiments with a non-constant normal profile of the downslope velocity demonstrates the accuracy of the numerical model. Finally, by comparing the numerical results with experimental data on granular collapses, we show that the proposed multilayer model with the
${\it\mu}(I)$-rheology through an asymptotic analysis. The main advantages of this approximation are (i) the low cost associated with the numerical treatment of the free surface of the modelled flows, (ii) the exact conservation of mass and (iii) the ability to compute two-dimensional profiles of the velocities in the directions along and normal to the slope. The derivation of the model follows Fernández-Nieto et al. (J. Comput. Phys., vol. 60, 2014, pp. 408–437) and introduces a dimensional analysis based on the shallow flow hypothesis. The proposed first-order multilayer model fully satisfies a dissipative energy equation. A comparison with steady uniform Bagnold flow – with and without the sidewall friction effect – and laboratory experiments with a non-constant normal profile of the downslope velocity demonstrates the accuracy of the numerical model. Finally, by comparing the numerical results with experimental data on granular collapses, we show that the proposed multilayer model with the 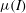 ${\it\mu}(I)$-rheology qualitatively reproduces the effect of the erodible bed on granular flow dynamics and deposits, such as the increase of runout distance with increasing thickness of the erodible bed. We show that the use of a constant friction coefficient in the multilayer model leads to the opposite behaviour. This multilayer model captures the strong change in shape of the velocity profile (from S-shaped to Bagnold-like) observed during the different phases of the highly transient flow, including the presence of static and flowing zones within the granular column.
${\it\mu}(I)$-rheology qualitatively reproduces the effect of the erodible bed on granular flow dynamics and deposits, such as the increase of runout distance with increasing thickness of the erodible bed. We show that the use of a constant friction coefficient in the multilayer model leads to the opposite behaviour. This multilayer model captures the strong change in shape of the velocity profile (from S-shaped to Bagnold-like) observed during the different phases of the highly transient flow, including the presence of static and flowing zones within the granular column.