Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-25T05:09:32.233Z Has data issue: false hasContentIssue false
  • English
  • Français

Modelling solute transport in the brain microcirculation: is it really well mixed inside the blood vessels?

Published online by Cambridge University Press:  17 December 2019

Maxime Berg ,
Yohan Davit ,
Michel Quintard Open the ORCID record for Michel Quintard [Opens in a new window]  and
Sylvie Lorthois Open the ORCID record for Sylvie Lorthois [Opens in a new window]
Maxime Berg
Affiliation:
Institut de Mécanique des Fluides de Toulouse (IMFT), Université de Toulouse, CNRS,Toulouse, France
Yohan Davit*
Affiliation:
Institut de Mécanique des Fluides de Toulouse (IMFT), Université de Toulouse, CNRS,Toulouse, France
Michel Quintard
Affiliation:
Institut de Mécanique des Fluides de Toulouse (IMFT), Université de Toulouse, CNRS,Toulouse, France
Sylvie Lorthois*
Affiliation:
Institut de Mécanique des Fluides de Toulouse (IMFT), Université de Toulouse, CNRS,Toulouse, France
*
†Email addresses for correspondence: [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Most network models describing solute transport in the brain microcirculation use the well-mixed hypothesis and assume that radial gradients inside the blood vessels are negligible. Recent experimental data suggest that these gradients, which may result from heterogeneities in the velocity field or consumption in the tissue, may in fact be important. Here, we study the validity of the well-mixed hypothesis in network models of solute transport using theoretical and computational approaches. We focus on regimes of weak coupling where the transport problem inside the vasculature is independent of the concentration field in the tissue. In these regimes, the boundary condition between vessels and tissue can be modelled by a Robin boundary condition. For this boundary condition and for a single cylindrical capillary, we derive a one-dimensional cross-section average transport problem with dispersion and exchange coefficients capturing the effects of radial gradients. We then extend this model to a network of connected tubes and solve the problem in a complex anatomical network. By comparing with results based on the well-mixed hypothesis, we find that dispersive effects are a fundamental component of transport in transient situations with relatively rapid injections, i.e. frequencies above one Hertz. For slowly varying signals and steady states, radial gradients also significantly impact the spatial distribution of vessel/tissue exchange for molecules that easily cross the blood brain barrier. This suggests that radial gradients cannot be systematically neglected and that there is a crucial need to determine the impact of spatio-temporal heterogeneities on transport in the brain microcirculation.

JFM classification

Biological Fluid Dynamics: Blood flow Low-Reynolds-number flows: Porous media
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 884 , 10 February 2020 , A39
Copyright
© 2019 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abbott, N. J., Patabendige, A. A. K., Dolman, D. E. M., Yusof, S. R. & Begley, D. J. 2010 Structure and function of the blood-brain barrier. Neurobiol. Disease 37 (1), 13–25.CrossRefGoogle ScholarPubMed
Allaire, G., Brizzi, R., Mikelić, A. & Piatnitski, A. 2010 Two-scale expansion with drift approach to the Taylor dispersion for reactive transport through porous media. Chem. Engng Sci. 65 (7), 2292–2300.CrossRefGoogle Scholar
Alsop, D. C., Detre, J. A., Golay, X., Gunther, M., Hendrikse, J., Hernandez-Garcia, L., Lu, H., MacIntosh, B. J., Parkes, L. M., Smits, M. et al. 2015 Recommended implementation of arterial spin-labeled perfusion MRI for clinical applications: a consensus of the ISMRM perfusion study group and the European consortium for ASL in dementia. Magn. Reson. Med. 73 (1), 102–116.CrossRefGoogle Scholar
Aris, R. 1956 On the dispersion of a solute in a fluid flowing through a tube. Proc. R. Soc. Lond. A 235 (1200), 67–77.Google Scholar
Atkins, P. W. & De Paula, J. 2011 Physical Chemistry for the Life Sciences. Oxford University Press.Google Scholar
Auriault, J. L. 2005 Transport in porous media: upscaling by multiscale asymptotic expansions. In Applied Micromechanics of Porous Materials (ed. Dormieux, L. & Ulm, F.-J.), pp. 3–56. Springer.CrossRefGoogle Scholar
Auriault, J. L. & Adler, P. M. 1995 Taylor dispersion in porous media: analysis by multiple scale expansions. Adv. Water Resour. 18 (4), 217–226.CrossRefGoogle Scholar
Barnes, S. L., Quarles, C. C. & Yankeelov, T. E. 2014 Modeling the effect of intra-voxel diffusion of contrast agent on the quantitative analysis of dynamic contrast enhanced magnetic resonance imaging. PLoS ONE 9 (10), 1–12.CrossRefGoogle ScholarPubMed
Bate, H., Rowlands, S. & Sirs, J. A. 1973 Influence of diffusion on dispersion of indicators in blood flow. J. Appl. Physiol. 34 (6), 866–872.CrossRefGoogle ScholarPubMed
Baxter, L. T., Yuan, F. & Jain, R. K. 1992 Pharmacokinetic analysis of the perivascular distribution of bifunctional antibodies and haptens: comparison with experimental data. Cancer Res. 52 (20), 5838–5844.Google ScholarPubMed
Beard, D. A. 2001 Taylor dispersion of a solute in a microfluidic channel. J. Appl. Phys. 89 (8), 4667–4669.CrossRefGoogle Scholar
Bello, M. S., Rezzonico, R. & Righetti, P. G. 1994 Use of Taylor–Aris dispersion for measurement of a solute diffusion coefficient in thin capillaries. Science 266 (5186), 773–776.CrossRefGoogle ScholarPubMed
Benson, M. J., Elkins, C. J., Mobley, P. D., Alley, M. T. & Eaton, J. K. 2010 Three-dimensional concentration field measurements in a mixing layer using magnetic resonance imaging. Exp. Fluids 49 (1), 43–55.CrossRefGoogle Scholar
Bensoussan, A., Lions, J. L. & Papanicolaou, G. 1978 Asymptotic Analysis for Periodic Structures, vol. 374. North Holland.Google Scholar
Bishop, J. J., Popel, A. S., Intaglietta, M. & Johnson, P. C. 2002 Effect of aggregation and shear rate on the dispersion of red blood cells flowing in venules. Am. J. Physiol. Heart Circ. Physiol. 283 (5), 1985–1996.CrossRefGoogle ScholarPubMed
Blinder, P., Tsai, P. S., Kaufhold, J. P, Knutsen, P. M., Suhl, H. & Kleinfeld, D. 2013 The cortical angiome: an interconnected vascular network with noncolumnar patterns of blood flow. Nature Neurosci. 16 (7), 889–897.CrossRefGoogle ScholarPubMed
Brenner, H. & Stewartson, K. 1980 Dispersion resulting from flow through spatially periodic porous media. Phil. Trans. R. Soc. Lond. A 297 (1430), 81–133.CrossRefGoogle Scholar
Brundel, M., de Bresser, J., van Dillen, J. J., Kappelle, L. J. & Biessels, G. J. 2012 Cerebral microinfarcts: a systematic review of neuropathological studies. J. Cerebral Blood Flow Metabolism 32 (3), 425–436.CrossRefGoogle ScholarPubMed
Cruz-Hernández, J. C., Bracko, O., Kersbergen, C. J., Muse, V., Haft-Javaherian, M., Berg, M., Park, L., Vinarcsik, L. K., Ivasyk, I., Kang, Y. et al. 2019 Neutrophil adhesion in brain capillaries contributes to cortical blood flow decreases and impaired memory function in a mouse model of Alzheimer’s disease. Nature Neurosci. 22, 413–420.CrossRefGoogle Scholar
Damiano, E. R., Long, D. S. & Smith, M. L. 2004 Estimation of viscosity profiles using velocimetry data from parallel flows of linearly viscous fluids: application to microvascular haemodynamics. J. Fluid Mech. 512, 1–19.CrossRefGoogle Scholar
Davit, Y., Bell, C. G., Byrne, H. M., Chapman, L. A. C., Kimpton, L. S., Lang, G. E., Leonard, K. H. L., Oliver, J. M., Pearson, N. C., Shipley, R. J. et al. 2013 Homogenization via formal multiscale asymptotics and volume averaging: how do the two techniques compare? Adv. Water Resour. 62, 178–206.CrossRefGoogle Scholar
Denk, W., Strickler, J. H. & Webb, W. W. 1990 Two-photon laser scanning fluorescence microscopy. Science 248 (4951), 73–76.CrossRefGoogle ScholarPubMed
van Duijn, C. J., Mikelić, A., Pop, I. S. & Rosier, C. 2008 Chapter 1. Effective dispersion equations for reactive flows with dominant Péclet and Damkohler numbers. Adv. Chem. Engng 34, 1–45.CrossRefGoogle Scholar
Faghri, A. 1995 Heat Pipe Science and Technology. Taylor & Francis.Google Scholar
Fallon, M. S. & Anuj, C. 2005 Dispersion in core-annular flow with a solid annulus. AIChE J. 51 (9), 2415–2427.CrossRefGoogle Scholar
Fan, L. T. & Hwang, W. S. 1965 Dispersion of Ostwald–de Waele fluid in laminar flow through a cylindrical tube. Proc. R. Soc. Lond. A 283 (1395), 576–582.Google Scholar
Fang, Q., Sakadžić, S., Ruvinskaya, L., Devor, A., Dale, A. M. & Boas, D. A. 2008 Oxygen advection and diffusion in a three-dimensional vascular anatomical network. Opt. Express 16 (22), 17530–17541.CrossRefGoogle Scholar
Fry, B. C., Lee, J., Smith, N. P. & Secomb, T. W. 2012 Estimation of blood flow rates in large microvascular networks. Microcirculation 19 (6), 530–538.CrossRefGoogle ScholarPubMed
Gagnon, L., Sakadžić, S., Lesage, F., Musacchia, J. J., Lefebvre, J., Fang, Q., Yucel, M. A., Evans, K. C., Mandeville, E. T. et al. 2015 Quantifying the microvascular origin of BOLD-fMRI from first principles with two-photon microscopy and an oxygen-sensitive nanoprobe. J. Neurosci. 35 (8), 3663–3675.CrossRefGoogle ScholarPubMed
Gentile, F., Ferrari, M. & Decuzzi, P. 2008 The transport of nanoparticles in blood vessels: the effect of vessel permeability and blood rheology. Ann. Biomed. Engng 36 (2), 254–261.CrossRefGoogle ScholarPubMed
Goldman, D. M. & Popel, A. S. 1999 Computational modeling of oxygen transport from complex capillary networks. Relation to the microcirculation physiome. Adv. Exp. Med. Biol. 471, 555–563.CrossRefGoogle ScholarPubMed
Golfier, F., Quintard, M. & Whitaker, S. 2002 Heat and mass transfer in tubes: an analysis using the method of volume averaging. J. Porous Media 5 (04), 169–185.CrossRefGoogle Scholar
Gorelick, P. B., Scuteri, A., Black, S. E., Decarli, C., Greenberg, S. M., Iadecola, C., Launer, L. J., Laurent, S., Lopez, O. L., Nyenhuis, D. et al. 2011 Vascular contributions to cognitive impairment and dementia. Stroke 42 (9), 2672–2713.CrossRefGoogle ScholarPubMed
Gould, I. G., Tsai, P., Kleinfeld, D. & Linninger, A. 2016 The capillary bed offers the largest hemodynamic resistance to the cortical blood supply. J. Cerebral Blood Flow Metabolism 33 (1), 52–68.Google Scholar
Grinberg, O., Novozhilov, B., Grinberg, S., Friedman, B. & Swartz, H. M. 2005 Axial oxygen diffusion in the Krogh model. In Oxygen Transport to Tissue XXVI, pp. 127–134. Springer.CrossRefGoogle Scholar
Guibert, R., Fonta, C., Risser, L. & Plouraboué, F. 2012 Coupling and robustness of intra-cortical vascular territories. NeuroImage 62 (1), 408–417.CrossRefGoogle ScholarPubMed
Hellums, J. D. 1977 The resistance to oxygen transport in the capillaries relative to that in the surrounding tissue. Microvasc. Res. 13 (1), 131–136.CrossRefGoogle ScholarPubMed
Hellums, J. D., Nair, P. K., Huang, N. S. & Ohshima, N. 1995 Simulation of intraluminal gas transport processes in the microcirculation. Ann. Biomed. Engng 24 (1), 1–24.CrossRefGoogle Scholar
Holdsworth, S. J. & Bammer, R. 2008 Magnetic resonance imaging techniques: fMRI, DWI, and PWI. Seminars in Neurology 395–406.CrossRefGoogle ScholarPubMed
Holter, K. E., Kehlet, B., Devor, A., Sejnowski, T. J., Dale, A. M., Omholt, S. W., Ottersen, O. P., Nagelhus, E. A., Mardal, K. A. & Pettersen, K. H. 2017 Interstitial solute transport in 3D reconstructed neuropil occurs by diffusion rather than bulk flow. Proc. Natl Acad. Sci. USA 114 (37), 9894–9899.CrossRefGoogle ScholarPubMed
Hsu, R. & Secomb, T. W. 1989 A Green’s function method for analysis of oxygen delivery to tissue by microvascular networks. Math. Biosci. 96 (1), 61–78.CrossRefGoogle ScholarPubMed
Kabacaoglu, G., Quaife, B. & Biros, G. 2017 Quantification of mixing in vesicle suspensions using numerical simulations in two dimensions. Phys. Fluids 29 (2), 021901.CrossRefGoogle ScholarPubMed
Koch, D. L. & Brady, J. F. 1985 Dispersion in fixed beds. J. Fluid Mech. 154, 399–427.CrossRefGoogle Scholar
Kojic, M., Milosevic, M., Simic, V., Koay, E. J., Fleming, J. B., Nizzero, S., Kojic, N., Ziemys, A. & Ferrari, M. 2017 A composite smeared finite element for mass transport in capillary systems and biological tissue. Comput. Meth. Appl. Mech. Engng 324, 413–437.CrossRefGoogle ScholarPubMed
Kovačević, N., Henderson, J. T., Chan, E., Lifshitz, N., Bishop, J., Evans, A. C., Henkelman, R. M. & Chen, X. J. 2005 A three-dimensional MRI atlas of the mouse brain with estimates of the average and variability. Cerebral Cortex 15 (5), 639–645.CrossRefGoogle ScholarPubMed
Krogh, A. 1919 The number and distribution of capillaries in muscles with calculations of the oxygen pressure head necessary for supplying the tissue. J. Physiol. 52 (6), 409–415.CrossRefGoogle ScholarPubMed
Kutuzov, N., Flyvbjerg, H. & Lauritzen, M. 2018 Contributions of the glycocalyx, endothelium, and extravascular compartment to the blood–brain barrier. Proc. Natl Acad. Sci. USA 115 (40), 9429–9438.CrossRefGoogle ScholarPubMed
Lane, D. A. & Sirs, J. A. 1974 Indicator dilution measurement of mean transit time and flow in a straight tube. J. Phys. E 7 (1), 51–55.CrossRefGoogle Scholar
Lei, H., Fedosov, D. A., Caswell, B. & Karniadakis, G. E. 2013 Blood flow in small tubes: quantifying the transition to the non-continuum regime. J. Fluid Mech. 722, 214–239.CrossRefGoogle ScholarPubMed
Leonard, E. F. & Jørgensen, S. B. 1974 The analysis of convection and diffusion in capillary beds. Annu. Rev. Biophys. Bioengng 3 (1), 293–339.CrossRefGoogle ScholarPubMed
Levitt, D. G. 1972 Capillary-tissue exchange kinetics: an analysis of the Krogh cylinder model. J. Theor. Biol. 34 (1), 103–124.CrossRefGoogle ScholarPubMed
Lincoff, A. M., Borovetz, H. S. & Inskeep, W. H. 1983 Characterisation of the unsteady transport of labelled species in permeable capillaries: role of convective dispersion. Phys. Med. Biol. 28 (11), 1191–1208.CrossRefGoogle ScholarPubMed
Linninger, A. A., Gould, I. G., Marinnan, T., Hsu, C. Y., Chojecki, M. & Alaraj, A. 2013 Cerebral microcirculation and oxygen tension in the human secondary cortex. Ann. Biomed. Engng. 41 (11), 2264–2284.CrossRefGoogle ScholarPubMed
Lorthois, S. & Cassot, F. 2010 Fractal analysis of vascular networks: insights from morphogenesis. J. Theor. Biol. 262 (4), 614–633.CrossRefGoogle ScholarPubMed
Lorthois, S., Cassot, F. & Lauwers, F. 2011 Simulation study of brain blood flow regulation by intra-cortical arterioles in an anatomically accurate large human vascular network. Part I. Methodology and baseline flow. NeuroImage 54 (2), 1031–1042.CrossRefGoogle Scholar
Lorthois, S., Duru, P., Billanou, I., Quintard, M. & Celsis, P. 2014a Kinetic modeling in the context of cerebral blood flow quantification by H2O15 positron emission tomography: the meaning of the permeability coefficient in Renkin–Crone’s model revisited at capillary scale. J. Theor. Biol. 353, 157–169.CrossRefGoogle Scholar
Lorthois, S., Lauwers, F. & Cassot, F. 2014b Tortuosity and other vessel attributes for arterioles and venules of the human cerebral cortex. Microvasc. Res. 91, 99–109.CrossRefGoogle Scholar
Mei, C. C. 1992 Method of homogenization applied to dispersion in porous media. Transp. Porous Med. 9 (3), 261–274.CrossRefGoogle Scholar
Nicholson, C. 2001 Diffusion and related transport mechanisms in brain tissue. Rep. Prog. Phys. 64 (7), 815–884.CrossRefGoogle Scholar
Obrist, D., Weber, B., Buck, A. & Jenny, P. 2010 Red blood cell distribution in simplified capillary networks. Phil. Trans. R. Soc. Lond. A 368 (1921), 2897–2918.CrossRefGoogle ScholarPubMed
Peyrounette, M., Davit, Y., Quintard, M. & Lorthois, S. 2018 Multiscale modelling of blood flow in cerebral microcirculation: details at capillary scale control accuracy at the level of the cortex. PLoS ONE 13 (1), 1–35.CrossRefGoogle ScholarPubMed
Pries, A. R. & Secomb, T. W. 2005 Microvascular blood viscosity in vivo and the endothelial surface layer. Am. J. Physiol. Heart Circ. Physiol. 289 (6), 2657–2664.CrossRefGoogle ScholarPubMed
Pries, A. R., Ley, K., Claassen, M. & Gaehtgens, P. 1989 Red cell distribution at microvascular bifurcations. Microvasc. Res. 38 (1), 81–101.CrossRefGoogle ScholarPubMed
Pries, A. R., Secomb, T. W., Gaehtgens, P. & Gross, J. F. 1990 Blood flow in microvascular networks. Experiments and simulation. Circul. Res. 67 (4), 826–834.CrossRefGoogle ScholarPubMed
Pries, A. R., Secomb, T. W. & Gaehtgens, P. 1996 Biophysical aspects of blood flow in the microvasculature. Cardiovasc. Res. 32 (4), 654–667.CrossRefGoogle ScholarPubMed
Reay, D. A., Kew, P. A. J. & McGlen, R.(Eds) 2014 Heat Pipes, 6th edn. Butterworth-Heinemann.Google Scholar
Reneau, D. D., Bruley, D. F. & Knisely, M. H. 1969 A digital simulation of transient oxygen transport in capillary-tissue systems (cerebral grey matter). Development of a numerical method for solution of transport equations describing coupled convection-diffusion systems. AIChE J. 15 (6), 916–925.CrossRefGoogle Scholar
Roman, S., Lorthois, S., Duru, P. & Risso, F. 2012 Velocimetry of red blood cells in microvessels by the dual-slit method: effect of velocity gradients. Microvasc. Res. 84 (3), 249–261.CrossRefGoogle ScholarPubMed
Roman, S., Merlo, A., Duru, P., Risso, F. & Lorthois, S. 2016 Going beyond 20 μm-sized channels for studying red blood cell phase separation in microfluidic bifurcations. Biomicrofluidics 10 (3), 034103.CrossRefGoogle ScholarPubMed
Safaeian, N. & David, T. 2013 A computational model of oxygen transport in the cerebrocapillary levels for normal and pathologic brain function. J. Cerebral Blood Flow Metabolism 33 (10), 1633–1641.CrossRefGoogle ScholarPubMed
Sakadžić, S., Mandeville, E. T., Gagnon, L., Musacchia, J. J., Yaseen, M. A., Yucel, M. A., Lefebvre, J., Lesage, F., Dale, A. M. et al. 2014 Large arteriolar component of oxygen delivery implies a safe margin of oxygen supply to cerebral tissue. Nature Commun. 5, 5734.CrossRefGoogle ScholarPubMed
Santisakultarm, T. P., Cornelius, N. R., Nishimura, N., Schafer, A. I., Silver, R. T., Doerschuk, P. C., Olbricht, W. L. & Schaffer, C. B. 2012 In vivo two-photon excited fluorescence microscopy reveals cardiac- and respiration-dependent pulsatile blood flow in cortical blood vessels in mice. Am. J. Physiol.: Heart Circulatory Physiol. 302 (7), 1367–1377.Google ScholarPubMed
Schmid, F., Tsai, P. S., Kleinfeld, D., Jenny, P. & Weber, B. 2017 Depth-dependent flow and pressure characteristics in cortical microvascular networks. PLOS Comput. Biol. 13 (2), e1005392.CrossRefGoogle ScholarPubMed
Secomb, T. W. 2015 Krogh-cylinder and infinite-domain models for washout of an inert diffusible solute from tissue. Microcirculation 22 (1), 91–98.CrossRefGoogle Scholar
Secomb, T. W., Hsu, R., Park, E. Y. H. & Dewhirst, M. W. 2004 Green’s function methods for analysis of oxygen delivery to tissue by microvascular networks. Ann. Biomed. Engng 32 (11), 1519–1529.CrossRefGoogle ScholarPubMed
Secomb, T. W. 2017 Blood flow in the microcirculation. Annu. Rev. Fluid Mech. 49 (1), 443–461.CrossRefGoogle Scholar
Shapiro, M. & Brenner, H. 1986 Taylor dispersion of chemically reactive species: irreversible first-order reactions in bulk and on boundaries. Chem. Engng Sci. 41 (6), 1417–1433.CrossRefGoogle Scholar
Sherwood, J. M., Holmes, D., Kaliviotis, E. & Balabani, S. 2014 Spatial distributions of red blood cells significantly alter local haemodynamics. PLoS ONE 9 (6), 1–13.CrossRefGoogle ScholarPubMed
Shih, A. Y., Driscoll, J. D., Drew, P. J., Nishimura, N., Schaffer, C. B. & Kleinfeld, D. 2012 Two-photon microscopy as a tool to study blood flow and neurovascular coupling in the rodent brain. J. Cerebral Blood Flow Metabolism 32 (7), 1277–1309.CrossRefGoogle ScholarPubMed
Sweeney, P. W., Walker-Samuel, S. & Shipley, R. J. 2018 Insights into cerebral haemodynamics and oxygenation utilising in vivo mural cell imaging and mathematical modelling. Sci. Rep. 8, 1373.CrossRefGoogle ScholarPubMed
Tang, J., Erdener, S. E., Li, B., Fu, B., Sakadzic, S. A., Carp, S., Lee, J. & Boas, D. A. 2018 Shear-induced diffusion of red blood cells measured with dynamic light scattering-optical coherence tomography. J. Biophotonics 11 (2), e201700070.CrossRefGoogle ScholarPubMed
Taylor, G. I. 1953 Dispersion of soluble matter in solvent flowing slowly through a tube. Proc. R. Soc. Lond. A 219 (1137), 186–203.Google Scholar
Taylor, Z. J., Hui, E. S., Watson, A. N., Nie, X., Deardorff, R. L., Jensen, J. H., Helpern, J. A. & Shih, A. Y. 2016 Microvascular basis for growth of small infarcts following occlusion of single penetrating arterioles in mouse cortex. J. Cerebral Blood Flow Metabolism 36 (8), 1357–1373.CrossRefGoogle ScholarPubMed
Tepper, R. S., Lee, H. L. & Lightfoot, E. N. 1978 Transient convective mass transfer in Krogh tissue cylinders. Ann. Biomed. Engng 6 (4), 506–530.CrossRefGoogle ScholarPubMed
Tsai, P. S., Kaufhold, J. P., Blinder, P., Friedman, B., Drew, P. J., Karten, H. J., Lyden, P. D. & Kleinfeld, D. 2009 Correlations of neuronal and microvascular densities in murine cortex revealed by direct counting and colocalization of nuclei and vessels. J. Neurosci. 29 (46), 14553–14570.CrossRefGoogle ScholarPubMed
Vikhansky, A. & Wang, W. 2011 Taylor dispersion in finite-length capillaries. Chem. Engng Sci. 66 (4), 642–649.CrossRefGoogle Scholar
Weigl, B. H. & Yager, P. 1999 Microfluidic diffusion-based separation and detection. Science 283 (5400), 346–347.CrossRefGoogle Scholar
Whitaker, S. 1999 The Method of Volume Averaging. Springer.CrossRefGoogle Scholar
Wieseotte, C., Wagner, M. & Schreiber, L. M. 2014 An estimate of Gd-DOTA diffusivity in blood by direct NMR diffusion measurement of its hydrodynamic analogue Ga-DOTA. In Conference Paper, ISMRM Annual Meeting, International Society of Magnetic Resonance in Medicine.Google Scholar
Zlokovic, B. V. 2011 Neurovascular pathways to neurodegeneration in Alzheimer’s disease and other disorders. Nature Rev. Neurosci. 12 (12), 723–738.CrossRefGoogle ScholarPubMed

Berg et al. supplementary movie

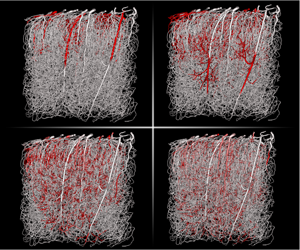
Transient numerical solution (1s) of average concentration of an intravascular tracer with a high diffusion coefficient ($D_V=10^{-9}m^2.s^{-1}$) resulting from a square input of 0.1s duration. This solution has been obtained using the WCA model on the network shown in figure 2a and using the velocity field computed in Cruz-Hernández et al. (2019).

Download Berg et al. supplementary movie(Video)
Video 7.7 MB