Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Shishkina, Olga
2017.
Progress in Turbulence VII.
Vol. 196,
Issue. ,
p.
245.
Vinnichenko, Nikolay A.
Pushtaev, Alexey V.
Plaksina, Yulia Yu.
Rudenko, Yulia K.
and
Uvarov, Alexander V.
2018.
Horizontal convection driven by nonuniform radiative heating in liquids with different surface behavior.
International Journal of Heat and Mass Transfer,
Vol. 126,
Issue. ,
p.
400.
Nandukumar, Yada
Chakraborty, Suman
Verma, Mahendra K.
and
Lakkaraju, Rajaram
2019.
On heat transport and energy partition in thermal convection with mixed boundary conditions.
Physics of Fluids,
Vol. 31,
Issue. 6,
Hossain, Sajjad
Vo, Tony
and
Sheard, Gregory J.
2019.
Horizontal convection in shallow enclosures scales with height, not length, at low Rayleigh numbers.
International Communications in Heat and Mass Transfer,
Vol. 109,
Issue. ,
p.
104308.
Cox, Grace A.
Davies, Christopher J.
Livermore, Philip W.
and
Singleton, James
2019.
Penetration of boundary-driven flows into a rotating spherical thermally stratified fluid.
Journal of Fluid Mechanics,
Vol. 864,
Issue. ,
p.
519.
Tsai, Tzekih
Hussam, Wisam K.
King, Martin P.
and
Sheard, Gregory J.
2020.
Transitions and scaling in horizontal convection driven by different temperature profiles.
International Journal of Thermal Sciences,
Vol. 148,
Issue. ,
p.
106166.
Reiter, Philipp
and
Shishkina, Olga
2020.
Classical and symmetrical horizontal convection: detaching plumes and oscillations.
Journal of Fluid Mechanics,
Vol. 892,
Issue. ,
Noto, Daisuke
Terada, Tomomi
Yanagisawa, Takatoshi
Miyagoshi, Takehiro
and
Tasaka, Yuji
2021.
Developing horizontal convection against stable temperature stratification in a rectangular container.
Physical Review Fluids,
Vol. 6,
Issue. 8,
Yan, Bo
Shishkina, Olga
and
He, Xiaozhou
2021.
Thermal boundary-layer structure in laminar horizontal convection.
Journal of Fluid Mechanics,
Vol. 915,
Issue. ,
Ding, Guang-Yu
Chong, Kai Leong
and
Xia, Ke-Qing
2021.
A comparative study of linear and step forcing temperature profiles in horizontal convection
(a)
.
EPL (Europhysics Letters),
Vol. 135,
Issue. 2,
p.
24006.
Shishkina, Olga
2021.
Rayleigh-Bénard convection: The container shape matters.
Physical Review Fluids,
Vol. 6,
Issue. 9,
Ding, Guang-Yu
He, Yu-Hao
and
Xia, Ke-Qing
2022.
The effect of tidal force and topography on horizontal convection.
Journal of Fluid Mechanics,
Vol. 932,
Issue. ,
Qi, Yi
Chen, Enhui
and
Xu, Feng
2022.
A transient intrusion flow on a cooled horizontal surface.
Physics of Fluids,
Vol. 34,
Issue. 1,
Kar, Prabir Kumar
Chetan, Ujjwal
Mahato, Jagannath
Sahu, Toshan Lal
Das, P. K.
and
Lakkaraju, Rajaram
2022.
Heat flux enhancement by regular surface protrusion in partitioned thermal convection.
Physics of Fluids,
Vol. 34,
Issue. 12,
Rudenko, Yu.K.
Vinnichenko, N.A.
Plaksina, Yu.Yu.
Pushtaev, A.V.
and
Uvarov, A.V.
2022.
Horizontal convective flow from a line heat source located at the liquid–gas interface in presence of surface film.
Journal of Fluid Mechanics,
Vol. 944,
Issue. ,
Yan, Bo
and
He, Xiaozhou
2022.
Transitional Fluctuations of Thermal Boundary Layer in Horizontal Convection.
SSRN Electronic Journal ,
Yan, Bo
and
He, Xiaozhou
2023.
Transitional fluctuations of thermal boundary layer in horizontal convection.
International Journal of Heat and Mass Transfer,
Vol. 200,
Issue. ,
p.
123542.
Lohse, Detlef
and
Shishkina, Olga
2024.
Ultimate Rayleigh-Bénard turbulence.
Reviews of Modern Physics,
Vol. 96,
Issue. 3,
 $\unicode[STIX]{x1D6E4}=10$, Rayleigh number
$\unicode[STIX]{x1D6E4}=10$, Rayleigh number 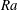 $\mathit{Ra}$ from
$\mathit{Ra}$ from 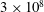 $3\times 10^{8}$ to
$3\times 10^{8}$ to 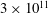 $3\times 10^{11}$ and Prandtl number
$3\times 10^{11}$ and Prandtl number 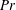 $\mathit{Pr}$ from 0.05 to 50. The structure of the mean flows in horizontal convection is described in terms of time-averaged spatial distributions of the temperature, velocity, kinetic energy, thermal and kinetic dissipation rates. A possible scenario of transition to turbulent horizontal convection in the whole convection cell of a large aspect ratio is discussed.
$\mathit{Pr}$ from 0.05 to 50. The structure of the mean flows in horizontal convection is described in terms of time-averaged spatial distributions of the temperature, velocity, kinetic energy, thermal and kinetic dissipation rates. A possible scenario of transition to turbulent horizontal convection in the whole convection cell of a large aspect ratio is discussed.