Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-05T04:14:07.544Z Has data issue: false hasContentIssue false
  • English
  • Français

Laminar–turbulent coexistence in annular Couette flow

Published online by Cambridge University Press:  01 October 2019

Kohei Kunii ,
Takahiro Ishida ,
Yohann Duguet Open the ORCID record for Yohann Duguet [Opens in a new window]  and
Takahiro Tsukahara Open the ORCID record for Takahiro Tsukahara [Opens in a new window]
Kohei Kunii
Affiliation:
Department of Mechanical Engineering, Tokyo University of Science, Chiba 278-8510, Japan
Takahiro Ishida
Affiliation:
Department of Mechanical Engineering, Tokyo University of Science, Chiba 278-8510, Japan
Yohann Duguet
Affiliation:
LIMSI-CNRS, Université Paris-Sud, Université Paris-Saclay, F-91405 Orsay, France
Takahiro Tsukahara*
Affiliation:
Department of Mechanical Engineering, Tokyo University of Science, Chiba 278-8510, Japan
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Annular Couette flow is the flow between two coaxial cylinders driven by the axial translation of the inner cylinder. It is investigated using direct numerical simulation in long domains, with an emphasis on the laminar–turbulent coexistence regime found for marginally low values of the Reynolds number. Three distinct flow regimes are demonstrated as the radius ratio 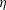$\unicode[STIX]{x1D702}$ is decreased from 0.8 to 0.5 and finally to 0.1. The high-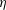$\unicode[STIX]{x1D702}$ regime features helically shaped turbulent patches coexisting with laminar flow, as in planar shear flows. The moderate-$\unicode[STIX]{x1D702}$ regime does not feature any marked laminar–turbulent coexistence. In an effort to discard confinement effects, proper patterning is, however, recovered by artificially extending the azimuthal span beyond 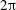$2\unicode[STIX]{x03C0}$. Eventually, the low-$\unicode[STIX]{x1D702}$ regime features localised turbulent structures different from the puffs commonly encountered in transitional pipe flow. In this new coexistence regime, turbulent fluctuations are surprisingly short-ranged. Implications are discussed in terms of phase transition and critical scaling.

JFM classification

Turbulent Flows: Turbulent transition Nonlinear Dynamical Systems: Pattern formation
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 879 , 25 November 2019 , pp. 579 - 603
Check for updates
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

‡

Present address: Japan Aerospace Exploration Agency, Tokyo 182-8522, Japan.

References

Abe, H., Kawamura, H. & Matsuo, Y. 2001 Direct numerical simulation of a fully developed turbulent channel flow with respect to the Reynolds number dependence. J. Fluids Engng 123 (2), 382–393.10.1115/1.1366680Google Scholar
Alfredsson, P. H. & Matsubara, M. 2000 Free-stream turbulence, streaky structures and transition in boundary layer flows. AIAA Paper 2000–2543.Google Scholar
Avila, K., Moxey, D., de Lozar, A., Avila, M., Barkley, D. & Hof, B. 2011 The onset of turbulence in pipe flow. Science 333 (6039), 192–196.10.1126/science.1203223Google Scholar
Bandyopadhyay, P. R. 1986 Aspects of the equilibrium puff in transitional pipe flow. J. Fluid Mech. 163, 439–458.Google Scholar
Barkley, D., Song, B., Mukund, V., Lemoult, G., Avila, M. & Hof, B. 2015 The rise of fully turbulent flow. Nature 526 (7574), 550–553.10.1038/nature15701Google Scholar
Barkley, D. & Tuckerman, L. S. 2005 Computational study of turbulent laminar patterns in couette flow. Phys. Rev. Lett. 94 (1), 014502.Google Scholar
Barkley, D. & Tuckerman, L. S. 2007 Mean flow of turbulent–laminar patterns in plane Couette flow. J. Fluid Mech. 576, 109–137.10.1017/S002211200600454XGoogle Scholar
Bottin, S., Daviaud, F., Manneville, P. & Dauchot, O. 1998 Discontinuous transition to spatiotemporal intermittency in plane Couette flow. Europhys. Lett. 43 (2), 171–176.10.1209/epl/i1998-00336-3Google Scholar
Brethouwer, G., Duguet, Y. & Schlatter, P. 2012 Turbulent-laminar coexistence in wall flows with coriolis, buoyancy or Lorentz forces. J. Fluid Mech. 704, 137–172.10.1017/jfm.2012.224Google Scholar
Chantry, M., Tuckerman, L. S. & Barkley, D. 2017 Universal continuous transition to turbulence in a planar shear flow. J. Fluid Mech. 824, R1.10.1017/jfm.2017.405Google Scholar
Coles, D. 1965 Transition in circular Couette flow. J. Fluid Mech. 21 (3), 385–425.10.1017/S0022112065000241Google Scholar
Deguchi, K. & Nagata, M. 2011 Bifurcations and instabilities in sliding Couette flow. J. Fluid Mech. 678, 156–178.Google Scholar
Duguet, Y. & Schlatter, P. 2013 Oblique laminar–turbulent interfaces in plane shear flows. Phys. Rev. Lett. 110 (3), 034502.10.1103/PhysRevLett.110.034502Google Scholar
Duguet, Y., Schlatter, P. & Henningson, D. S. 2010a Formation of turbulent patterns near the onset of transition in plane Couette flow. J. Fluid Mech. 650, 119–129.10.1017/S0022112010000297Google Scholar
Duguet, Y., Willis, A. P. & Kerswell, R. R. 2010b Slug genesis in cylindrical pipe flow. J. Fluid Mech. 663, 180–208.10.1017/S0022112010003435Google Scholar
Faisst, H. & Eckhardt, B. 2000 Transition from the Couette–Taylor system to the plane Couette system. Phys. Rev. E 61 (6), 7227.Google Scholar
Frei, C., Lüscher, P. & Wintermantel, E. 2000 Thread-annular flow in vertical pipes. J. Fluid Mech. 410, 185–210.Google Scholar
Fukudome, K. & Iida, O. 2012 Large-scale flow structure in turbulent Poiseuille flows at low-Reynolds numbers. J. Fluid Sci. Technol. 7 (1), 181–195.10.1299/jfst.7.181Google Scholar
Gittler, P. 1993 Stability of axial Poiseuille–Couette flow between concentric cylinders. Acta Mech. 101 (1–4), 1–13.10.1007/BF01175593Google Scholar
Hof, B., de Lozar, A., Avila, M., Tu, X. & Schneider, T. M. 2010 Eliminating turbulence in spatially intermittent flows. Science 327 (5972), 1491–1494.10.1126/science.1186091Google Scholar
Hof, B., Westerweel, J., Schneider, T. M. & Eckhardt, B. 2006 Finite lifetime of turbulence in shear flows. Nature 443 (7107), 59–62.10.1038/nature05089Google Scholar
Ishida, T., Duguet, Y. & Tsukahara, T. 2016 Transitional structures in annular Poiseuille flow depending on radius ratio. J. Fluid Mech. 794, R2.10.1017/jfm.2016.192Google Scholar
Ishida, T., Duguet, Y. & Tsukahara, T. 2017 Turbulent bifurcations in intermittent shear flows: from puffs to oblique stripes. Phys. Rev. Fluids 2, 073902.10.1103/PhysRevFluids.2.073902Google Scholar
Lemoult, G., Shi, L., Avila, K., Jalikop, S. V., Avila, M. & Hof, B. 2016 Directed percolation phase transition to sustained turbulence in Couette flow. Nat. Phys. 12, 254–258.10.1038/nphys3675Google Scholar
Liu, R. & Liu, Q.-S. 2012 Non-modal stability in sliding Couette flow. J. Fluid Mech. 710, 505–544.Google Scholar
Nagata, M. 1990 Three-dimensional finite-amplitude solutions in plane Couette flow: bifurcation from infinity. J. Fluid Mech. 217, 519–527.10.1017/S0022112090000829Google Scholar
Orszag, S. A. 1971 Accurate solution of the Orr–Sommerfeld stability equation. J. Fluid Mech. 50 (4), 689–703.10.1017/S0022112071002842Google Scholar
Prigent, A., Grégoire, G., Chaté, H., Dauchot, O. & van Saarloos, W. 2002 Large-scale finite-wavelength modulation within turbulent shear flows. Phys. Rev. Lett. 89 (1), 014501.Google Scholar
Robertson, J. M. 1959 On turbulent plane-Couette flow. In Proceedings of the Sixth Midwestern Conference on Fluid Mech., pp. 169–182. University of Austin, Texas.Google Scholar
Samanta, D., de Lozar, A. & Hof, B. 2011 Experimental investigation of laminar turbulent intermittency in pipe flow. J. Fluid Mech. 681, 193–204.10.1017/jfm.2011.189Google Scholar
Sano, M. & Tamai, K. 2016 A universal transition to turbulence in channel flow. Nat. Phys. 12, 249–253.10.1038/nphys3659Google Scholar
Schmid, P. J. & Henningson, D. S. 2001 Stability and Transition in Shear Flows, Applied Mathematical Sciences, vol. 142. Springer.10.1007/978-1-4613-0185-1Google Scholar
Seki, D. & Matsubara, M. 2012 Experimental investigation of relaminarizing and transitional channel flows. Phys. Fluids 24 (12), 124102.10.1063/1.4772065Google Scholar
Shands, J., Alfredsson, P. H. & Lindgren, E. R. 1980 Annular pipe flow subject to axial motion of the inner boundary. Phys. Fluids 23 (10), 2144–2145.10.1063/1.862871Google Scholar
Shimizu, M. & Kida, S. 2009 A driving mechanism of a turbulent puff in pipe flow. Fluid Dyn. Res. 41 (4), 045501.Google Scholar
Shimizu, M., Manneville, P., Duguet, Y. & Kawahara, G. 2014 Splitting of a turbulent puff in pipe flow. Fluid Dyn. Res. 46 (6), 061403.Google Scholar
Tsukahara, T., Kawaguchi, Y., Kawamura, H., Tillmark, N. & Alfredsson, P. H. 2010 Turbulence stripe in transitional channel flow with/without system rotation. In Proceedings of Seventh IUTAM Symposium on Laminar–Turbulent Transition (ed. Schlatter, P. & Henningson, D. S.), pp. 421–426. Springer.10.1007/978-90-481-3723-7_68Google Scholar
Tsukahara, T., Kawamura, H. & Shingai, K. 2006 DNS of turbulent Couette flow with emphasis on the large-scale structure in the core region. J. Turbul. 7, N19.10.1080/14685240600609866Google Scholar
Tsukahara, T., Seki, Y., Kawamura, H. & Tochio, D. 2005 DNS of turbulent channel flow at very low Reynolds numbers. In Proceedings of the Fourth International Symposium on Turbulence and Shear Flow Phenomena, TSFP DIGITAL LIBRARY ONLINE (ed. Humphrey, J. A. C. et al. ), pp. 935–940. Begel House Inc.Google Scholar
Tsukahara, T., Tillmark, N. & Alfredsson, P. H. 2010b Flow regimes in a plane Couette flow with system rotation. J. Fluid Mech. 648, 5–33.10.1017/S0022112009993880Google Scholar
Walton, A. G. 2003 The nonlinear instability of thread–annular flow at high Reynolds number. J. Fluid Mech. 477, 227–257.10.1017/S0022112002003002Google Scholar
Walton, A. G. 2004 Stability of circular Poiseuille–Couette flow to axisymmetric disturbances. J. Fluid Mech. 500, 169–210.10.1017/S0022112003007158Google Scholar
Walton, A. G. 2005 The linear and nonlinear stability of thread-annular flow. Phil. Trans. R. Soc. Lond. A 363 (1830), 1223–1233.Google Scholar
Webber, M. 2008 Instability of thread-annular flow with small characteristic length to three-dimensional disturbances. Phil. Trans. R. Soc. Lond. A 464 (2091), 673–690.Google Scholar
Willis, A. P. & Kerswell, R. R. 2008 Coherent structures in localized and global pipe turbulence. Phys. Rev. Lett. 100 (12), 124501.Google Scholar
Wygnanski, I. J. & Champagne, F. H. 1973 On transition in a pipe. Part 1. The origin of puffs and slugs and the flow in a turbulent slug. J. Fluid Mech. 59 (2), 281–335.Google Scholar
Wygnanski, I., Sokolov, M. & Friedman, D. 1975 On transition in a pipe. Part 2. The equilibrium puff. J. Fluid Mech. 69 (2), 283–304.Google Scholar

Kunii et al. supplementary movie

Time evolution of fluctuating velocity fields viewed at mid-gap (top) and on a cross section (bottom three panels), for η = 0.1, Lθ= 16π, and Re = 275, where the new flow regime has been observed. From top to bottom, contours show x-z (or x-θ) distribution of ur', and r-θ views of the streamwise ux', radial ur', and azimuthal components uθ', at the same time. The time span visualized here is 800 time units (h/uw).

Download Kunii et al. supplementary movie(Video)
Video 37.4 MB