Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Le Rallic, Maxime
Jordan, Peter
and
Gervais, Yves
2016.
Jet-noise reduction: the effect of azimuthal actuation modes.
Le Rallic, Maxime
Jordan, Peter
and
Gervais, Yves
2017.
Understanding jet-noise reduction with wavepackets.
Mourão Soares, Luiz Fernando
Cavalieri, André V.
Kopiev, Victor
and
Faranosov, Georgy
2017.
Flight effects on turbulent-jet wavepackets.
Kleine, Vitor G.
Sasaki, Kenzo
Cavalieri, André V.
Brès, Guillaume A.
and
Colonius, Tim
2017.
Evaluation of PSE as a Model for Supersonic Jet Using Transfer Functions.
Sasaki, Kenzo
Piantanida, Selene
Cavalieri, André V. G.
and
Jordan, Peter
2017.
Real-time modelling of wavepackets in turbulent jets.
Journal of Fluid Mechanics,
Vol. 821,
Issue. ,
p.
458.
Sasaki, Kenzo
Cavalieri, André V. G.
Jordan, Peter
Schmidt, Oliver T.
Colonius, Tim
and
Brès, Guillaume A.
2017.
High-frequency wavepackets in turbulent jets.
Journal of Fluid Mechanics,
Vol. 830,
Issue. ,
Sasaki, Kenzo
Tissot, Gilles
Cavalieri, André V. G.
Silvestre, Flávio J.
Jordan, Peter
and
Biau, Damien
2018.
Closed-loop control of a free shear flow: a framework using the parabolized stability equations.
Theoretical and Computational Fluid Dynamics,
Vol. 32,
Issue. 6,
p.
765.
Kopiev, Victor
Faranosov, Georgy
Kopiev, Vladimir A.
Bychkov, Oleg P.
Moralev, Ivan
and
Kazansky, Pavel
2019.
Plasma-based active closed-loop control of instability waves in unexcited turbulent jet. Part 2. Installed jet..
Cavalieri, André V. G.
Jordan, Peter
and
Lesshafft, Lutz
2019.
Wave-Packet Models for Jet Dynamics and Sound Radiation.
Applied Mechanics Reviews,
Vol. 71,
Issue. 2,
Edgington-Mitchell, Daniel
2019.
Aeroacoustic resonance and self-excitation in screeching and impinging supersonic jets – A review.
International Journal of Aeroacoustics,
Vol. 18,
Issue. 2-3,
p.
118.
Lajús, F. C.
Sinha, A.
Cavalieri, A. V. G.
Deschamps, C. J.
and
Colonius, T.
2019.
Spatial stability analysis of subsonic corrugated jets.
Journal of Fluid Mechanics,
Vol. 876,
Issue. ,
p.
766.
Faranosov, Georgy
Bychkov, Oleg P.
Kopiev, Victor
Kopiev, Vladimir A.
Moralev, Ivan
and
Kazansky, Pavel
2019.
Plasma-based active closed-loop control of instability waves in unexcited turbulent jet. Part 1. Free jet..
Zhou, Yu
Fan, Dewei
Zhang, Bingfu
Li, Ruiying
and
Noack, Bernd R.
2020.
Artificial intelligence control of a turbulent jet.
Journal of Fluid Mechanics,
Vol. 897,
Issue. ,
Kopiev, Victor
Faranosov, Georgy
Bychkov, Oleg
Kopiev, Vladimir
Moralev, Ivan
and
Kazansky, Pavel
2020.
Active control of jet-plate interaction noise for excited jets by plasma actuators.
Journal of Sound and Vibration,
Vol. 484,
Issue. ,
p.
115515.
Soares, Luiz F. M.
Cavalieri, André V. G.
Kopiev, Victor
and
Faranosov, Georgy
2020.
Flight Effects on Turbulent-Jet Wave Packets.
AIAA Journal,
Vol. 58,
Issue. 9,
p.
3877.
Heidt, Liam
Colonius, Tim
Nekkanti, Akhil
Schmdit, Oliver
Maia, Igor
and
Jordan, Peter
2021.
Analysis of forced subsonic jets using spectral proper orthogonal decomposition and resolvent analysis.
Kopiev, V. F.
Bychkov, O. P.
Kopiev, V. A.
Faranosov, G. A.
Moralev, I. A.
and
Kazansky, P. N.
2021.
Control of Instability Waves in an Unexcited Turbulent Jet Using Plasma Actuators in a Narrow Frequency Band.
Acoustical Physics,
Vol. 67,
Issue. 4,
p.
413.
Bychkov, O. P.
Kopiev, V. F.
and
Faranosov, G. A.
2022.
Azimuthal Decomposition of the Noise of a Dual-Stream Coaxial Jet.
Acoustical Physics,
Vol. 68,
Issue. 4,
p.
371.
Bychkov, Oleg
Faranosov, Georgy
Kopiev, Victor
Soares, Luiz F. M.
and
Cavalieri, André V. G.
2022.
Jet Installation Noise Modeling in Static and Flight Conditions Using Centerline Fluctuations.
AIAA Journal,
Vol. 60,
Issue. 6,
p.
3620.
Maia, Igor A.
Jordan, Peter
and
Cavalieri, André V. G.
2022.
Wave cancellation in jets with laminar and turbulent boundary layers: The effect of nonlinearity.
Physical Review Fluids,
Vol. 7,
Issue. 3,
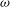 ${\it\omega}$–
${\it\omega}$–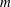 $m$) combination to which the mean flow is stable. When the actuation excites the flow with an
$m$) combination to which the mean flow is stable. When the actuation excites the flow with an  ${\it\omega}$–
${\it\omega}$–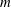 $m$ combination to which the mean flow is unstable, the response is dominated by coherent structures, whose rapid growth takes them beyond the linear limit, where they undergo quadratic wave interactions and lead, consequently, to a louder flow.
$m$ combination to which the mean flow is unstable, the response is dominated by coherent structures, whose rapid growth takes them beyond the linear limit, where they undergo quadratic wave interactions and lead, consequently, to a louder flow.