Published online by Cambridge University Press: 05 January 2017
The phenomenon of drag crisis for uniform flow past a smooth sphere is investigated via experiments in a low-turbulence wind tunnel for 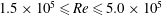 $1.5\times 10^{5}\leqslant Re\leqslant 5.0\times 10^{5}$. The Reynolds number,
$1.5\times 10^{5}\leqslant Re\leqslant 5.0\times 10^{5}$. The Reynolds number, 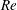 $Re$, is based on the free-stream speed and the diameter of the sphere. Based on the activity related to the laminar separation bubble (LSB), the critical regime for the occurrence of drag crisis (
$Re$, is based on the free-stream speed and the diameter of the sphere. Based on the activity related to the laminar separation bubble (LSB), the critical regime for the occurrence of drag crisis ( $3.4\times 10^{5}<Re<4.4\times 10^{5}$) is further divided into three subregimes. The gradual decrease of mean drag coefficient (
$3.4\times 10^{5}<Re<4.4\times 10^{5}$) is further divided into three subregimes. The gradual decrease of mean drag coefficient (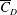 $\overline{C}_{D}$) with
$\overline{C}_{D}$) with 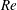 $Re$, in subregime I, is due to the increase of base pressure and suction near the shoulder of the sphere. The flow is devoid of an LSB in this regime. The coefficient
$Re$, in subregime I, is due to the increase of base pressure and suction near the shoulder of the sphere. The flow is devoid of an LSB in this regime. The coefficient 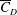 $\overline{C}_{D}$ decreases very rapidly with increase in
$\overline{C}_{D}$ decreases very rapidly with increase in  $Re$ in subregime II primarily due to the increase in mean base pressure (
$Re$ in subregime II primarily due to the increase in mean base pressure (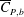 $\overline{C}_{P,b}$). This subregime is characterized by intermittent switching of
$\overline{C}_{P,b}$). This subregime is characterized by intermittent switching of  $C_{D}$ and
$C_{D}$ and 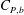 $C_{P,b}$ between bistable states. Statistical analysis of the surface-pressure and force coefficients relates this behaviour to the intermittent appearance/disappearance of the LSB. The two states of the flow are referred to as the LSB and non-LSB states. The frequency of appearance of the LSB and the duration of its stay increase with increase in
$C_{P,b}$ between bistable states. Statistical analysis of the surface-pressure and force coefficients relates this behaviour to the intermittent appearance/disappearance of the LSB. The two states of the flow are referred to as the LSB and non-LSB states. The frequency of appearance of the LSB and the duration of its stay increase with increase in 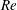 $Re$. An intermittency factor
$Re$. An intermittency factor 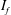 $I_{f}$, defined as the fraction of time during which the LSB exists in the flow, is estimated at each
$I_{f}$, defined as the fraction of time during which the LSB exists in the flow, is estimated at each 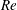 $Re$. The value of
$Re$. The value of  $I_{f}$ is zero in subregime I and increases from zero to one, with increase in
$I_{f}$ is zero in subregime I and increases from zero to one, with increase in 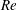 $Re$, in subregime II. The variation of
$Re$, in subregime II. The variation of 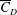 $\overline{C}_{D}$ with
$\overline{C}_{D}$ with  $Re$ is found to follow the variation of (
$Re$ is found to follow the variation of ( $1-I_{f}$) with
$1-I_{f}$) with  $Re$. This shows that the decrease of
$Re$. This shows that the decrease of  $\overline{C}_{D}$ with increase in
$\overline{C}_{D}$ with increase in  $Re$, during drag crisis, is primarily due to the increased probability of the LSB state as opposed to the non-LSB state. A spatio-temporal analysis of the surface pressure measured at various polar locations on the surface of the sphere confirms the axisymmetric nature of the intermittent LSB. In subregime III of the critical regime, the LSB exists at all time instants (
$Re$, during drag crisis, is primarily due to the increased probability of the LSB state as opposed to the non-LSB state. A spatio-temporal analysis of the surface pressure measured at various polar locations on the surface of the sphere confirms the axisymmetric nature of the intermittent LSB. In subregime III of the critical regime, the LSB exists at all time instants (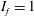 $I_{f}=1$). The
$I_{f}=1$). The  $\overline{C}_{D}$ value continues to decrease with
$\overline{C}_{D}$ value continues to decrease with 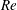 $Re$ in this subregime due to increase in
$Re$ in this subregime due to increase in 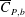 $\overline{C}_{P,b}$. Unlike the general belief that the decrease in
$\overline{C}_{P,b}$. Unlike the general belief that the decrease in  $\overline{C}_{D}$ with increase in
$\overline{C}_{D}$ with increase in 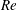 $Re$ is due only to the increase in
$Re$ is due only to the increase in  $\overline{C}_{P,b}$, it is found that the increase in suction upstream of the shoulder of the sphere, with increase in
$\overline{C}_{P,b}$, it is found that the increase in suction upstream of the shoulder of the sphere, with increase in 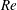 $Re$, also plays an important role. In particular, in the high subcritical regime (
$Re$, also plays an important role. In particular, in the high subcritical regime ( $2.5\times 10^{5}<Re<3.4\times 10^{5}$), the gradual decrease in
$2.5\times 10^{5}<Re<3.4\times 10^{5}$), the gradual decrease in 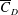 $\overline{C}_{D}$ with increase in
$\overline{C}_{D}$ with increase in 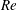 $Re$ is due solely to the increase in suction in a region upstream of the shoulder of the sphere.
$Re$ is due solely to the increase in suction in a region upstream of the shoulder of the sphere.