Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-23T02:31:51.017Z Has data issue: false hasContentIssue false
  • English
  • Français

The hydrodynamics of an active squirming particle inside of a porous container

Published online by Cambridge University Press:  28 May 2021

Kevin J. Marshall Open the ORCID record for Kevin J. Marshall [Opens in a new window]  and
John F. Brady
Kevin J. Marshall*
Affiliation:
Division of Chemistry and Chemical Engineering, California Institute of Technology, Pasadena, CA91125, USA
John F. Brady*
Affiliation:
Division of Chemistry and Chemical Engineering, California Institute of Technology, Pasadena, CA91125, USA
*
†Email addresses for correspondence: [email protected]; [email protected]
†Email addresses for correspondence: [email protected]; [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

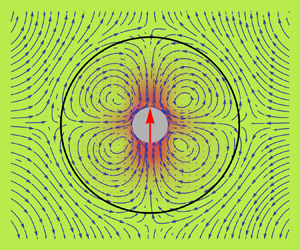
A microswimmer placed inside of a passive lamellar vesicle can hydrodynamically induce directed motion of the vesicle so long as fluid is permitted to pass through the vesicle's surface. With an interest in understanding the underlying theoretical mechanism responsible for this directed motion, we study the low Reynolds number fluid mechanics of a reduced system in which a spherical squirming particle is encapsulated inside of a rigid porous spherical container (membrane). We create a theoretical model for this system and obtain two exact analytical solutions to the Stokes equations which describe the motion of the squirmer and container under porous and non-porous container descriptions. Fluid flow through the container's surface is described using a model similar to Darcy's law where proportionality constants, $R_{\parallel }$ and $R_{\perp }$, parameterize the container's resistance to permeable flow parallel and normal to the container's surface. We numerically simulate trajectories of the squirmer–container system by reformulating the fluid mechanics problem as a coupled set of second kind boundary integral equations (BIEs). This system of BIEs is solved numerically using a Galerkin boundary element discretization on graphics processing units enabled with NVIDIA's Compute Unified Device Architecture. We obtain excellent agreement between the analytical and numerical solutions for the concentric geometry. Trajectories of pusher squirmers show earlier radial spread towards the container's surface, whereas puller squirmers tend to move radially inwards, towards the container's centre. Both the squirmer type (pusher, puller, neutral) and container resistance parameters heavily influence net container motion and early squirmer dynamics.

JFM classification

Low-Reynolds-number flows: Boundary integral methods Biological Fluid Dynamics: Membranes Biological Fluid Dynamics: Micro-organism dynamics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 919 , 25 July 2021 , A31
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Ando, T. & Skolnick, J. 2010 Crowding and hydrodynamic interactions likely dominate in vivo macromolecular motion. Proc. Natl Acad. Sci. 107 (43), 18457–18462.CrossRefGoogle ScholarPubMed
Beavers, G.S. & Joseph, D.D. 1967 Boundary conditions at a naturally permeable wall. J. Fluid Mech. 30 (1), 197–207.CrossRefGoogle Scholar
Blake, J.R. 1971 A spherical envelope approach to ciliary propulsion. J. Fluid Mech. 46 (1), 199–208.CrossRefGoogle Scholar
Bunea, A.I. & Taboryski, R. 2020 Recent advances in microswimmers for biomedical applications. Micromachines 11 (12), 1–24.CrossRefGoogle ScholarPubMed
Ding, Y., Qiu, F., Casadevall i Solvas, X., Chiu, F.W.Y., Nelson, B.J. & De Mello, A. 2016 Microfluidic-based droplet and cell manipulations using artificial bacterial flagella. Micromachines 7 (2), 1–13.CrossRefGoogle ScholarPubMed
Drescher, K., Dunkel, J., Cisneros, L.H., Ganguly, S. & Goldstein, R.E. 2011 Fluid dynamics and noise in bacterial cell–cell and cell–surface scattering. Proc. Natl Acad. Sci. 108 (27), 10940–10945.CrossRefGoogle ScholarPubMed
Dunavant, D.A. 1985 High degree efficient symmetrical Gaussian quadrature rules for the triangle. Intl J. Numer. Meth. Engng 21 (6), 1129–1148.CrossRefGoogle Scholar
Erichsen, S. & Sauter, S.A. 1998 Efficient automatic quadrature in 3-D Galerkin BEM. Comput. Meth. Appl. Mech. Engng 157 (3–4), 215–224.CrossRefGoogle Scholar
Erkoc, P., Yasa, I.C., Ceylan, H., Yasa, O., Alapan, Y. & Sitti, M. 2019 Mobile microrobots for active therapeutic delivery. Adv. Therapeut. 2 (1), 1800064.CrossRefGoogle Scholar
Felfoul, O., et al. 2016 Magneto-aerotactic bacteria deliver drug-containing nanoliposomes to tumour hypoxic regions. Nat. Nanotechnol. 11 (11), 941–947.CrossRefGoogle ScholarPubMed
Feliciano, D., Nixon-Abell, J. & Lippincott-Schwartz, J. 2018 Triggered cell-cell fusion assay for cytoplasmic and organelle intermixing studies. Curr. Protoc. Cell Biol. 81 (1), e61.CrossRefGoogle ScholarPubMed
Goldstein, R.E. & van de Meent, J.W. 2015 A physical perspective on cytoplasmic streaming. Interface Focus 5 (4), 20150030.CrossRefGoogle ScholarPubMed
Happel, J. & Brenner, H. 1983 Low Reynolds Number Hydrodynamics: With Special Applications to Particulate Media, Mechanics of fluids and transport processes, vol. 1. Springer.Google Scholar
Hsiao, G.C. & Wendland, W.L. 2008 Boundary Integral Equations, Applied Mathematical Sciences, vol. 164. Springer.CrossRefGoogle Scholar
Ishikawa, T., Locsei, J.T. & Pedley, T.J. 2008 Development of coherent structures in concentrated suspensions of swimming model micro-organisms. J. Fluid Mech. 615, 401–431.CrossRefGoogle Scholar
Ishikawa, T. & Pedley, T.J. 2007 a Diffusion of swimming model micro-organisms in a semi-dilute suspension. J. Fluid Mech. 588, 437–462.CrossRefGoogle Scholar
Ishikawa, T. & Pedley, T.J. 2007 b The rheology of a semi-dilute suspension of swimming model micro-organisms. J. Fluid Mech. 588, 399–435.CrossRefGoogle Scholar
Ishikawa, T., Simmonds, M.P. & Pedley, T.J. 2006 Hydrodynamic interaction of two swimming model micro-organisms. J. Fluid Mech. 568, 119–160.CrossRefGoogle Scholar
Jackson, L.A., et al. 2020 An mRNA vaccine against SARS-CoV-2 – preliminary report. New Engl. J. Med. 383 (20), 1920–1931.CrossRefGoogle ScholarPubMed
Jones, I.P. 1973 Low Reynolds number flow past a porous spherical shell. Math. Proce. Camb. Phil. Soc. 73 (1), 231–238.CrossRefGoogle Scholar
Karrila, S.J. & Kim, S. 1989 Integral equations of the second kind for Stokes flow: direct solution for physical variables and removal of inherent accuracy limitations. Chem. Engng Commun. 82 (1), 123–161.CrossRefGoogle Scholar
Keh, H.J. & Lu, Y.S. 2005 Creeping motions of a porous spherical shell in a concentric spherical cavity. J. Fluids Struct. 20 (5), 735–747.CrossRefGoogle Scholar
Kim, S. & Karrila, S.J. 2005 Microhydrodynamics: Principles and Selected Applications, Butterworth – Heinemann Series in Chemical Engineering, vol. 1. Dover Publications.Google Scholar
Kress, R. 2014 Linear Integral Equations, Applied Mathematical Sciences, vol. 82. Springer.CrossRefGoogle Scholar
Leal, L. 2007 Advanced Transport Phenomena: Fluid Mechanics and Convective Transport Processes, vol. 9780521849. Cambridge University Press.CrossRefGoogle Scholar
Lebègue, E., Farre, C., Jose, C., Saulnier, J., Lagarde, F., Chevalier, Y., Chaix, C. & Jaffrezic-Renault, N. 2018 Responsive polydiacetylene vesicles for biosensing microorganisms. Sensors 18 (2), 1–16.CrossRefGoogle ScholarPubMed
Li, T., Wan, M. & Mao, C. 2020 Research progress of micro/nanomotors for cancer treatment. ChemPlusChem 85 (12), 2586–2598.CrossRefGoogle ScholarPubMed
Lighthill, M.J. 1952 On the squirming motion of nearly spherical deformable bodies through liquids at very small Reynolds numbers. Commun. Pure Appl. Maths 5 (2), 109–118.CrossRefGoogle Scholar
Marchianò, V., Matos, M., Serrano-Pertierra, E., Gutiérrez, G. & Blanco-López, M.C. 2020 Vesicles as antibiotic carrier: state of art. Intl J. Pharm. 585 (May), 119478.CrossRefGoogle ScholarPubMed
Marshall, K.J. 2018 The hydrodynamics of active particles inside of a porous container and the galerkin boundary element discretization of Stokes flow. PhD thesis, California Institute of Technology, CA.Google Scholar
Medina-Sánchez, M., Xu, H. & Schmidt, O.G. 2018 Micro- and nano-motors: the new generation of drug carriers. Ther. Deliv. 9 (4), 303–316.CrossRefGoogle ScholarPubMed
Mitchell, W.F. 1989 A comparison of adaptive refinement techniques for elliptic problems. ACM Trans. Math. Softw. 15 (4), 326–347.CrossRefGoogle Scholar
Nazockdast, E., Rahimian, A., Zorin, D. & Shelley, M. 2017 A fast platform for simulating semi-flexible fiber suspensions applied to cell mechanics. J. Comput. Phys. 329, 173–209.CrossRefGoogle Scholar
Nganguia, H., Zhu, L., Palaniappan, D. & Pak, O.S. 2020 Squirming in a viscous fluid enclosed by a Brinkman medium. Phys. Rev. E 101 (6), 063105.CrossRefGoogle Scholar
Nguyen, H. 2007 GPU Gems 3, Lab Companion Series, vol. 3. Addison-Wesley.Google Scholar
Park, B.W., Zhuang, J., Yasa, O. & Sitti, M. 2017 Multifunctional bacteria-driven microswimmers for targeted active drug delivery. ACS Nano 11 (9), 8910–8923.CrossRefGoogle ScholarPubMed
Pattni, B.S., Chupin, V.V. & Torchilin, V.P. 2015 New developments in liposomal drug delivery. Chem. Rev. 115 (19), 10938–10966.CrossRefGoogle ScholarPubMed
Phan-Thien, N. & Kim, S. 1994 Microstructures in Elastic Media: Principles and Computational Methods. Oxford University Press.CrossRefGoogle Scholar
Power, H. & Miranda, G. 1987 Second kind integral equation formulation of Stokes’ flows past a particle of arbitrary shape. SIAM J. Appl. Maths 47 (4), 689–698.CrossRefGoogle Scholar
Pozrikidis, C. 1992 Boundary Integral and Singularity Methods for Linearized Viscous Flow, Cambridge Texts in Applied Mathematics. Cambridge University Press.CrossRefGoogle Scholar
Reigh, S.Y. & Lauga, E. 2017 Two-fluid model for locomotion under self-confinement. Phys. Rev. Fluids 2 (9), 093101.CrossRefGoogle Scholar
Reigh, S.Y., Zhu, L., Gallaire, F. & Lauga, E. 2017 Swimming with a cage: low-Reynolds-number locomotion inside a droplet. Soft Matt. 13 (17), 3161–3173.CrossRefGoogle Scholar
Saffman, P.G. 1971 On the boundary condition at the surface of a porous medium. Studi. Appl. Maths 50 (2), 93–101.CrossRefGoogle Scholar
Sauter, S.A. & Schwab, C. 2011 Boundary Element Methods, Springer Series in Computational Mathematics, vol. 39. Springer.CrossRefGoogle Scholar
Schauer, O., Mostaghaci, B., Colin, R., Hürtgen, D., Kraus, D., Sitti, M. & Sourjik, V. 2018 Motility and chemotaxis of bacteria-driven microswimmers fabricated using antigen 43-mediated biotin display. Sci. Rep. 8 (1), 1–11.CrossRefGoogle ScholarPubMed
Shelley, M.J. 2016 The dynamics of microtubule/motor-protein assemblies in biology and physics. Annu. Rev. Fluid Mech. 48 (1), 487–506.CrossRefGoogle Scholar
Singh, A.V., Ansari, M.H.D., Laux, P. & Luch, A. 2019 Micro-nanorobots: important considerations when developing novel drug delivery platforms. Expert Opin. Drug Deliv. 16 (11), 1259–1275.CrossRefGoogle ScholarPubMed
Singh, A.V., Hosseinidoust, Z., Park, B.W., Yasa, O. & Sitti, M. 2017 Microemulsion-based soft bacteria-driven microswimmers for active cargo delivery. ACS. Nano 11 (10), 9759–9769.CrossRefGoogle ScholarPubMed
Spagnolie, S.E. & Lauga, E. 2012 Hydrodynamics of self-propulsion near a boundary: predictions and accuracy of far-field approximations. J. Fluid Mech. 700, 105–147.CrossRefGoogle Scholar
Sprenger, A.R., et al. 2020 Towards an analytical description of active microswimmers in clean and in surfactant-covered drops. Eur. Phys. J. E 43 (58), 1–18.CrossRefGoogle ScholarPubMed
Steinbach, O. 2008 Numerical Approximation Methods for Elliptic Boundary Value Problems: Finite and Boundary Elements. Springer.CrossRefGoogle Scholar
Stone, H.A. & Samuel, A.D.T. 1996 Propulsion of microorganisms by surface distortions. Phys. Rev. Lett. 77 (19), 4102–4104.CrossRefGoogle ScholarPubMed
Takatori, S.C. & Sahu, A. 2020 Active contact forces drive nonequilibrium fluctuations in membrane vesicles. Phys. Rev. Lett. 124 (15), 158102.CrossRefGoogle ScholarPubMed
Trantidou, T., Dekker, L., Polizzi, K., Ces, O. & Elani, Y. 2018 Functionalizing cell-mimetic giant vesicles with encapsulated bacterial biosensors. Interface Focus 8 (5), 20180024.CrossRefGoogle ScholarPubMed
Vutukuri, H.R., Hoore, M., Abaurrea-Velasco, C., van Buren, L., Dutto, A., Auth, T., Fedosov, D.A., Gompper, G. & Vermant, J. 2020 Active particles induce large shape deformations in giant lipid vesicles. Nature 586 (7827), 52–56.CrossRefGoogle ScholarPubMed
Walkington, N. 2000 Quadrature on Simplices of Arbitrary Dimension. Carnegie Mellon University, Department of Mathematical Sciences, Center for Nonlinear Analysis.Google Scholar
Zampogna, G.A. & Gallaire, F. 2020 Effective stress jump across membranes. J. Fluid Mech. 892, A9.CrossRefGoogle Scholar
Zhang, L., Cui, T. & Liu, H. 2009 A set of symmetric quadrature rules on triangles and tetrahedra. J. Comput. Maths 27 (1), 89–96.Google Scholar