Hostname: page-component-78c5997874-fbnjt Total loading time: 0 Render date: 2024-11-17T11:22:58.156Z Has data issue: false hasContentIssue false
  • English
  • Français

Heat transfer in rotating Rayleigh–Bénard convection with rough plates

Published online by Cambridge University Press:  03 October 2017

Pranav Joshi Open the ORCID record for Pranav Joshi [Opens in a new window] ,
Hadi Rajaei ,
Rudie P. J. Kunnen Open the ORCID record for Rudie P. J. Kunnen [Opens in a new window]  and
Herman J. H. Clercx
Pranav Joshi*
Affiliation:
Fluid Dynamics Laboratory, Department of Applied Physics and J. M. Burgers Center for Fluid Dynamics, Eindhoven University of Technology, P.O. Box 513, 5600 MB Eindhoven, The Netherlands Experimental Aerodynamics Division, National Aerospace Laboratories, Bangalore 560017, India
Hadi Rajaei
Affiliation:
Fluid Dynamics Laboratory, Department of Applied Physics and J. M. Burgers Center for Fluid Dynamics, Eindhoven University of Technology, P.O. Box 513, 5600 MB Eindhoven, The Netherlands
Rudie P. J. Kunnen
Affiliation:
Fluid Dynamics Laboratory, Department of Applied Physics and J. M. Burgers Center for Fluid Dynamics, Eindhoven University of Technology, P.O. Box 513, 5600 MB Eindhoven, The Netherlands
Herman J. H. Clercx
Affiliation:
Fluid Dynamics Laboratory, Department of Applied Physics and J. M. Burgers Center for Fluid Dynamics, Eindhoven University of Technology, P.O. Box 513, 5600 MB Eindhoven, The Netherlands
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This experimental study focuses on the effect of horizontal boundaries with pyramid-shaped roughness elements on the heat transfer in rotating Rayleigh–Bénard convection. It is shown that the Ekman pumping mechanism, which is responsible for the heat transfer enhancement under rotation in the case of smooth top and bottom surfaces, is unaffected by the roughness as long as the Ekman layer thickness 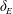$\unicode[STIX]{x1D6FF}_{E}$ is significantly larger than the roughness height $k$. As the rotation rate increases, and thus $\unicode[STIX]{x1D6FF}_{E}$ decreases, the roughness elements penetrate the radially inward flow in the interior of the Ekman boundary layer that feeds the columnar Ekman vortices. This perturbation generates additional thermal disturbances which are found to increase the heat transfer efficiency even further. However, when 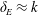$\unicode[STIX]{x1D6FF}_{E}\approx k$, the Ekman boundary layer is strongly perturbed by the roughness elements and the Ekman pumping mechanism is suppressed. The results suggest that the Ekman pumping is re-established for 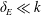$\unicode[STIX]{x1D6FF}_{E}\ll k$ as the faces of the pyramidal roughness elements then act locally as a sloping boundary on which an Ekman layer can be formed.

JFM classification

Geophysical and Geological Flows: Geophysical and Geological Flows Turbulent Flows: Rotating turbulence
Type
Rapids
Information
Journal of Fluid Mechanics , Volume 830 , 10 November 2017 , R3
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Acton, F. S. 1966 Analysis of Straight-Line Data. Dover.Google Scholar
Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81 (2), 503–537.Google Scholar
Aurnou, J. M., Calkins, M. A., Cheng, J. S., Julien, K., King, E. M., Nieves, D., Soderlund, K. M. & Stellmach, S. 2015 Rotating convective turbulence in Earth and planetary cores. Phys. Earth Planet. Inter. 246, 52–71.CrossRefGoogle Scholar
Boubnov, B. M. & Golitsyn, G. S. 1990 Temperature and velocity field regimes of convective motions in a rotating plane fluid layer. J. Fluid Mech. 219, 215–239.CrossRefGoogle Scholar
Chandrasekhar, S. 1961 Hydrodynamic and Hydromagnetic Stability. Oxford University Press.Google Scholar
Cheng, J. S., Stellmach, S., Ribeiro, A., Grannan, A., King, E. M. & Aurnou, J. M. 2015 Laboratory-numerical models of rapidly rotating convection in planetary cores. Geophys. J. Intl 201, 1–17.Google Scholar
Ciliberto, S. & Laroche, C. 1999 Random roughness of boundary increases the turbulent convection scaling exponent. Phys. Rev. Lett. 82 (20), 3998–4001.Google Scholar
Du, Y.-B. & Tong, P. 1998 Enhanced heat transport in turbulent convection over a rough surface. Phys. Rev. Lett. 81 (5), 987–990.CrossRefGoogle Scholar
Du, Y.-B. & Tong, P. 2000 Turbulent thermal convection in a cell with ordered rough boundaries. J. Fluid Mech. 407, 57–84.Google Scholar
Funfschilling, D., Brown, E., Nikolaenko, A. & Ahlers, G. 2005 Heat transport by turbulent Rayleigh–Bénard convection in cylindrical samples with aspect ratio one and larger. J. Fluid Mech. 536, 145–154.Google Scholar
Garrett, C., MacCready, P. & Rhines, P. 1993 Boundary mixing and arrested Ekman layers: rotating stratified flow near a sloping boundary. Annu. Rev. Fluid Mech. 25 (1), 291–323.CrossRefGoogle Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying theory. J. Fluid Mech. 407, 27–56.Google Scholar
Grossmann, S. & Lohse, D. 2001 Thermal convection for large Prandtl numbers. Phys. Rev. Lett. 86 (15), 3316–3319.Google Scholar
Grossmann, S. & Lohse, D. 2002 Prandtl and Rayleigh number dependence of the Reynolds number in turbulent thermal convection. Phys. Rev. E 66, 016305.Google Scholar
Jimenez, J. 2004 Turbulent flows over rough walls. Annu. Rev. Fluid Mech. 36, 173–196.CrossRefGoogle Scholar
Joshi, P., Rajaei, H., Kunnen, R. P. J. & Clercx, H. J. H. 2016 Effect of particle injection on heat transfer in rotating Rayleigh–Bénard convection. Phys. Rev. Fluids 1 (8), 084301.Google Scholar
Julien, K., Knobloch, E., Rubio, A. M. & Vasil, G. M. 2012 Heat transport in low-Rossby-number Rayleigh–Bénard convection. Phys. Rev. Lett. 109 (25), 254503.Google Scholar
King, E. M., Stellmach, S. & Buffett, B. 2013 Scaling behaviour in Rayleigh–Bénard convection with and without rotation. J. Fluid Mech. 717, 449–471.CrossRefGoogle Scholar
King, E. M., Stellmach, S., Noir, J., Hansen, U. & Aurnou, J. M. 2009 Boundary layer control of rotating convection systems. Nature 457 (7227), 301–304.Google Scholar
Kunnen, R. P. J., Clercx, H. J. H. & Geurts, B. J. 2006 Heat flux intensification by vortical flow localization in rotating convection. Phys. Rev. E 74 (5), 056306.Google ScholarPubMed
Kunnen, R. P. J., Clercx, H. J. H. & Geurts, B. J. 2008 Breakdown of large-scale circulation in turbulent rotating convection. Europhys. Lett. 84 (2), 24001.Google Scholar
Kunnen, R. P. J., Clercx, H. J. H. & Geurts, B. J. 2010a Vortex statistics in turbulent rotating convection. Phys. Rev. E 82 (3), 036306.Google ScholarPubMed
Kunnen, R. P. J., Geurts, B. J. & Clercx, H. J. H. 2010b Experimental and numerical investigation of turbulent convection in a rotating cylinder. J. Fluid Mech. 642, 445–476.Google Scholar
Kunnen, R. P. J., Ostilla-Mónico, R., van der Poel, E. P., Verzicco, R. & Lohse, D. 2016 Transition to geostrophic convection: the role of the boundary conditions. J. Fluid Mech. 799, 413–432.Google Scholar
Kunnen, R. P. J., Stevens, R. J. A. M., Overkamp, J., Sun, C., van Heijst, G. J. F. & Clercx, H. J. H. 2011 The role of Stewartson and Ekman layers in turbulent rotating Rayleigh–Bénard convection. J. Fluid Mech. 688, 422–442.Google Scholar
LaCasce, J. H. & Brink, K. H. 2000 Geostrophic turbulence over a slope. J. Phys. Oceanogr. 30 (6), 1305–1324.Google Scholar
Pedlosky, J. 1987 Geophysical Fluid Dynamics, 2nd edn. Springer.Google Scholar
Qiu, X.-L., Xia, K.-Q. & Tong, P. 2005 Experimental study of velocity boundary layer near a rough conducting surface in turbulent natural convection. J. Turbul. 6, N30.Google Scholar
Rajaei, H., Joshi, P., Alards, K. M. J., Kunnen, R. P. J., Toschi, F. & Clercx, H. J. H. 2016 Transitions in turbulent rotating convection: a Lagrangian perspective. Phys. Rev. E 93 (4), 043129.Google Scholar
Rossby, H. T. 1969 A study of Bénard convection with and without rotation. J. Fluid Mech. 36, 309–335.Google Scholar
Shen, Y., Tong, P. & Xia, K.-Q. 1996 Turbulent convection over rough surfaces. Phys. Rev. Lett. 76 (6), 908–911.Google Scholar
Stevens, R. J. A. M., Clercx, H. J. H. & Lohse, D. 2010 Optimal Prandtl number for heat transfer in rotating Rayleigh–Bénard convection. New J. Phys. 12 (7), 075005.Google Scholar
Stevens, R. J. A. M., Clercx, H. J. H. & Lohse, D. 2013a Heat transport and flow structure in rotating Rayleigh–Bénard convection. Eur. J. Mech. (B/Fluids) 40, 41–49.Google Scholar
Stevens, R. J. A. M., van der Poel, E. P., Grossmann, S. & Lohse, D. 2013b The unifying theory of scaling in thermal convection: the updated prefactors. J. Fluid Mech. 730, 295–308.CrossRefGoogle Scholar
Stevens, R. J. A. M., Zhong, J.-Q., Clercx, H. J. H., Ahlers, G. & Lohse, D. 2009 Transitions between turbulent states in rotating Rayleigh–Bénard convection. Phys. Rev. Lett. 103 (2), 024503.Google Scholar
Stringano, G., Pascazio, G. & Verzicco, R. 2006 Turbulent thermal convection over grooved plates. J. Fluid Mech. 557, 307–336.CrossRefGoogle Scholar
Tisserand, J.-C., Creyssels, M., Gasteuil, Y., Pabiou, H., Gibert, M., Castaing, B. & Chilla, F. 2011 Comparison between rough and smooth plates within the same Rayleigh–Bénard cell. Phys. Fluids 23 (1), 015105.Google Scholar
Toppaladoddi, S., Succi, S. & Wettlaufer, J. S. 2017 Roughness as a route to the ultimate regime of thermal convection. Phys. Rev. Lett. 118, 074503.Google Scholar
Vorobieff, P. & Ecke, R. E. 2002 Turbulent rotating convection: an experimental study. J. Fluid Mech. 458, 191–218.Google Scholar
Wagner, S. & Shishkina, O. 2015 Heat flux enhancement by regular surface roughness in turbulent thermal convection. J. Fluid Mech. 763, 109–135.Google Scholar
Wei, P., Chan, T.-S., Ni, R., Zhao, X.-Z. & Xia, K.-Q. 2014 Heat transport properties of plates with smooth and rough surfaces in turbulent thermal convection. J. Fluid Mech. 740, 28–46.CrossRefGoogle Scholar
Weiss, S., Stevens, R. J. A. M., Zhong, J.-Q., Clercx, H. J. H., Lohse, D. & Ahlers, G. 2010 Finite-size effects lead to supercritical bifurcations in turbulent rotating Rayleigh–Bénard convection. Phys. Rev. Lett. 105 (22), 224501.CrossRefGoogle ScholarPubMed
Xi, H.-D., Lam, S. & Xia, K.-Q. 2004 From laminar plumes to organized flows: the onset of large-scale circulation in turbulent thermal convection. J. Fluid Mech. 503, 47–56.Google Scholar
Xie, Y.-C. & Xia, K.-Q.2017 Turbulent thermal convection over rough plates with varying roughness geometries. arXiv:1703.03137.Google Scholar
Zhong, J.-Q. & Ahlers, G. 2010 Heat transport and the large-scale circulation in rotating turbulent Rayleigh–Bénard convection. J. Fluid Mech. 665, 300–333.Google Scholar