Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Julien, Keith
Knobloch, Edgar
Rubio, Antonio M.
and
Vasil, Geoffrey M.
2012.
Heat Transport in Low-Rossby-Number Rayleigh-Bénard Convection.
Physical Review Letters,
Vol. 109,
Issue. 25,
King, Eric M.
and
Aurnou, Jonathan M.
2012.
Thermal evidence for Taylor columns in turbulent rotating Rayleigh-Bénard convection.
Physical Review E,
Vol. 85,
Issue. 1,
Stevens, Richard J. A. M.
Clercx, Herman J. H.
and
Lohse, Detlef
2012.
Breakdown of the large-scale circulation inΓ=1/2rotating Rayleigh-Bénard flow.
Physical Review E,
Vol. 86,
Issue. 5,
Julien, K.
Rubio, A.M.
Grooms, I.
and
Knobloch, E.
2012.
Statistical and physical balances in low Rossby number Rayleigh–Bénard convection.
Geophysical & Astrophysical Fluid Dynamics,
Vol. 106,
Issue. 4-5,
p.
392.
Stevens, Richard J.A.M.
Clercx, Herman J.H.
and
Lohse, Detlef
2013.
Heat transport and flow structure in rotating Rayleigh–Bénard convection.
European Journal of Mechanics - B/Fluids,
Vol. 40,
Issue. ,
p.
41.
Soderlund, K.M.
Heimpel, M.H.
King, E.M.
and
Aurnou, J.M.
2013.
Turbulent models of ice giant internal dynamics: Dynamos, heat transfer, and zonal flows.
Icarus,
Vol. 224,
Issue. 1,
p.
97.
Olson, Peter
2013.
Experimental Dynamos and the Dynamics of Planetary Cores.
Annual Review of Earth and Planetary Sciences,
Vol. 41,
Issue. 1,
p.
153.
King, Eric M.
and
Aurnou, Jonathan M.
2013.
Turbulent convection in liquid metal with and without rotation.
Proceedings of the National Academy of Sciences,
Vol. 110,
Issue. 17,
p.
6688.
King, E. M.
Stellmach, S.
and
Buffett, B.
2013.
Scaling behaviour in Rayleigh–Bénard convection with and without rotation.
Journal of Fluid Mechanics,
Vol. 717,
Issue. ,
p.
449.
Sommer, Tobias
Carpenter, Jeffrey R.
Schmid, Martin
Lueck, Rolf G.
Schurter, Michael
and
Wüest, Alfred
2013.
Interface structure and flux laws in a natural double-diffusive layering.
Journal of Geophysical Research: Oceans,
Vol. 118,
Issue. 11,
p.
6092.
Calkins, Michael A.
Julien, Keith
and
Marti, Philippe
2013.
Three-dimensional quasi-geostrophic convection in the rotating cylindrical annulus with steeply sloping endwalls.
Journal of Fluid Mechanics,
Vol. 732,
Issue. ,
p.
214.
Gastine, T.
Wicht, J.
and
Aurnou, J.M.
2013.
Zonal flow regimes in rotating anelastic spherical shells: An application to giant planets.
Icarus,
Vol. 225,
Issue. 1,
p.
156.
Xia, Ke-Qing
2013.
Current trends and future directions in turbulent thermal convection.
Theoretical and Applied Mechanics Letters,
Vol. 3,
Issue. 5,
p.
052001.
Lebrun, T.
Massol, H.
Chassefière, E.
Davaille, A.
Marcq, E.
Sarda, P.
Leblanc, F.
and
Brandeis, G.
2013.
Thermal evolution of an early magma ocean in interaction with the atmosphere.
Journal of Geophysical Research: Planets,
Vol. 118,
Issue. 6,
p.
1155.
Stelzer, Z.
and
Jackson, A.
2013.
Extracting scaling laws from numerical dynamo models.
Geophysical Journal International,
Vol. 193,
Issue. 3,
p.
1265.
Stellmach, S.
Lischper, M.
Julien, K.
Vasil, G.
Cheng, J. S.
Ribeiro, A.
King, E. M.
and
Aurnou, J. M.
2014.
Approaching the Asymptotic Regime of Rapidly Rotating Convection: Boundary Layers versus Interior Dynamics.
Physical Review Letters,
Vol. 113,
Issue. 25,
Mathis, S.
Neiner, C.
and
Tran Minh, N.
2014.
Impact of rotation on stochastic excitation of gravity and gravito-inertial waves in stars.
Astronomy & Astrophysics,
Vol. 565,
Issue. ,
p.
A47.
Mishra, Subhash C.
Akhtar, Adnan
and
Garg, Anshul
2014.
Numerical Analysis of Rayleigh-Bénard Convection with and Without Volumetric Radiation.
Numerical Heat Transfer, Part A: Applications,
Vol. 65,
Issue. 2,
p.
144.
Horn, Susanne
and
Shishkina, Olga
2014.
Rotating non-Oberbeck–Boussinesq Rayleigh–Bénard convection in water.
Physics of Fluids,
Vol. 26,
Issue. 5,
Soderlund, K. M.
Schmidt, B. E.
Wicht, J.
and
Blankenship, D. D.
2014.
Ocean-driven heating of Europa’s icy shell at low latitudes.
Nature Geoscience,
Vol. 7,
Issue. 1,
p.
16.
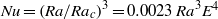 , by considering the stability of the thermal boundary layers, where
, by considering the stability of the thermal boundary layers, where  ,
, 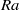 and
and 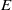 are the Nusselt, Rayleigh and Ekman numbers, respectively, and
are the Nusselt, Rayleigh and Ekman numbers, respectively, and 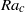 is the critical Rayleigh number for the onset of convection. Furthermore, we use the scaling behaviour of the thermal and Ekman boundary layer thicknesses to quantify the necessary conditions for geostrophic convection:
is the critical Rayleigh number for the onset of convection. Furthermore, we use the scaling behaviour of the thermal and Ekman boundary layer thicknesses to quantify the necessary conditions for geostrophic convection: 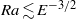 . Interestingly, the predictions of both heat flux and regime transition do not depend on the total height of the fluid layer. We test these scaling arguments with data from laboratory and numerical experiments. Adequate agreement is found between theory and experiment, although there is a paucity of convection data for low
. Interestingly, the predictions of both heat flux and regime transition do not depend on the total height of the fluid layer. We test these scaling arguments with data from laboratory and numerical experiments. Adequate agreement is found between theory and experiment, although there is a paucity of convection data for low 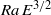 .
.