Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-26T10:36:46.474Z Has data issue: false hasContentIssue false
  • English
  • Français

Global linear instability of the rotating-disk flow investigated through simulations

Published online by Cambridge University Press:  30 January 2015

E. Appelquist ,
P. Schlatter ,
P. H. Alfredsson  and
R. J. Lingwood
E. Appelquist*
Affiliation:
Linné FLOW Centre, KTH Mechanics, SE-100 44 Stockholm, Sweden Swedish e-Science Research Centre (SeRC), SE-100 44 Stockholm, Sweden
P. Schlatter*
Affiliation:
Linné FLOW Centre, KTH Mechanics, SE-100 44 Stockholm, Sweden Swedish e-Science Research Centre (SeRC), SE-100 44 Stockholm, Sweden
P. H. Alfredsson
Affiliation:
Linné FLOW Centre, KTH Mechanics, SE-100 44 Stockholm, Sweden
R. J. Lingwood
Affiliation:
Linné FLOW Centre, KTH Mechanics, SE-100 44 Stockholm, Sweden Institute of Continuing Education, University of Cambridge, Madingley Hall, Madingley, Cambridge CB23 8AQ, UK
*
†Email addresses for correspondence: [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Numerical simulations of the flow developing on the surface of a rotating disk are presented based on the linearized incompressible Navier–Stokes equations. The boundary-layer flow is perturbed by an impulsive disturbance within a linear global framework, and the effect of downstream turbulence is modelled by a damping region further downstream. In addition to the outward-travelling modes, inward-travelling disturbances excited at the radial end of the simulated linear region, $r_{end}$, by the modelled turbulence are included within the simulations, potentially allowing absolute instability to develop. During early times the flow shows traditional convective behaviour, with the total energy slowly decaying in time. However, after the disturbances have reached 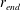$r_{end}$, the energy evolution reaches a turning point and, if the location of 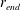$r_{end}$ is at a Reynolds number larger than approximately 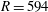$R=594$ (radius non-dimensionalized by 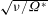$\sqrt{{\it\nu}/{\rm\Omega}^{\ast }}$, where ${\it\nu}$ is the kinematic viscosity and 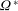${\rm\Omega}^{\ast }$ is the rotation rate of the disk), there will be global temporal growth. The global frequency and mode shape are clearly imposed by the conditions at 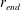$r_{end}$. Our results suggest that the linearized Ginzburg–Landau model by Healey (J. Fluid Mech., vol. 663, 2010, pp. 148–159) captures the (linear) physics of the developing rotating-disk flow, showing that there is linear global instability provided the Reynolds number of 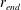$r_{end}$ is sufficiently larger than the critical Reynolds number for the onset of absolute instability.

JFM classification

Instability: Absolute/convective instability Boundary Layers: Boundary layer stability Geophysical and Geological Flows: Rotating flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 765 , 25 February 2015 , pp. 612 - 631
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Appelquist, E.2014 Direct numerical simulations of the rotating-disk boundary-layer flow. Licentiate thesis, Royal Institute of Technology, KTH Mechanics, ISBN: 978-91-7595-202-4.Google Scholar
Bödewadt, U. T. 1940 Die Drehströmung über festem Grund. Z. Angew. Math. Mech. 20, 241–253.CrossRefGoogle Scholar
Chevalier, M., Schlatter, P., Lundbladh, A. & Henningson, D. S.2007 SIMSON – a pseudo-spectral solver for incompressible boundary layer flows. Tech. Rep. Royal Institute of Technology, KTH Mechanics, SE-100 44 Stockholm, Sweden.Google Scholar
Davies, C. & Carpenter, P. W. 2003 Global behaviour corresponding to the absolute instability of the rotating-disc boundary layer. J. Fluid Mech. 486, 287–329.CrossRefGoogle Scholar
Davies, C., Thomas, C. & Carpenter, P. W. 2007 Global stability of the rotating-disk boundary layer. J. Engng Maths 57, 219–236.CrossRefGoogle Scholar
Deville, M. O., Fischer, P. F. & Mund, E. H. 2002 High-Order Methods for Incompressible Fluid Flow. Cambridge University Press.CrossRefGoogle Scholar
Ekman, V. W. 1905 On the influence of the Earth’s rotation on ocean currents. Ark. Mat. Astron. Fys. 2 (11), 1–52.Google Scholar
Fischer, P. F., Lottes, J. W. & Kerkemeier, S. G.2012 Nek5000, http://nek5000.mcs.anl.gov.Google Scholar
Gregory, N., Stuart, J. T. & Walker, W. S. 1955 On the stability of three-dimensional boundary layers with application to the flow due to a rotating disk. Phil. Trans. R. Soc. Lond. A 248, 155–199.Google Scholar
Healey, J. J. 2008 Inviscid axisymmetric absolute instability of swirling jets. J. Fluid Mech. 613, 1–33.CrossRefGoogle Scholar
Healey, J. J. 2010 Model for unstable global modes in the rotating-disk boundary layer. J. Fluid Mech. 663, 148–159.CrossRefGoogle Scholar
Ho, L.-W.1989 A Legendre spectral element method for simulation of incompressible unsteady viscous free-surface flows. PhD thesis, Massachusetts Institute of Technology.Google Scholar
Huerre, P. & Monkewitz, P. A. 1990 Local and global instabilities in spatially developing flows. Annu. Rev. Fluid Mech. 22, 473–537.CrossRefGoogle Scholar
Imayama, S., Alfredsson, P. H. & Lingwood, R. J. 2013 An experimental study of edge effects on rotating-disk transition. J. Fluid Mech. 716, 638–657.CrossRefGoogle Scholar
Imayama, S., Alfredsson, P. H. & Lingwood, R. J. 2014 a On the laminar–turbulent transition of the rotating-disk flow – the role of absolute instability. J. Fluid Mech. 745, 132–163.CrossRefGoogle Scholar
Imayama, S., Lingwood, R. J. & Alfredsson, P. H. 2014b The turbulent rotating-disk boundary layer. Eur. J. Mech. (B/Fluids) 48, 245–253.CrossRefGoogle Scholar
von Kármán, T. 1921 Über laminare und turbulente Reibung. Z. Angew. Math. Mech. 1, 232–252.Google Scholar
Kloker, M. & Konzelmann, U. 1993 Outflow boundary conditions for spatial Navier–Stokes simulations of transition boundary layers. AIAA J. 31, 620–628.CrossRefGoogle Scholar
Lingwood, R. J. 1995a Absolute instability of the boundary layer on a rotating disk. J. Fluid Mech. 299, 17–33.CrossRefGoogle Scholar
Lingwood, R. J.1995b Stability and transition of the boundary layer on a rotating disk. PhD thesis, Cambridge University.Google Scholar
Lingwood, R. J. 1996 An experimental study of absolute instability of the rotating-disk boundary-layer flow. J. Fluid Mech. 314, 373–405.CrossRefGoogle Scholar
Lingwood, R. J. 1997 On the effects of suction and injection on the absolute instability of the rotating-disk boundary layer. Phys. Fluids 9, 1317–1328.CrossRefGoogle Scholar
Littell, H. S. & Eaton, J. K. 1994 Turbulence characteristics of the boundary layer on a rotating disk. J. Fluid Mech. 266, 175–207.CrossRefGoogle Scholar
Mack, L. M.1985 The wave pattern produced by a point source on a rotating disk. AIAA Paper 85-0490.CrossRefGoogle Scholar
Maday, Y. & Patera, A. T. 1989 Spectral element methods for the incompressible Navier–Stokes equations. In State-of-the-Art Surveys on Computational Mechanics (ed. Noor, A. K. & Oden, J. T.), chap. 3, The American Society of Mechanical Engineers.Google Scholar
Malik, M. R. 1986 The neutral curve for stationary disturbances in rotating-disk flow. J. Fluid Mech. 164, 275–287.CrossRefGoogle Scholar
Othman, H. & Corke, T. C. 2006 Experimental investigation of absolute instability of a rotating-disk boundary layer. J. Fluid Mech. 565, 63–94.CrossRefGoogle Scholar
Patera, A. T. 1984 A spectral element method for fluid dynamics: laminar flow in a channel expansion. J. Comput. Phys. 54, 468–488.CrossRefGoogle Scholar
Pier, B. 2003 Finite-amplitude crossflow vortices, secondary instability and transition in the rotating-disk boundary layer. J. Fluid Mech. 487, 315–343.CrossRefGoogle Scholar
Pier, B. 2007 Primary crossflow vortices, secondary absolute instabilities and their control in the rotating-disk boundary layer. J. Engng Maths 57, 237–251.CrossRefGoogle Scholar
Pier, B. 2013 Transition near the edge of a rotating disk. J. Fluid Mech. 737, R1.CrossRefGoogle Scholar
Pier, B. & Huerre, P. 2001 Nonlinear synchronization in open flows. J. Fluids Struct. 15, 471–480.CrossRefGoogle Scholar
Siddiqui, M. E., Mukund, V., Scott, J. & Pier, B. 2013 Experimental characterization of transition region in rotating-disk boundary layer. Phys. Fluids 25, 573–576.CrossRefGoogle Scholar
Thomas, C. & Davies, C. 2010 The effects of mass transfer on the global stability of the rotating-disk boundary layer. J. Fluid Mech. 663, 401–433.CrossRefGoogle Scholar
Thomas, C. & Davies, C. 2013 Global stability of the rotating-disc boundary layer with an axial magnetic field. J. Fluid Mech. 724, 510–526.CrossRefGoogle Scholar
Tufo, H. M. & Fischer, P. F. 2001 Fast parallel direct solvers for coarse grid problems. J. Parallel Distrib. Comput. 61 (2), 151–177.CrossRefGoogle Scholar
Viaud, B., Serre, E. & Chomaz, J.-M. 2008 The elephant mode between two rotating disks. J. Fluid Mech. 598, 451–464.CrossRefGoogle Scholar
Viaud, B., Serre, E. & Chomaz, J.-M. 2011 Transition to turbulence through steep global-modes cascade in an open rotating cavity. J. Fluid Mech. 688, 493–506.CrossRefGoogle Scholar
Wilkinson, S. & Malik, M. R. 1985 Stability experiments in the flow over a rotating disk. AIAA J. 23, 588–595.CrossRefGoogle Scholar