Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Chen, Chen
Duru, Paul
Joseph, Pierre
Geoffroy, Sandrine
and
Prat, Marc
2017.
Control of evaporation by geometry in capillary structures. From confined pillar arrays in a gap radial gradient to phyllotaxy-inspired geometry.
Scientific Reports,
Vol. 7,
Issue. 1,
Cejas, Cesare M.
Hough, Lawrence A.
Frétigny, Christian
and
Dreyfus, Rémi
2018.
Effect of geometry on the dewetting of granular chains by evaporation.
Soft Matter,
Vol. 14,
Issue. 34,
p.
6994.
Qin, Feifei
Del Carro, Luca
Mazloomi Moqaddam, Ali
Kang, Qinjun
Brunschwiler, Thomas
Derome, Dominique
and
Carmeliet, Jan
2019.
Study of non-isothermal liquid evaporation in synthetic micro-pore structures with hybrid lattice Boltzmann model.
Journal of Fluid Mechanics,
Vol. 866,
Issue. ,
p.
33.
Qin, Feifei
Su, Meng
Zhao, Jianlin
Mazloomi Moqaddam, Ali
Carro, Luca Del
Brunschwiler, Thomas
Kang, Qinjun
Song, Yanlin
Derome, Dominique
and
Carmeliet, Jan
2020.
Controlled 3D nanoparticle deposition by drying of colloidal suspension in designed thin micro-porous architectures.
International Journal of Heat and Mass Transfer,
Vol. 158,
Issue. ,
p.
120000.
Lerouge, T.
Maillet, B.
Coutier-Murias, D.
Grande, D.
Le Droumaguet, B.
Pitois, O.
and
Coussot, P.
2020.
Drying of a Compressible Biporous Material.
Physical Review Applied,
Vol. 13,
Issue. 4,
Mahmood, Hafiz Tariq
Tsotsas, Evangelos
and
Kharaghani, Abdolreza
2021.
The Role of Discrete Capillary Rings in Mass Transfer From the Surface of a Drying Capillary Porous Medium.
Transport in Porous Media,
Vol. 140,
Issue. 1,
p.
351.
Kharaghani, Abdolreza
Mahmood, Hafiz Tariq
Wang, Yujing
and
Tsotsas, Evangelos
2021.
Three-dimensional visualization and modeling of capillary liquid rings observed during drying of dense particle packings.
International Journal of Heat and Mass Transfer,
Vol. 177,
Issue. ,
p.
121505.
Qin, Feifei
Zhao, Jianlin
Kang, Qinjun
Derome, Dominique
and
Carmeliet, Jan
2021.
Lattice Boltzmann Modeling of Drying of Porous Media Considering Contact Angle Hysteresis.
Transport in Porous Media,
Vol. 140,
Issue. 1,
p.
395.
Zhao, Xiaozheng
and
Hu, Mingming
2021.
Capillary forces between particles: role of biochar in improving water retention capacity of soil.
Arabian Journal of Geosciences,
Vol. 14,
Issue. 17,
He, Xukun
and
Cheng, Jiangtao
2021.
Evaporation-triggered directional transport of asymmetrically confined droplets.
Journal of Colloid and Interface Science,
Vol. 604,
Issue. ,
p.
550.
Qin, Feifei
Zhao, Jianlin
Kang, Qinjun
Brunschwiler, Thomas
Derome, Dominique
and
Carmeliet, Jan
2021.
Lattice Boltzmann modeling of heat conduction enhancement by colloidal nanoparticle deposition in microporous structures.
Physical Review E,
Vol. 103,
Issue. 2,
Chakraborty, Partha Pratim
Ross, Molly
Bindra, Hitesh
and
Derby, Melanie M.
2022.
Evaporative Drying from Hydrophilic or Hydrophobic Homogeneous Porous Columns: Consequences of Wettability, Porous Structure and Hydraulic Connectivity.
Transport in Porous Media,
Vol. 143,
Issue. 3,
p.
551.
Reis, Paula
Moura, Marcel
Linga, Gaute
Rikvold, Per Arne
Toussaint, Renaud
Flekkøy, Eirik Grude
and
Måløy, Knut Jørgen
2023.
A simplified pore-scale model for slow drainage including film-flow effects.
Advances in Water Resources,
Vol. 182,
Issue. ,
p.
104580.
Cheon, Hyejeong L.
Fyhn, Hursanay
Hansen, Alex
Wilhelmsen, Øivind
and
Sinha, Santanu
2023.
Steady-State Two-Phase Flow of Compressible and Incompressible Fluids in a Capillary Tube of Varying Radius.
Transport in Porous Media,
Vol. 147,
Issue. 1,
p.
15.
Lu, Shun
Zhu, Qingyong
and
Ying, Hao
2024.
The effect of pore size distribution on the fractal evaporative interface in porous media.
Applied Thermal Engineering,
Vol. 246,
Issue. ,
p.
122960.
Chakraborty, Partha Pratim
and
Derby, Melanie M.
2024.
Analysis of Drying Front Propagation and Coupled Heat and Mass Transfer During Evaporation From Additively Manufactured Porous Structures Under a Solar Flux.
ASME Journal of Heat and Mass Transfer,
Vol. 146,
Issue. 2,
Ghiringhelli, Elisa
Marcoux, Manuel
Geoffroy, Sandrine
and
Prat, Marc
2024.
Evaporation in the presence of particles in a model porous medium: impact of corner liquid films.
InterPore Journal,
Vol. 1,
Issue. 1,
p.
ipj260424.
Lan, Tian
Hu, Ran
Wang, Guan‐Xiong
Yang, Zhibing
and
Chen, Yi‐Feng
2024.
Impact of Corner‐Bridge Flow on Capillary Pressure Curve: Insights From Microfluidic Experiments and Pore‐Network Modeling.
Water Resources Research,
Vol. 60,
Issue. 12,
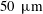 $50~\unicode[STIX]{x03BC}\text{m}$ in both height and diameter, radially arranged as a set of neighbouring spirals and sandwiched between two horizontal flat plates. As drying proceeds, air invades the pore space and elongated liquid films trapped by capillary forces form along the spirals. These films consist of ‘chains’ of liquid bridges connecting neighbouring cylinders. They provide hydraulic connectivity between the central bulk liquid cluster and the external rim of the cylinder pattern, where evaporation takes place during a first constant-evaporation-rate drying stage. The first goal of the present paper is to describe experimentally the phase distribution during drying, notably the evolution of liquid films, which controls the evaporation kinetics (e.g. the depinning of the films from the external rim signals the end of the constant-evaporation-rate period). Then, a viscocapillary model for the drying process is presented. It is based on numerical simulations of a liquid film capillary shape and viscous flow within a film. The model shows a reasonably good agreement with the experimental data. Thus, the present study is a step towards direct modelling of the effect of films on the drying of more complex porous media (e.g. packing of beads) and should be of interest for multiphase flow applications in porous media, involving transport within liquid films.
$50~\unicode[STIX]{x03BC}\text{m}$ in both height and diameter, radially arranged as a set of neighbouring spirals and sandwiched between two horizontal flat plates. As drying proceeds, air invades the pore space and elongated liquid films trapped by capillary forces form along the spirals. These films consist of ‘chains’ of liquid bridges connecting neighbouring cylinders. They provide hydraulic connectivity between the central bulk liquid cluster and the external rim of the cylinder pattern, where evaporation takes place during a first constant-evaporation-rate drying stage. The first goal of the present paper is to describe experimentally the phase distribution during drying, notably the evolution of liquid films, which controls the evaporation kinetics (e.g. the depinning of the films from the external rim signals the end of the constant-evaporation-rate period). Then, a viscocapillary model for the drying process is presented. It is based on numerical simulations of a liquid film capillary shape and viscous flow within a film. The model shows a reasonably good agreement with the experimental data. Thus, the present study is a step towards direct modelling of the effect of films on the drying of more complex porous media (e.g. packing of beads) and should be of interest for multiphase flow applications in porous media, involving transport within liquid films.