Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-26T05:24:08.985Z Has data issue: false hasContentIssue false
  • English
  • Français

Deterministic and stochastic bifurcations in two-dimensional electroconvective flows

Published online by Cambridge University Press:  12 July 2021

Zhe Feng Open the ORCID record for Zhe Feng [Opens in a new window] ,
Mengqi Zhang Open the ORCID record for Mengqi Zhang [Opens in a new window] ,
Pedro A. Vazquez Open the ORCID record for Pedro A. Vazquez [Opens in a new window]  and
Chang Shu Open the ORCID record for Chang Shu [Opens in a new window]
Zhe Feng
Affiliation:
Department of Mechanical Engineering, National University of Singapore, 9 Engineering Drive 1, 117575Republic of Singapore
Mengqi Zhang*
Affiliation:
Department of Mechanical Engineering, National University of Singapore, 9 Engineering Drive 1, 117575Republic of Singapore
Pedro A. Vazquez
Affiliation:
Departamento de Física Aplicada III, Universidad de Sevilla, ESI, Camino de los Descubrimientos s/n, 41092Sevilla, Spain
Chang Shu
Affiliation:
Department of Mechanical Engineering, National University of Singapore, 9 Engineering Drive 1, 117575Republic of Singapore
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

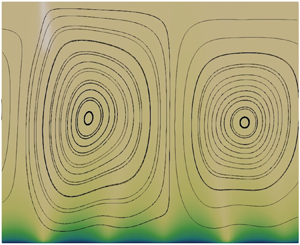
We investigate deterministic and stochastic bifurcations in electroconvecitve flows of a dielectric liquid confined between two parallel plates subjected to a strong unipolar injection by direct numerical simulations. A long-standing discrepancy of linear instability criteria between the experiment and theory exists in this flow. We here test the hypothesis that the discrepancy may be related to the inhomogeneity in ion-exchange membranes used in experiments, contrasted by the homogeneous ion injection assumed in theoretical and numerical analyses. To study this effect, we consider stochastic boundary conditions around linear criticality and first bifurcations in this flow. For a complete presentation of flow bifurcations, deterministic bifurcation analysis (without stochasticity) is first performed to investigate primary bifurcations in this flow by progressively increasing the strength of electric field. Lyapunov spectrum and dimension are calculated and probed to characterise the chaotic motion therein. Our results confirm the high dimensionality of chaos in electroconvective flows and reveal for the first time that its chaos is extensive in a range of finite-sized systems. We then conduct stochastic bifurcation analyses by considering random perturbations in the boundary conditions of charge density and electric potential. Owing to the subcritical nature of electroconvective flows, the linear instability criteria under stochastic boundaries are closer to the experimental values than former theoretical and numerical results (assuming the homogeneous charge injection) for different levels of stochasticity, which confirms the hypothesis aforementioned. Furthermore, stochasticity can also enhance the efficiency of ionic transport.

JFM classification

Complex Fluids: Dielectrics Nonlinear Dynamical Systems: Bifurcation
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 922 , 10 September 2021 , A20
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Ahlers, G., Meyer, C.W. & Cannell, D.S. 1989 Deterministic and stochastic effects near the convective onset. J. Stat. Phys. 54 (5–6), 1121–1131.CrossRefGoogle Scholar
Alj, A., Denat, A., Gosse, J., Gosse, B. & Nakamura, I. 1985 Creation of charge carriers in nonpolar liquids. IEEE Trans. Elec. Insul. 20 (2), 221–231.CrossRefGoogle Scholar
Atten, P., Caputo, J., Malraison, B. & Gagne, Y. 1984 Détermination de dimension d'attracteurs pour différents écoulements. J. Méc. Théor. Appl., 133–156.Google Scholar
Atten, P. & Gosse, J. 1969 Transient of one-carrier injections in polar liquids. J. Chem. Phys. 51 (7), 2804–2811.CrossRefGoogle Scholar
Atten, P. & Lacroix, J. 1978 Electrohydrodynamic stability of liquids subjected to unipolar injection: non linear phenomena. J. Electrost. 5, 439–452.CrossRefGoogle Scholar
Atten, P. & Lacroix, J. 1979 Non-linear hydrodynamic stability of liquids subjected to unipolar injection. J. Méc. 18, 469–510.Google Scholar
Atten, P., Lacroix, J. & Malraison, B. 1980 Chaotic motion in a Coulomb force driven instability: large aspect ratio experiments. Phys. Lett. A 79 (4), 255–258.CrossRefGoogle Scholar
Atten, P. & Moreau, R. 1972 Stabilité électrohydrodynamique des liquides isolants soumis à une injection unipolaire. J. Méc. 11 (3), 471–521.Google Scholar
Cadou, J.M., Potier-Ferry, M. & Cochelin, B. 2006 A numerical method for the computation of bifurcation points in fluid mechanics. Eur. J. Mech. (B/Fluids) 25 (2), 234–254.CrossRefGoogle Scholar
Castellanos, A. 1990 Injection induced instabilities and chaos in electrohydrodynamics. J. Phys.: Condens. Matter 2 (S), SA499–SA503.Google Scholar
Castellanos, A. 1998 Electrohydrodynamics, vol. 380. Springer Science & Business Media.CrossRefGoogle Scholar
Castellanos, A. & Agrait, N. 1992 Unipolar injection induced instabilities in plane parallel flows. IEEE Trans. Ind. Applics. 28 (3), 513–519.CrossRefGoogle Scholar
Castellanos, A., Atten, P. & Perez, A. 1987 Finite-amplitude electroconvection in liquids in the case of weak unipolar injection. Physico-Chem. Hydrodyn. 9 (3–4), 443–452.Google Scholar
Castellanos, A., Pérez, A. & Atten, P. 1989 Charge diffusion versus Coulomb repulsion in finite amplitude electroconvection. In Conference Record of the IEEE Industry Applications Society Annual Meeting, pp. 2112–2117. IEEE.Google Scholar
Chicón, R., Pérez, A. & Castellanos, A. 2001 Lyapunov exponents of time series in finite amplitude electroconvection. In 2001 Annual Report Conference on Electrical Insulation and Dielectric Phenomena (Cat. No. 01CH37225), pp. 520–523. IEEE.Google Scholar
Delbende, I. & Chomaz, J.-M. 1998 Nonlinear convective/absolute instabilities in parallel two-dimensional wakes. Phys. Fluids 10 (11), 2724–2736.CrossRefGoogle Scholar
Eckmann, J.P. & Ruelle, D. 1985 Ergodic theory of chaos and strange attractors. Rev. Mod. Phys. 57, 617–656.CrossRefGoogle Scholar
Edwards, W., Tuckerman, L.S., Friesner, R.A. & Sorensen, D. 1994 Krylov methods for the incompressible Navier–Stokes equations. J. Comput. Phys. 110 (1), 82–102.CrossRefGoogle Scholar
Egolf, D.A. & Greenside, H.S. 1994 Relation between fractal dimension and spatial correlation length for extensive chaos. Nature 369 (6476), 129–131.CrossRefGoogle Scholar
Egolf, D.A., Melnikov, I.V., Pesch, W. & Ecke, R.E. 2000 Mechanisms of extensive spatiotemporal chaos in Rayleigh–Bénard convection. Nature 404 (6779), 733–736.CrossRefGoogle ScholarPubMed
Farmer, J.D., Ott, E. & Yorke, J.A. 1983 The dimension of chaotic attractors. Physica D 7 (1–3), 153–180.CrossRefGoogle Scholar
Félici, N. 1971 DC conduction in liquid dielectrics. Part II: electrohydrodynamic phenomena. Direct Curr. Power Electron. 2, 147–165.Google Scholar
Fischer, P.F., Lottes, J.W. & Kerkemeier, S.G. 2008 nek5000 web page. Available at: http://nek5000.mcs.anl.gov.Google Scholar
Galama, A., Hoog, N. & Yntema, D. 2016 Method for determining ion exchange membrane resistance for electrodialysis systems. Desalination 380, 1–11.CrossRefGoogle Scholar
Grassberger, P. & Procaccia, I. 1983 Characterization of strange attractors. Phys. Rev. Lett. 50 (5), 346–349.CrossRefGoogle Scholar
Henderson, R.D. & Barkley, D. 1996 Secondary instability in the wake of a circular cylinder. Phys. Fluids 8 (6), 1683–1685.CrossRefGoogle Scholar
Ibanez, R., Stamatialis, D. & Wessling, M. 2004 Role of membrane surface in concentration polarization at cation exchange membranes. J. Membr. Sci. 239 (1), 119–128.CrossRefGoogle Scholar
Jalaal, M., Khorshidi, B. & Esmaeilzadeh, E. 2013 Electrohydrodynamic (EHD) mixing of two miscible dielectric liquids. Chem. Engng J. 219, 118–123.CrossRefGoogle Scholar
Kamcev, J., Paul, D.R. & Freeman, B.D. 2017 Effect of fixed charge group concentration on equilibrium ion sorption in ion exchange membranes. J. Mater. Chem. 5 (9), 4638–4650.CrossRefGoogle Scholar
Kantz, H. & Schreiber, T. 2004 Nonlinear Time Series Analysis, vol. 7. Cambridge University Press.Google Scholar
Kaplan, J.L. & Yorke, J.A. 1979 Chaotic behavior of multidimensional difference equations. In Functional Differential Equations and Approximation of Fixed Points (ed. H.O. Peitgen & H.O. Walther), pp. 204–227. Springer.CrossRefGoogle Scholar
Kelly, R. & Pal, D. 1978 Thermal convection with spatially periodic boundary conditions: resonant wavelength excitation. J. Fluid Mech. 86 (3), 433–456.CrossRefGoogle Scholar
Lacroix, J., Atten, P. & Hopfinger, E. 1975 Electro-convection in a dielectric liquid layer subjected to unipolar injection. J. Fluid Mech. 69 (3), 539–563.CrossRefGoogle Scholar
Lehoucq, R.B., Sorensen, D.C. & Yang, C. 1998 ARPACK Users’ Guide: Solution of Large-Scale Eigenvalue Problems with Implicitly Restarted Arnoldi Methods, vol. 6. SIAM.CrossRefGoogle Scholar
Lestandi, L., Bhaumik, S., Avatar, G., Azaiez, M. & Sengupta, T.K. 2018 Multiple Hopf bifurcations and flow dynamics inside a 2D singular lid driven cavity. Comput. Fluids 166, 86–103.CrossRefGoogle Scholar
Levanger, R., Xu, M., Cyranka, J., Schatz, M., Mischaikow, K. & Paul, M. 2019 Correlations between the leading Lyapunov vector and pattern defects for chaotic Rayleigh–Bénard convection. Chaos 29 (5), 053103.CrossRefGoogle ScholarPubMed
Li, F., Wang, B.-F., Wan, Z.-H., Wu, J. & Zhang, M. 2019 Absolute and convective instabilities in electrohydrodynamic flow subjected to a poiseuille flow: a linear analysis. J. Fluid Mech. 862, 816–844.CrossRefGoogle Scholar
Luis, P. 2018 Introduction. In Fundamental Modelling of Membrane Systems (ed. P. Luis), chap. 1, pp. 1–23. Elsevier.CrossRefGoogle Scholar
Malraison, B. & Atten, P. 1982 Chaotic behavior of instability due to unipolar ion injection in a dielectric liquid. Phys. Rev. Lett. 49 (10), 723–726.CrossRefGoogle Scholar
Malraison, B., Atten, P., Berge, P. & Dubois, M. 1983 Dimension of strange attractors: an experimental determination for the chaotic regime of two convective systems. J. Phys. Lett. 44 (22), 897–902.CrossRefGoogle Scholar
McCluskey, F., Atten, P. & Perez, A. 1991 Heat transfer enhancement by electroconvection resulting from an injected space charge between parallel plates. Intl J. Heat Mass Transfer 34 (9), 2237–2250.CrossRefGoogle Scholar
Moleón, J. & Moya, A. 2009 Transient electrical response of ion-exchange membranes with fixed-charge due to ion adsorption. A network simulation approach. J. Electroanalyt. Chem. 633 (2), 306–313.CrossRefGoogle Scholar
Patera, A.T. 1984 A spectral element method for fluid dynamics: laminar flow in a channel expansion. J. Comput. Phys. 54 (3), 468–488.CrossRefGoogle Scholar
Paul, M., Chiam, K.-H., Cross, M., Fischer, P. & Greenside, H. 2003 Pattern formation and dynamics in Rayleigh–Bénard convection: numerical simulations of experimentally realistic geometries. Physica D 184 (1–4), 114–126.CrossRefGoogle Scholar
Paul, M., Einarsson, M., Fischer, P. & Cross, M. 2007 Extensive chaos in Rayleigh–Bénard convection. Phys. Rev. E 75 (4), 045203.CrossRefGoogle ScholarPubMed
Pérez, A., Vázquez, P., Wu, J. & Traoré, P. 2014 Electrohydrodynamic linear stability analysis of dielectric liquids subjected to unipolar injection in a rectangular enclosure with rigid sidewalls. J. Fluid Mech. 758, 586–602.CrossRefGoogle Scholar
Pérez, A.T. & Castellanos, A. 1989 Role of charge diffusion in finite-amplitude electroconvection. Phys. Rev. A 40, 5844–5855.CrossRefGoogle ScholarPubMed
Ruelle, D. 1982 Large volume limit of the distribution of characteristic exponents in turbulence. Commun. Math. Phys. 87 (2), 287–302.CrossRefGoogle Scholar
Sakievich, P., Peet, Y. & Adrian, R. 2016 Large-scale thermal motions of turbulent Rayleigh–Bénard convection in a wide aspect-ratio cylindrical domain. Intl J. Heat Fluid Flow 61, 183–196.CrossRefGoogle Scholar
Schneider, J. & Watson, P. 1970 Electrohydrodynamic stability of space-charge-limited currents in dielectric liquids. I. Theoretical study. Phys. Fluids 13 (8), 1948–1954.CrossRefGoogle Scholar
Selvey, C. & Reiss, H. 1985 Ion transport in inhomogeneous ion exchange membranes. J. Membr. Sci. 23 (1), 11–27.CrossRefGoogle Scholar
Seyed-Yagoobi, J. 2005 Electrohydrodynamic pumping of dielectric liquids. J. Electrostat. 63 (6–10), 861–869.CrossRefGoogle Scholar
Shin, K. & Hammond, J. 1998 The instantaneous lyapunov exponent and its application to chaotic dynamical systems. J. Sound Vib. 218 (3), 389–403.CrossRefGoogle Scholar
Sokirko, A.V., Manzanares, J.A. & Pellicer, J. 1994 The permselectivity of membrane systems with an inhomogeneous distribution of fixed charge groups. J. Colloid Interface Sci. 168 (1), 32–39.CrossRefGoogle Scholar
Takagi, R., Vaselbehagh, M. & Matsuyama, H. 2014 Theoretical study of the permselectivity of an anion exchange membrane in electrodialysis. J. Membr. Sci. 470, 486–493.CrossRefGoogle Scholar
Tanaka, Y. 2015 Ion Exchange Membranes: Fundamentals and Applications. Elsevier Science.CrossRefGoogle Scholar
Theofilis, V. 2011 Global linear instability. Annu. Rev. Fluid Mech. 43 (1), 319–352.CrossRefGoogle Scholar
Traoré, P. & Pérez, A. 2012 Two-dimensional numerical analysis of electroconvection in a dielectric liquid subjected to strong unipolar injection. Phys. Fluids 24 (3), 037102.CrossRefGoogle Scholar
Tuckerman, L.S. & Barkley, D. 1999 Bifurcation Analysis of Time Steppers. IMA Volumes in Mathematics and its Applications, pp. 453–466. Springer.Google Scholar
Urata, K. 1987 Low dimensional chaos in Boussinesq convection. Fluid Dyn. Res. 1 (3–4), 257–282.CrossRefGoogle Scholar
Venturi, D., Choi, M. & Karniadakis, G. 2012 Supercritical quasi-conduction states in stochastic Rayleigh–Bénard convection. Intl J. Heat Mass Transfer 55 (13–14), 3732–3743.CrossRefGoogle Scholar
Venturi, D., Wan, X. & Karniadakis, G.E. 2008 Stochastic low-dimensional modelling of a random laminar wake past a circular cylinder. J. Fluid Mech. 606, 339–367.CrossRefGoogle Scholar
Venturi, D., Wan, X. & Karniadakis, G.E. 2010 Stochastic bifurcation analysis of Rayleigh–Bénard convection. J. Fluid Mech. 650, 391–413.CrossRefGoogle Scholar
Vrijenhoek, E.M., Hong, S. & Elimelech, M. 2001 Influence of membrane surface properties on initial rate of colloidal fouling of reverse osmosis and nanofiltration membranes. J. Membr. Sci. 188 (1), 115–128.CrossRefGoogle Scholar
Wang, B.-F. & Sheu, T.W.-H. 2016 Numerical investigation of electrohydrodynamic instability and bifurcation in a dielectric liquid subjected to unipolar injection. Comput. Fluids 136, 1–10.CrossRefGoogle Scholar
Watson, P., Schneider, J. & Till, H. 1970 Electrohydrodynamic stability of space-charge-limited currents in dielectric liquids. II. Experimental study. Phys. Fluids 13 (8), 1955–1961.CrossRefGoogle Scholar
Wolf, A., Swift, J.B., Swinney, H.L. & Vastano, J.A. 1985 Determining lyapunov exponents from a time series. Physica D 16 (3), 285–317.CrossRefGoogle Scholar
Wu, J., Traoré, P., Pérez, A.T. & Vázquez, P.A. 2015 On two-dimensional finite amplitude electro-convection in a dielectric liquid induced by a strong unipolar injection. J. Electrostat. 74, 85–95.CrossRefGoogle Scholar
Zabolotsky, V.I. & Nikonenko, V.V. 1993 Effect of structural membrane inhomogeneity on transport properties. J. Membr. Sci. 79 (2–3), 181–198.CrossRefGoogle Scholar
Zhang, M. 2016 Weakly nonlinear stability analysis of subcritical electrohydrodynamic flow subject to strong unipolar injection. J. Fluid Mech. 792, 328–363.CrossRefGoogle Scholar
Zhang, M., Martinelli, F., Wu, J., Schmid, P.J. & Quadrio, M. 2015 Modal and non-modal stability analysis of electrohydrodynamic flow with and without cross-flow. J. Fluid Mech. 770, 319–349.CrossRefGoogle Scholar