Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Pähtz, Thomas
and
Durán, Orencio
2017.
Fluid forces or impacts: What governs the entrainment of soil particles in sediment transport mediated by a Newtonian fluid?.
Physical Review Fluids,
Vol. 2,
Issue. 7,
Chauchat, Julien
Cheng, Zhen
Nagel, Tim
Bonamy, Cyrille
and
Hsu, Tian-Jian
2017.
SedFoam-2.0: a 3-D two-phase flow numerical model for sediment transport.
Geoscientific Model Development,
Vol. 10,
Issue. 12,
p.
4367.
Frey, Philippe
Maurin, Raphaël
Morchid-Alaoui, Lamia
Gupta, Shashank
Chauchat, Julien
Radjai, F.
Nezamabadi, S.
Luding, S.
and
Delenne, J.Y.
2017.
Investigation of vertical size segregation in bedload sediment transport with a coupled fluid-discrete element model.
EPJ Web of Conferences,
Vol. 140,
Issue. ,
p.
09025.
Ferdowsi, Behrooz
Ortiz, Carlos P.
Houssais, Morgane
and
Jerolmack, Douglas J.
2017.
River-bed armouring as a granular segregation phenomenon.
Nature Communications,
Vol. 8,
Issue. 1,
Maurin, Raphael
Chauchat, Julien
and
Frey, Philippe
2018.
Revisiting slope influence in turbulent bedload transport: consequences for vertical flow structure and transport rate scaling.
Journal of Fluid Mechanics,
Vol. 839,
Issue. ,
p.
135.
Ni, Wei‐Jay
and
Capart, Hervé
2018.
Stresses and Drag in Turbulent Bed Load From Refractive Index‐Matched Experiments.
Geophysical Research Letters,
Vol. 45,
Issue. 14,
p.
7000.
Frey, Philippe
Chassagne, Rémi
Maurin, Raphaël
Chauchat, Julien
Paquier, A.
and
Rivière, N.
2018.
Vertical grain size sorting in bedload transport on steep slopes with a coupled fluid-discrete element model.
E3S Web of Conferences,
Vol. 40,
Issue. ,
p.
04013.
Lee, Dylan B.
and
Jerolmack, Douglas
2018.
Determining the scales of collective entrainment in collision-driven bed load.
Earth Surface Dynamics,
Vol. 6,
Issue. 4,
p.
1089.
Elghannay, Husam
and
Tafti, Danesh
2018.
LES-DEM simulations of sediment transport.
International Journal of Sediment Research,
Vol. 33,
Issue. 2,
p.
137.
Lafaye de Micheaux, Hugo
Ducottet, Christophe
and
Frey, Philippe
2018.
Multi-model particle filter-based tracking with switching dynamical state to study bedload transport.
Machine Vision and Applications,
Vol. 29,
Issue. 5,
p.
735.
Gonzalez-Ondina, Jose M.
Fraccarollo, Luigi
and
Liu, Philip L.-F.
2018.
Two-level, two-phase model for intense, turbulent sediment transport.
Journal of Fluid Mechanics,
Vol. 839,
Issue. ,
p.
198.
Yang, Jiecheng
Low, Ying Min
Lee, Cheng-Hsien
and
Chiew, Yee-Meng
2018.
Numerical simulation of scour around a submarine pipeline using computational fluid dynamics and discrete element method.
Applied Mathematical Modelling,
Vol. 55,
Issue. ,
p.
400.
Ouillon, Sylvain
2018.
Why and How Do We Study Sediment Transport? Focus on Coastal Zones and Ongoing Methods.
Water,
Vol. 10,
Issue. 4,
p.
390.
Pähtz, Thomas
and
Durán, Orencio
2018.
Universal friction law at granular solid-gas transition explains scaling of sediment transport load with excess fluid shear stress.
Physical Review Fluids,
Vol. 3,
Issue. 10,
Chauchat, Julien
2018.
A comprehensive two-phase flow model for unidirectional sheet-flows.
Journal of Hydraulic Research,
Vol. 56,
Issue. 1,
p.
15.
Pähtz, Thomas
and
Durán, Orencio
2018.
The Cessation Threshold of Nonsuspended Sediment Transport Across Aeolian and Fluvial Environments.
Journal of Geophysical Research: Earth Surface,
Vol. 123,
Issue. 8,
p.
1638.
Jerolmack, Douglas J.
and
Daniels, Karen E.
2019.
Viewing Earth’s surface as a soft-matter landscape.
Nature Reviews Physics,
Vol. 1,
Issue. 12,
p.
716.
Gu, Yile
Ozel, Ali
Kolehmainen, Jari
and
Sundaresan, Sankaran
2019.
Computationally generated constitutive models for particle phase rheology in gas-fluidized suspensions.
Journal of Fluid Mechanics,
Vol. 860,
Issue. ,
p.
318.
ADONG, Feddy
Bennis, Anne-Claire
and
Mouazé, Dominique
2019.
Numerical investigation of bore hole filling volume in a coastal area.
Applied Ocean Research,
Vol. 83,
Issue. ,
p.
112.
Albaba, Adel
Schwarz, Massimiliano
Wendeler, Corinna
Loup, Bernard
and
Dorren, Luuk
2019.
Numerical modeling using an elastoplastic-adhesive discrete element code for simulating hillslope debris flows and calibration against field experiments .
Natural Hazards and Earth System Sciences,
Vol. 19,
Issue. 11,
p.
2339.
 $\unicode[STIX]{x1D707}(I)$ rheology and exhibit a collapse of both the shear to normal stress ratio and the solid volume fraction over a wide range of inertial numbers. Contrary to expectations, the effect of the interstitial fluid on the granular rheology is shown to be negligible, supporting recent work suggesting the absence of a clear transition between the free-fall and turbulent regimes. In addition, data collapse is observed up to unexpectedly high inertial numbers
$\unicode[STIX]{x1D707}(I)$ rheology and exhibit a collapse of both the shear to normal stress ratio and the solid volume fraction over a wide range of inertial numbers. Contrary to expectations, the effect of the interstitial fluid on the granular rheology is shown to be negligible, supporting recent work suggesting the absence of a clear transition between the free-fall and turbulent regimes. In addition, data collapse is observed up to unexpectedly high inertial numbers 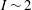 $I\sim 2$, challenging the existing conceptions and parametrisation of the
$I\sim 2$, challenging the existing conceptions and parametrisation of the 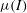 $\unicode[STIX]{x1D707}(I)$ rheology. Focusing upon bedload transport modelling, the results are pragmatically analysed in the
$\unicode[STIX]{x1D707}(I)$ rheology. Focusing upon bedload transport modelling, the results are pragmatically analysed in the 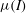 $\unicode[STIX]{x1D707}(I)$ framework in order to propose a granular rheology for bedload transport. The proposed rheology is tested using a 1D volume-averaged two-phase continuous model, and is shown to accurately reproduce the dense granular flow profiles and the sediment transport rate over a wide range of Shields numbers. The present contribution represents a step in the upscaling process from particle-scale simulations towards large-scale applications involving complex flow geometry.
$\unicode[STIX]{x1D707}(I)$ framework in order to propose a granular rheology for bedload transport. The proposed rheology is tested using a 1D volume-averaged two-phase continuous model, and is shown to accurately reproduce the dense granular flow profiles and the sediment transport rate over a wide range of Shields numbers. The present contribution represents a step in the upscaling process from particle-scale simulations towards large-scale applications involving complex flow geometry.