Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Renard, Nicolas
and
Deck, Sébastien
2015.
On the scale-dependent turbulent convection velocity in a spatially developing flat plate turbulent boundary layer at Reynolds number .
Journal of Fluid Mechanics,
Vol. 775,
Issue. ,
p.
105.
Hwang, Yongyun
2015.
Statistical structure of self-sustaining attached eddies in turbulent channel flow.
Journal of Fluid Mechanics,
Vol. 767,
Issue. ,
p.
254.
Keylock, Christopher J.
2015.
Flow resistance in natural, turbulent channel flows: The need for a fluvial fluid mechanics.
Water Resources Research,
Vol. 51,
Issue. 6,
p.
4374.
Duvvuri, Subrahmanyam
and
McKeon, Beverley J.
2015.
Triadic scale interactions in a turbulent boundary layer.
Journal of Fluid Mechanics,
Vol. 767,
Issue. ,
Baars, W. J.
Talluru, K. M.
Hutchins, N.
and
Marusic, I.
2015.
Wavelet analysis of wall turbulence to study large-scale modulation of small scales.
Experiments in Fluids,
Vol. 56,
Issue. 10,
Lee, Jin
Ahn, Junsun
and
Sung, Hyung Jin
2015.
Comparison of large- and very-large-scale motions in turbulent pipe and channel flows.
Physics of Fluids,
Vol. 27,
Issue. 2,
de Silva, C. M.
Marusic, I.
Woodcock, J. D.
and
Meneveau, C.
2015.
Scaling of second- and higher-order structure functions in turbulent boundary layers.
Journal of Fluid Mechanics,
Vol. 769,
Issue. ,
p.
654.
Hwang, Jinyul
Lee, Jin
Sung, Hyung Jin
and
Zaki, Tamer A.
2016.
Inner–outer interactions of large-scale structures in turbulent channel flow.
Journal of Fluid Mechanics,
Vol. 790,
Issue. ,
p.
128.
Agostini, Lionel
Leschziner, Michael
and
Gaitonde, Datta
2016.
Skewness-induced asymmetric modulation of small-scale turbulence by large-scale structures.
Physics of Fluids,
Vol. 28,
Issue. 1,
Squire, D. T.
Baars, W. J.
Hutchins, N.
and
Marusic, I.
2016.
Inner–outer interactions in rough-wall turbulence.
Journal of Turbulence,
Vol. 17,
Issue. 12,
p.
1159.
Blackman, Karin
and
Perret, Laurent
2016.
Non-linear interactions in a boundary layer developing over an array of cubes using stochastic estimation.
Physics of Fluids,
Vol. 28,
Issue. 9,
Hwang, Jinyul
Lee, Jin
and
Sung, Hyung Jin
2016.
Influence of large-scale accelerating motions on turbulent pipe and channel flows.
Journal of Fluid Mechanics,
Vol. 804,
Issue. ,
p.
420.
Agostini, Lionel
and
Leschziner, Michael
2016.
On the validity of the quasi-steady-turbulence hypothesis in representing the effects of large scales on small scales in boundary layers.
Physics of Fluids,
Vol. 28,
Issue. 4,
Anderson, William
2016.
Amplitude modulation of streamwise velocity fluctuations in the roughness sublayer: evidence from large-eddy simulations.
Journal of Fluid Mechanics,
Vol. 789,
Issue. ,
p.
567.
Squire, D. T.
Morrill-Winter, C.
Hutchins, N.
Marusic, I.
Schultz, M. P.
and
Klewicki, J. C.
2016.
Smooth- and rough-wall boundary layer structure from high spatial range particle image velocimetry.
Physical Review Fluids,
Vol. 1,
Issue. 6,
Tang, Zhanqi
and
Wu, Yanhua
2016.
Tomographic PIV measurements of turbulent boundary layer perturbed by a dynamic cylinder roughness element.
Tang, Zhanqi
Jiang, Nan
Zheng, Xiaobo
and
Wu, Yanhua
2016.
Bursting process of large- and small-scale structures in turbulent boundary layer perturbed by a cylinder roughness element.
Experiments in Fluids,
Vol. 57,
Issue. 5,
Tang, Yifeng
and
Akhavan, Rayhaneh
2016.
Computations of equilibrium and non-equilibrium turbulent channel flows using a nested-LES approach.
Journal of Fluid Mechanics,
Vol. 793,
Issue. ,
p.
709.
Krug, Dominik
Yang, Xiang I. A.
de Silva, Charitha M.
Ostilla-Mónico, Rodolfo
Verzicco, Roberto
Marusic, Ivan
and
Lohse, Detlef
2017.
Statistics of turbulence in the energy-containing range of Taylor–Couette compared to canonical wall-bounded flows.
Journal of Fluid Mechanics,
Vol. 830,
Issue. ,
p.
797.
Yang, X. I. A.
Baidya, R.
Johnson, P.
Marusic, I.
and
Meneveau, C.
2017.
Structure function tensor scaling in the logarithmic region derived from the attached eddy model of wall-bounded turbulent flows.
Physical Review Fluids,
Vol. 2,
Issue. 6,
 $v$ and
$v$ and 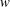 $w$) and the instantaneous Reynolds shear stress (
$w$) and the instantaneous Reynolds shear stress ( $-{uw}$) are modulated in a very similar manner to that previously noted for the streamwise fluctuations (
$-{uw}$) are modulated in a very similar manner to that previously noted for the streamwise fluctuations ( $u$). The envelope of the small scale fluctuations for all velocity components is well described by the large-scale component of the
$u$). The envelope of the small scale fluctuations for all velocity components is well described by the large-scale component of the  $u$ fluctuation. These results also confirm the conditional existence of roll modes associated with the very large-scale or ‘superstructure’ motions.
$u$ fluctuation. These results also confirm the conditional existence of roll modes associated with the very large-scale or ‘superstructure’ motions.