Published online by Cambridge University Press: 29 November 2019
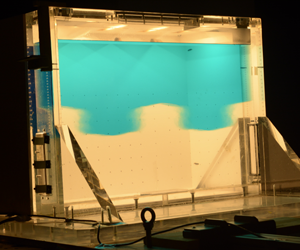
Experiments of a turbulent mixing zone created by the Faraday instability at the statically stable interface between salt and fresh water are presented. The two-layer system, contained in a cuboidal tank of large dimensions, is accelerated vertically and periodically at various frequencies and amplitudes for three different density contrasts. We have developed a linear approach accounting for the full inhomogeneous and viscous problem, that is applied to a linear piecewise background density profile, and recovers the limiting cases of interface and homogeneous turbulence with a fully developed mixing layer. At onset, the wavelength of the most amplified modes and the corresponding Floquet exponent of the interface both verify our predictions. The dynamics is rather different when the instability is triggered from a sharp or diffuse interface: in the latter case, a change of characteristic wavelengths can be observed experimentally and explained by the theory. In the turbulent regime, the time evolution of the mixing zone size  $L(t)$ for various experimental configurations compares well with confined direct numerical simulations. For some initial conditions, a short harmonic response of the instability is observed before the usual subharmonic one. Finally, the ultimate size of the mixing layer
$L(t)$ for various experimental configurations compares well with confined direct numerical simulations. For some initial conditions, a short harmonic response of the instability is observed before the usual subharmonic one. Finally, the ultimate size of the mixing layer  $L_{end}$, measured with a probe after the saturation of the instability and end of the forcing, is in excellent agreement with the recent theoretical prediction
$L_{end}$, measured with a probe after the saturation of the instability and end of the forcing, is in excellent agreement with the recent theoretical prediction  $L_{sat}=2{\mathcal{A}}g_{0}(2F+4)/\unicode[STIX]{x1D714}^{2}$, where
$L_{sat}=2{\mathcal{A}}g_{0}(2F+4)/\unicode[STIX]{x1D714}^{2}$, where  $g_{0}$ is the gravitational acceleration,
$g_{0}$ is the gravitational acceleration,  ${\mathcal{A}}$ the Atwood number,
${\mathcal{A}}$ the Atwood number,  $\unicode[STIX]{x1D714}/2\unicode[STIX]{x03C0}$ the frequency and
$\unicode[STIX]{x1D714}/2\unicode[STIX]{x03C0}$ the frequency and  $F$ the acceleration ratio.
$F$ the acceleration ratio.