Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-19T02:07:29.676Z Has data issue: false hasContentIssue false
  • English
  • Français

Transient effects in the translation of bubbles insonated with acoustic pulses of finite duration

Published online by Cambridge University Press:  12 December 2017

Elena Igualada-Villodre ,
Ana Medina-Palomo ,
Patricia Vega-Martínez  and
Javier Rodríguez-Rodríguez Open the ORCID record for Javier Rodríguez-Rodríguez [Opens in a new window]
Elena Igualada-Villodre
Affiliation:
Fluid Mechanics Group, Carlos III University of Madrid, Leganes, Madrid, 28911, Spain
Ana Medina-Palomo
Affiliation:
Fluid Mechanics Group, Carlos III University of Madrid, Leganes, Madrid, 28911, Spain
Patricia Vega-Martínez
Affiliation:
Fluid Mechanics Group, Carlos III University of Madrid, Leganes, Madrid, 28911, Spain
Javier Rodríguez-Rodríguez*
Affiliation:
Fluid Mechanics Group, Carlos III University of Madrid, Leganes, Madrid, 28911, Spain
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The translation of a bubble under the action of an acoustic forcing finds applications in fields ranging from drug delivery to sonoluminescence. This phenomenon has been widely studied for cases where the amplitude of the forcing remains constant over time. However, in many practical applications, the duration of the forcing is not long enough for the bubble to attain a constant translational velocity, mainly due to the effect of the history force. Here, we develop a formulation, valid in the limit of very viscous flow and small-amplitude acoustic forcing, that allows us to describe the transient dynamics of bubbles driven by acoustic pulses consisting of finite numbers of cycles. We also present an asymptotic solution to this theory for the case of a finite-duration sinusoidal pressure pulse. This solution takes into account both the history integral term and the transient period that the bubble needs to achieve steady radial oscillations, the former being dominant during most of the acceleration process. Moreover, by introducing some additional assumptions, we derive a simplified formula that describes the time evolution of the bubble velocity fairly well. Using this solution, we show that the convergence to the steady translational velocity, given by the so-called Bjerknes force, occurs rather slowly, namely as 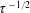$\unicode[STIX]{x1D70F}^{-1/2}$, where $\unicode[STIX]{x1D70F}$ is the time made dimensionless with the viscous time scale of the bubble, which explains the slow convergence of the bubble velocity and stresses the importance of taking the history force into account.

JFM classification

Drops and Bubbles: Bubble dynamics Biological Fluid Dynamics: Capsule/cell dynamics Low-Reynolds-number flows: Low-Reynolds-number flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 836 , 10 February 2018 , pp. 649 - 693
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abramowitz, M. & Stegun, I. 1964 Handbook of Mathematical Functions with Formulas, Graphs and Mathematical Tables. Dover.Google Scholar
Bjerknes, V. F. K. 1906 Fields of Force. The Columbia University Press.Google Scholar
Brodsky, E. E., Sturtevant, B. & Kanamori, H. 1998 Earthquakes, volcanoes, and rectified diffusion. J. Geophys. Res. 103, 23827–23838.Google Scholar
Chen, W.-S., Matula, T. J., Brayman, A. A. & Crum, L. A. 2003 A comparison of the fragmentation thresholds and inertial cavitation doses of different ultrasound contrast agents. J. Acoust. Soc. Am. 113, 643–651.Google Scholar
Dayton, P. A., Allen, J. S. & Ferrara, K. W. 2002 The magnitude of radiation force on ultrasound contrast agents. J. Acoust. Soc. Am. 112, 2183–2192.Google Scholar
Dollet, B., van der Meer, S. M., Garbin, V., de Jong, N., Lohse, D. & Versluis, M. 2008 Nonspherical oscillations of ultrasound contrast agent microbubbles. Ultrasound Med. Biol. 34, 1465–1473.Google Scholar
Faez, T., Emmer, M., Kooiman, K., Versluis, M., van der Steen, A. F. W. & de Jong, N. 2013 20 years of ultrasound contrast agent modeling. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 60, 7–20.Google Scholar
Garbin, V., Dollet, B., Overvelde, M., Cojoc, D., Fabrizio, E. D., van Wijngaarden, L., Prosperetti, A., de Jong, N., Lohse, D. & Versluis, M. 2009 History force on coated microbubbles propelled by ultrasound. Phys. Fluids 21, 029003.Google Scholar
Keller, J. B. & Miksis, M. 1980 Bubble oscillations of large amplitude. J. Acoust. Soc. Am. 68, 628–633.Google Scholar
Kim, I., Elghobashi, S. & Sirignano, W. A. 1998 On the equation for spherical-particle motion: effect of Reynolds and acceleration numbers. J. Fluid Mech. 367, 221–253.Google Scholar
Leighton, T. G. 1994 The Acoustic Bubble. Academic Press.Google Scholar
Magnaudet, J. & Legendre, D. 1998 A note on memory-integral contributions to the force on an accelerating spherical drop at low Reynolds number. Phys. Fluids 10, 550–554.Google Scholar
Manga, M. & Brodsky, E. E. 2006 Seismic triggering of eruptions in the far field: volcanoes and geysers. Annu. Rev. Earth Planet. Sci. 34, 263–291.Google Scholar
Maxey, M. R. & Riley, J. J. 1983 Equation of motion for a small rigid sphere in a nonuniform flow. Phys. Fluids 26, 883–889.Google Scholar
Medina-Palomo, A.2015 Experimental and analytical study of the interaction between short acoustic pulses and small clouds of microbubbles. PhD thesis, Carlos III University of Madrid.Google Scholar
Modestino, M. A., Hashemi, S. M. H. & Haussener, S. 2016 Mass transport aspects of electrochemical solar-hydrogen generation. Energy Environ. Sci. 9, 1533–1551.Google Scholar
Prosperetti, A. 1977 Thermal effects and damping mechanisms in the forced radial oscillations of gas bubbles in liquids. J. Acoust. Soc. Am. 61, 17–27.Google Scholar
Rensen, J., Bosman, D., Magnaudet, J., Ohl, C.-D., Prosperetti, A., Toegel, R., Versluis, M. & Lohse, D. 2001 Spiraling bubbles: how acoustic and hydrodynamic forces compete. Phys. Rev. Lett. 86, 4819–4822.Google Scholar
Riley, N. 2001 Steady streaming. Annu. Rev. Fluid Mech. 33, 43–65.Google Scholar
Rodríguez-Rodríguez, J. & Martínez-Bazán, C.2017 Rectified diffusion of a spherical gas bubble rising in a liquid pool at finite Reynolds number (in preparation).Google Scholar
Romero, L. A., Torczynski, J. R. & von Winckel, G. 2014 Terminal velocity of a bubble in a vertically vibrated liquid. Phys. Fluids 26, 053301.Google Scholar
Stepanyants, Y. A. & Yeoh, G. H. 2009 Particle and bubble dynamics in a creeping flow. Eur. J. Mech. (B/Fluids) 28, 619–629.Google Scholar
Sturtevant, B., Kanamori, H. & Brodsky, E. E. 1996 Seismic triggering by rectified diffusion in geothermal systems. J. Geophys. Res. 101, 25269–25282.Google Scholar
Toegel, R., Luther, S. & Lohse, D. 2006 Viscosity destabilizes sonoluminescing bubbles. Phys. Rev. Lett. 96, 114301.Google Scholar
Toilliez, J. O. & Szeri, A. J. 2008 Optimized translation of microbubbles driven by acoustic fields. J. Acoust. Soc. Am. 123, 1916–1930.Google Scholar
Yang, S.-M. & Leal, L. G. 1991 A note on memory-integral contributions to the force on an accelerating spherical drop at low Reynolds number. Phys. Fluids 3, 1822–1824.Google Scholar