Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-27T04:19:15.322Z Has data issue: false hasContentIssue false
  • English
  • Français

Spreading dynamics and contact angle of completely wetting volatile drops

Published online by Cambridge University Press:  12 April 2018

Etienne Jambon-Puillet Open the ORCID record for Etienne Jambon-Puillet [Opens in a new window] ,
Odile Carrier ,
Noushine Shahidzadeh ,
David Brutin ,
Jens Eggers  and
Daniel Bonn
Etienne Jambon-Puillet*
Affiliation:
Institute of Physics, Van der Waals-Zeeman Institute, University of Amsterdam, Science Park 904, 1098 XH Amsterdam, The Netherlands
Odile Carrier
Affiliation:
Institute of Physics, Van der Waals-Zeeman Institute, University of Amsterdam, Science Park 904, 1098 XH Amsterdam, The Netherlands
Noushine Shahidzadeh
Affiliation:
Institute of Physics, Van der Waals-Zeeman Institute, University of Amsterdam, Science Park 904, 1098 XH Amsterdam, The Netherlands
David Brutin
Affiliation:
Aix-Marseille University, IUSTI UMR 7343, 13453 Marseille, France
Jens Eggers
Affiliation:
School of Mathematics – University of Bristol, University Walk, Bristol BS8 1TW, UK
Daniel Bonn
Affiliation:
Institute of Physics, Van der Waals-Zeeman Institute, University of Amsterdam, Science Park 904, 1098 XH Amsterdam, The Netherlands
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

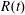The spreading of evaporating drops without a pinned contact line is studied experimentally and theoretically, measuring the radius $R(t)$ of completely wetting alkane drops of different volatility on glass. Initially the drop spreads (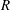$R$ increases), then owing to evaporation reverses direction and recedes with an almost constant non-zero contact angle 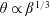$\unicode[STIX]{x1D703}\propto \unicode[STIX]{x1D6FD}^{1/3}$, where 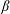$\unicode[STIX]{x1D6FD}$ measures the rate of evaporation; eventually the drop vanishes at a finite-time singularity. Our theory, based on a first-principles hydrodynamic description, well reproduces the dynamics of 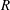$R$ and the value of 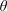$\unicode[STIX]{x1D703}$ during retraction.

JFM classification

Phase change: Condensation/evaporation Interfacial Flows (free surface): Contact lines Drops and Bubbles: Drops
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 844 , 10 June 2018 , pp. 817 - 830
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alizadeh Pahlavan, A., Cueto-Felgueroso, L., McKinley, G. H. & Juanes, R. 2015 Thin films in partial wetting: internal selection of contact-line dynamics. Phys. Rev. Lett. 115, 034502.10.1103/PhysRevLett.115.034502Google Scholar
Allen, J. S. 2003 An analytical solution for determination of small contact angles from sessile drops of arbitrary size. J. Colloid Interface Sci. 261 (2), 481–489.10.1016/S0021-9797(03)00127-9Google Scholar
Berezhnoi, A. N. & Semenov, A. V. 1997 Binary Diffusion Coefficients of Liquid Vapors in Gases. Begell House.Google Scholar
Beverley, K. J., Clint, J. H. & Fletcher, P. D. I. 1999 Evaporation rates of pure liquids measured using a gravimetric technique. Phys. Chem. Chem. Phys. 1, 149–153.10.1039/a805344hGoogle Scholar
Bonn, D., Eggers, J., Indekeu, J., Meunier, J. & Rolley, E. 2009 Wetting and spreading. Rev. Mod. Phys. 81, 739–805.10.1103/RevModPhys.81.739Google Scholar
Bonn, D. & Meunier, J. 1997 Comment on ‘evaporation preempts complete wetting’. Europhys. Lett. 39 (3), 341–342.10.1209/epl/i1997-00358-9Google Scholar
Bourges-Monnier, C. & Shanahan, M. E. R. 1995 Influence of evaporation on contact angle. Langmuir 11 (7), 2820–2829.10.1021/la00007a076Google Scholar
Cachile, M., Bénichou, O. & Cazabat, A. M. 2002a Evaporating droplets of completely wetting liquids. Langmuir 18 (21), 7985–7990.10.1021/la020231eGoogle Scholar
Cachile, M., Bénichou, O., Poulard, C. & Cazabat, A. M. 2002b Evaporating droplets. Langmuir 18 (21), 8070–8078.10.1021/la0204646Google Scholar
Carruth, G. F. & Kobayashi, R. 1973 Vapor pressure of normal paraffins ethane through n-decane from their triple points to about 10 mm mercury. J. Chem. Engng Data 18 (2), 115–126.10.1021/je60057a009Google Scholar
Cazabat, A. M. & Guena, G. 2010 Evaporation of macroscopic sessile droplets. Soft Matt. 6, 2591–2612.10.1039/b924477hGoogle Scholar
Deegan, R. D., Bakajin, O., Dupont, T. F., Huber, G., Nagel, S. R. & Witten, T. A. 1997 Capillary flow as the cause of ring stains from dried liquid drops. Nature 389 (6653), 827–829.10.1038/39827Google Scholar
Deegan, R. D., Bakajin, O., Dupont, T. F., Huber, G., Nagel, S. R. & Witten, T. A. 2000 Contact line deposits in an evaporating drop. Phys. Rev. E 62, 756–765.Google Scholar
Dunn, G. J., Wilson, S. K., Duffy, B. R. & Sefiane, K. 2009 Evaporation of a thin droplet on a thin substrate with a high thermal resistance. Phys. Fluids 21 (5), 052101.10.1063/1.3121214Google Scholar
Eggers, J. & Fontelos, M. A. 2015 Singularities: Formation, Structure, and Propagation. Cambridge University Press.10.1017/CBO9781316161692Google Scholar
Eggers, J. & Pismen, L. M. 2010 Nonlocal description of evaporating drops. Phys. Fluids 22 (11), 112101.10.1063/1.3491133Google Scholar
Elbaum, M., Lipson, S. G. & Wettlaufer, J. S. 1995 Evaporation preempts complete wetting. Europhys. Lett. 29 (6), 457–462.10.1209/0295-5075/29/6/005Google Scholar
Erbil, H. Y. 2012 Evaporation of pure liquid sessile and spherical suspended drops: a review. Adv. Colloid Interface Sci. 170 (1–2), 67–86.10.1016/j.cis.2011.12.006Google Scholar
de Gennes, P. G. 1985 Wetting: statics and dynamics. Rev. Mod. Phys. 57, 827–863.10.1103/RevModPhys.57.827Google Scholar
Hu, H. & Larson, R. G. 2006 Marangoni effect reverses coffee-ring depositions. J. Phys. Chem. B 110 (14), 7090–7094.10.1021/jp0609232Google Scholar
Israelachvili, J. N. 2011 Intermolecular and Surface Forces. Academic Press.Google Scholar
Jackson, J. D. 1975 Classical Electrodynamics. Wiley.Google Scholar
Janeček, V., Andreotti, B., Pražák, D., Bárta, T. & Nikolayev, V. S. 2013 Moving contact line of a volatile fluid. Phys. Rev. E 88, 060404.Google Scholar
Kelly-Zion, P. L., Pursell, C. J., Vaidya, S. & Batra, J. 2011 Evaporation of sessile drops under combined diffusion and natural convection. Colloids Surf. A 381 (1–3), 31–36.10.1016/j.colsurfa.2011.03.020Google Scholar
Larson, R. G. 2014 Transport and deposition patterns in drying sessile droplets. AIChE J. 60 (5), 1538–1571.10.1002/aic.14338Google Scholar
Lee, K. S., Cheah, C. Y., Copleston, R. J., Starov, V. M. & Sefiane, K. 2008 Spreading and evaporation of sessile droplets: universal behaviour in the case of complete wetting. Colloids Surf. A 323 (1–3), 63–72.10.1016/j.colsurfa.2007.09.033Google Scholar
Morris, S. J. S. 2014 On the contact region of a diffusion-limited evaporating drop: a local analysis. J. Fluid Mech. 739, 308–337.10.1017/jfm.2013.577Google Scholar
Perrin, H., Lhermerout, R., Davitt, K., Rolley, E. & Andreotti, B. 2016 Defects at the nanoscale impact contact line motion at all scales. Phys. Rev. Lett. 116, 184502.10.1103/PhysRevLett.116.184502Google Scholar
Poulard, C., Guéna, G., Cazabat, A. M., Boudaoud, A. & Ben Amar, M. 2005 Rescaling the dynamics of evaporating drops. Langmuir 21 (18), 8226–8233.10.1021/la050406vGoogle Scholar
Rednikov, A. & Colinet, P. 2013 Singularity-free description of moving contact lines for volatile liquids. Phys. Rev. E 87, 010401.Google Scholar
Savino, R. & Fico, S. 2004 Transient Marangoni convection in hanging evaporating drops. Phys. Fluids 16 (10), 3738–3754.10.1063/1.1772380Google Scholar
Saxton, M. A., Whiteley, J. P., Vella, D. & Oliver, J. M. 2016 On thin evaporating drops: When is the d 2 -law valid? J. Fluid Mech. 792, 134–167.10.1017/jfm.2016.76Google Scholar
Semenov, S., Starov, V. M., Rubio, R. G. & Velarde, M. G. 2012 Computer simulations of evaporation of pinned sessile droplets: influence of kinetic effects. Langmuir 28 (43), 15203–15211.10.1021/la303916uGoogle Scholar
Shahidzadeh-Bonn, N., Rafai, S., Azouni, A. & Bonn, D. 2006 Evaporating droplets. J. Fluid Mech. 549, 307–313.10.1017/S0022112005008190Google Scholar
Snoeijer, J. H. & Andreotti, B. 2013 Moving contact lines: scales, regimes, and dynamical transitions. Annu. Rev. Fluid Mech. 45 (1), 269–292.10.1146/annurev-fluid-011212-140734Google Scholar