Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-06T12:43:00.171Z Has data issue: false hasContentIssue false
  • English
  • Français

Near-onset dynamics in natural doubly diffusive convection

Published online by Cambridge University Press:  21 January 2022

Cédric Beaume Open the ORCID record for Cédric Beaume [Opens in a new window] ,
Alastair M. Rucklidge Open the ORCID record for Alastair M. Rucklidge [Opens in a new window]  and
Joanna Tumelty
Cédric Beaume*
Affiliation:
School of Mathematics, University of Leeds, LeedsLS2 9JT, UK
Alastair M. Rucklidge
Affiliation:
School of Mathematics, University of Leeds, LeedsLS2 9JT, UK
Joanna Tumelty
Affiliation:
School of Mathematics, University of Leeds, LeedsLS2 9JT, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

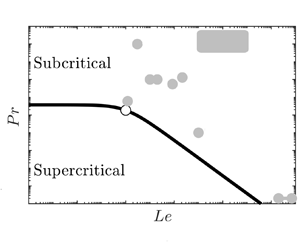
Doubly diffusive convection is considered in a vertical slot where horizontal temperature and solutal variations provide competing effects to the fluid density while allowing the existence of a conduction state. In this configuration, the linear stability of the conductive state is known, but the convection patterns arising from the primary instability have only been studied for specific parameter values. We have extended this by determining the nature of the primary bifurcation for all values of the Lewis and Prandtl numbers using a weakly nonlinear analysis. The resulting convection branches are extended using numerical continuation and we find large-amplitude steady convection states can coexist with the stable conduction state for sub- and supercritical primary bifurcations. The stability of the convection states is investigated and attracting travelling waves and periodic orbits are identified using time stepping when these steady states are unstable.

JFM classification

Convection: Double diffusive convection Nonlinear Dynamical Systems: Bifurcation
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 934 , 10 March 2022 , A42
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Batiste, O., Knobloch, E., Mercader, I. & Net, M. 2001 Simulations of oscillatory binary fluid convection in large aspect ratio containers. Phys. Rev. E 65, 016303.CrossRefGoogle ScholarPubMed
Beaume, C. 2017 Adaptive Stokes preconditioning for steady incompressible flows. Commun. Comput. Phys. 22 (2), 494–516.CrossRefGoogle Scholar
Beaume, C. 2020 Transition to doubly diffusive chaos. Phys. Rev. Fluids 5 (10), 103903.CrossRefGoogle Scholar
Beaume, C., Bergeon, A. & Knobloch, E. 2011 Homoclinic snaking of localized states in doubly diffusive convection. Phys. Fluids 23, 094102.CrossRefGoogle Scholar
Beaume, C., Bergeon, A. & Knobloch, E. 2013 a Convectons and secondary snaking in three-dimensional natural doubly diffusive convection. Phys. Fluids 25 (2), 024105.CrossRefGoogle Scholar
Beaume, C., Bergeon, A. & Knobloch, E. 2013 b Nonsnaking doubly diffusive convectons and the twist instability. Phys. Fluids 25, 114102.CrossRefGoogle Scholar
Beaume, C., Bergeon, A. & Knobloch, E. 2018 Three-dimensional doubly diffusive convectons: instability and transition to complex dynamics. J. Fluid Mech. 840, 74–105.CrossRefGoogle Scholar
Bergeon, A., Ghorayeb, K. & Mojtabi, A. 1999 Double diffusive convection in an inclined cavity. Phys. Fluids 11, 549–559.CrossRefGoogle Scholar
Bergeon, A. & Knobloch, E. 2002 Natural doubly diffusive convection in three-dimensional enclosures. Phys. Fluids 14 (9), 3233–3250.CrossRefGoogle Scholar
Bergeon, A. & Knobloch, E. 2008 a Periodic and localized states in natural doubly diffusive convection. Physica D 237 (8), 1139–1150.CrossRefGoogle Scholar
Bergeon, A. & Knobloch, E. 2008 b Spatially localized states in natural doubly diffusive convection. Phys. Fluids 20 (3), 034102.CrossRefGoogle Scholar
Bethe, H.A. 1990 Supernova mechanisms. Rev. Mod. Phys. 62, 801–866.CrossRefGoogle Scholar
Clever, R.M. & Busse, F.H. 1981 Low-Prandtl-number convection in a layer heated from below. J. Fluid Mech. 102, 61–74.CrossRefGoogle Scholar
Cross, M.C., Daniels, P.G., Hohenberg, P.C. & Siggia, E.D. 1983 Phase-winding solutions in a finite container above the convective threshold. J. Fluid Mech. 127, 155–183.CrossRefGoogle Scholar
Deane, A.E., Knobloch, E. & Toomre, J. 1988 Traveling waves in large-aspect-ratio thermosolutal convection. Phys. Rev. A 37, 1817–1820.CrossRefGoogle ScholarPubMed
Dijkstra, H.A. & Kranenborg, E.J. 1996 A bifurcation study of double diffusive flows in a laterally heated stably stratified liquid layer. Intl J. Heat Mass Transfer 39 (13), 2699–2710.CrossRefGoogle Scholar
Erenburg, V., Gelfgat, A.Y., Kit, E., Bar-Yoseph, P.Z. & Solan, A. 2003 Multiple states, stability and bifurcations of natural convection in a rectangular cavity with partially heated vertical walls. J. Fluid Mech. 492, 63–89.CrossRefGoogle Scholar
Garaud, P. 2018 Double-diffusive convection at low Prandtl number. Annu. Rev. Fluid Mech. 50, 275–298.CrossRefGoogle Scholar
Ghorayeb, K. & Mojtabi, A. 1997 Double diffusive convection in a vertical rectangular cavity. Phys. Fluids 9 (8), 2339–2348.CrossRefGoogle Scholar
Guckenheimer, J. & Holmes, P. 1983 Nonlinear Oscillations, Dynamical Systems and Bifurcations of Vector Fields. Springer.CrossRefGoogle Scholar
Huppert, H.E. & Sparks, R.S.J. 1984 Double diffusive convection due to crystallization in magmas. Annu. Rev. Earth Planet Sci. 12, 11–37.CrossRefGoogle Scholar
Huppert, H.E. & Turner, J.S. 1981 Double-diffusive convection. J. Fluid Mech. 106, 299–329.CrossRefGoogle Scholar
Karniadakis, G.E., Israeli, M. & Orszag, S.A. 1991 High-order splitting methods for the incompressible Navier–Stokes equations. J. Comput. Phys. 97 (2), 414–443.CrossRefGoogle Scholar
Kelley, D.E., Fernando, H.J.S., Gargett, A.E., Tanny, J. & Özsoy, E. 2003 The diffusive regime of double diffusive convection. Prog. Oceanogr. 56, 461–481.CrossRefGoogle Scholar
Knobloch, E. 2015 Spatial localization in dissipative systems. Annu. Rev. Condens. Matter Phys. 6, 325–359.CrossRefGoogle Scholar
Knobloch, E., Moore, D.R., Toomre, J. & Weiss, N.O. 1986 Transitions to chaos in two-dimensional double-diffusive convection. J. Fluid Mech. 166, 409–448.CrossRefGoogle Scholar
Knobloch, E., Uecker, H. & Wetzel, D. 2019 Defectlike structures and localized patterns in the cubic-quintic-septic Swift–Hohenberg equation. Phys. Rev. E 100 (1), 012204.CrossRefGoogle ScholarPubMed
Kolodner, P. 1991 Stable and unstable pulses of traveling-wave convection. Phys. Rev. A 43, 2827–2832.CrossRefGoogle ScholarPubMed
Krishnamurti, R. 2003 Double-diffusive transport in laboratory thermohaline staircases. J. Fluid Mech. 483, 287–314.CrossRefGoogle Scholar
Krishnamurti, R. 2009 Heat, salt and momentum transport in a laboratory thermohaline staircase. J. Fluid Mech. 638, 491–506.CrossRefGoogle Scholar
Lay, T., Hernlund, J. & Buffett, B.A. 2008 Core–mantle boundary heat flow. Nat. Geosci. 1, 25–32.CrossRefGoogle Scholar
Mamou, M., Vasseur, P. & Bilgen, E. 1998 Double-diffusive convection instability in a vertical porous enclosure. J. Fluid Mech. 368, 263–289.CrossRefGoogle Scholar
Mamun, C.K. & Tuckerman, L.S. 1995 Asymmetry and Hopf bifurcation in spherical Couette flow. Phys. Fluids 7 (1), 80–91.CrossRefGoogle Scholar
Matthews, P.C., Proctor, M.R.E., Rucklidge, A.M. & Weiss, N.O. 1993 Pulsating waves in nonlinear magnetoconvection. Phys. Lett. A 183 (1), 69–75.CrossRefGoogle Scholar
Mercader, I., Batiste, O., Alonso, A. & Knobloch, E. 2009 Localized pinning states in closed containers: homoclinic snaking without bistability. Phys. Rev. E 80, 025201.CrossRefGoogle ScholarPubMed
Mercader, I., Batiste, O., Alonso, A. & Knobloch, E. 2011 Convectons, anticonvectons and multiconvectons in binary fluid convection. J. Fluid Mech. 667, 586–606.CrossRefGoogle Scholar
Paliwal, R.C. & Chen, C.F. 1980 a Double-diffusive instability in an inclined fluid layer. Part 1. Experimental investigation. J. Fluid Mech. 98, 755–768.CrossRefGoogle Scholar
Paliwal, R.C. & Chen, C.F. 1980 b Double-diffusive instability in an inclined fluid layer. Part 2. Stability analysis. J. Fluid Mech. 98, 769–785.CrossRefGoogle Scholar
Pérez-Santos, I., Garcés-Vargas, J., Schneider, W., Ross, L., Parra, S. & Valle-Levinson, A. 2014 Double-diffusive layering and mixing in patagonian fjords. Prog. Oceanogr. 129, 35–49.CrossRefGoogle Scholar
Predtechensky, A.A., McCormich, W.D., Swift, J.B., Noszticzius, Z. & Swinney, H.L. 1994 Onset of traveling waves in isothermal double diffusive convection. Phys. Rev. Lett. 72, 218–221.CrossRefGoogle ScholarPubMed
Requilé, Y., Hirata, S.C., Ouarzazi, M.N. & Barletta, A. 2020 Weakly nonlinear analysis of viscous dissipation thermal instability in plane Poiseuille and plane Couette flows. J. Fluid Mech. 886, A26.CrossRefGoogle Scholar
Rucklidge, A.M. 1992 Chaos in models of double convection. J. Fluid Mech. 237, 209–229.CrossRefGoogle Scholar
Schmitt, R.W. 1983 The characteristics of salt fingers in a variety of fluid systems, including stellar interiors, liquid metals, oceans, and magmas. Phys. Fluids 26 (9), 2373–2377.CrossRefGoogle Scholar
Schmitt, R.W. 1994 Double diffusion in oceanography. Annu. Rev. Fluid Mech. 26, 255–285.CrossRefGoogle Scholar
Schmitt, R.W., Ledwell, J.R., Montgomery, E.T., Polzin, K.L. & Toole, J.M. 2005 Enhanced diapycnal mixing by salt fingers in the thermocline of the tropical Atlantic. Science 308 (5722), 685–688.CrossRefGoogle ScholarPubMed
Schmitt, R.W., Perkins, H., Boyd, J.D. & Stalcup, M.C. 1987 C-SALT: an investigation of the thermohaline staircase in the western tropical North Atlantic. Deep-Sea Res. 34 (10), 1655–1665.CrossRefGoogle Scholar
Shankar, B.M., Kumar, J. & Shivakumara, I.S. 2021 Stability of double-diffusive natural convection in a vertical fluid layer. Phys. Fluids 33 (9), 094113.CrossRefGoogle Scholar
Spiegel, E.A. 1969 Semiconvection. Comments on Astrophysics and Space Physics, 1–57.Google Scholar
Spiegel, E.A. 1972 Convection in stars II. Special effects. Annu. Rev. Astron. Astrophys. 10, 261–304.CrossRefGoogle Scholar
Spina, A., Toomre, J. & Knobloch, E. 1998 Confined states in large-aspect-ratio thermosolutal convection. Phys. Rev. E 57, 524–547.CrossRefGoogle Scholar
Tsitverblit, N. 1995 Bifurcation phenomena in confined thermosolutal convection with lateral heating: commencement of the double-diffusive region. Phys. Fluids 7 (4), 718–736.CrossRefGoogle Scholar
Tsitverblit, N. & Kit, E. 1993 The multiplicity of steady flows in confined double-diffusive convection with lateral heating. Phys. Fluids A 5 (4), 1062–1064.CrossRefGoogle Scholar
Umbría, J.S. & Net, M. 2019 Stationary flows and periodic dynamics of binary mixtures in tall laterally heated slots. In Computational Modelling of Bifurcations and Instabilities in Fluid Dynamics (ed. A. Gelfgat), pp. 171–216. Springer.CrossRefGoogle Scholar
Watanabe, T., Iima, M. & Nishiura, Y. 2012 Spontaneous formation of travelling localized structures and their asymptotic behaviour in binary fluid convection. J. Fluid Mech. 712, 219–243.CrossRefGoogle Scholar
Watanabe, T., Iima, M. & Nishiura, Y. 2016 A skeleton of collision dynamics: hierarchical network structure among even-symmetric steady pulses in binary fluid convection. SIAM J. Appl. Dyn. Syst. 15, 789–806.CrossRefGoogle Scholar
Wilcox, W.R. 1993 Transport phenomena in crystal growth from solution. Prog. Cryst. Growth Charact. Mater. 26, 153–194.CrossRefGoogle Scholar
Xin, S., Le Quéré, P. & Tuckerman, L.S. 1998 Bifurcation analysis of double-diffusive convection with opposing horizontal thermal and solutal gradients. Phys. Fluids 10 (4), 850–858.CrossRefGoogle Scholar
You, Y. 2002 A global ocean climatological atlas of the turner angle: implications for double-diffusion and water-mass structure. Deep-Sea Res. 49, 2075–2093.CrossRefGoogle Scholar