Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-19T05:45:22.048Z Has data issue: false hasContentIssue false
  • English
  • Français

Moving contact-line mobility measured

Published online by Cambridge University Press:  01 March 2018

Yi Xia Open the ORCID record for Yi Xia [Opens in a new window]  and
Paul H. Steen Open the ORCID record for Paul H. Steen [Opens in a new window]
Yi Xia*
Affiliation:
School of Mechanical and Aerospace Engineering, Cornell University, Ithaca, NY 14853, USA
Paul H. Steen*
Affiliation:
School of Mechanical and Aerospace Engineering, Cornell University, Ithaca, NY 14853, USA School of Chemical and Biomolecular Engineering, Cornell University, Ithaca, NY 14853, USA
*
†Email addresses for correspondence: [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

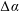Contact-line mobility characterizes how fast a liquid can wet or unwet a solid support by relating the contact angle $\unicode[STIX]{x0394}\unicode[STIX]{x1D6FC}$ to the contact-line speed 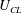$U_{CL}$. The contact angle changes dynamically with contact-line speeds during rapid movement of liquid across a solid. Speeds beyond the region of stick–slip are the focus of this experimental paper. For these speeds, liquid inertia and surface tension compete while damping is weak. The mobility parameter 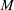$M$ is defined empirically as the proportionality, when it exists, between $\unicode[STIX]{x0394}\unicode[STIX]{x1D6FC}$ and $U_{CL}$, 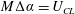$M\unicode[STIX]{x0394}\unicode[STIX]{x1D6FC}=U_{CL}$. We discover that $M$ exists and measure it. The experimental approach is to drive the contact line of a sessile drop by a plane-normal oscillation of the drop’s support. Contact angles, displacements and speeds of the contact line are measured. To unmask the mobility away from stick–slip, the diagram of 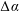$\unicode[STIX]{x0394}\unicode[STIX]{x1D6FC}$ against $U_{CL}$, the traditional diagram, is remapped to a new diagram by rescaling with displacement. This new diagram reveals a regime where $\unicode[STIX]{x0394}\unicode[STIX]{x1D6FC}$ is proportional to 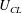$U_{CL}$ and the slope yields the mobility 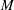$M$. The experimental approach reported introduces the cyclically dynamic contact angle goniometer. The concept and method of the goniometer are illustrated with data mappings for water on a low-hysteresis non-wetting substrate.

JFM classification

Interfacial Flows (free surface): Capillary flows Interfacial Flows (free surface): Contact lines Drops and Bubbles: Drops
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 841 , 25 April 2018 , pp. 767 - 783
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Andrieu, C., Sykes, C. & Brochard, F. 1994 Average spreading parameter on heterogeneous surfaces. Langmuir 10 (7), 2077–2080.CrossRefGoogle Scholar
Aussillous, P. & Quéré, D. 2004 Shapes of rolling liquid drops. J. Fluid Mech. 512, 133–151.CrossRefGoogle Scholar
Batchelor, G. K. 1999 An Introduction to Fluid Dynamics. Cambridge University Press.Google Scholar
Bird, J. C., Mandre, S. & Stone, H. A. 2008 Short-time dynamics of partial wetting. Phys. Rev. Lett. 100 (23), 234501.CrossRefGoogle ScholarPubMed
Blake, T. D. 2006 The physics of moving wetting lines. J. Colloid Interface Sci. 299 (1), 1–13.CrossRefGoogle ScholarPubMed
Blake, T. D. & Ruschak, K. J. 1979 A maximum speed of wetting. Nature 282 (5738), 489–491.CrossRefGoogle Scholar
Bonn, D., Eggers, J., Indekeu, J. & Meunier, J. 2009 Wetting and spreading. Rev. Mod. Phys. 81 (2), 739–805.CrossRefGoogle Scholar
Borkar, A. & Tsamopoulos, J. 1991 Boundary-layer analysis of the dynamics of axisymmetric capillary bridges. Phys. Fluids A 3 (12), 2866–2874.CrossRefGoogle Scholar
Bostwick, J. B. & Steen, P. H. 2014 Dynamics of sessile drops. Part 1. Inviscid theory. J. Fluid Mech. 760, 5–38.CrossRefGoogle Scholar
Carlson, A., Bellani, G. & Amberg, G. 2012 Contact line dissipation in short-time dynamic wetting. Europhys. Lett. 97 (4), 44004.CrossRefGoogle Scholar
Chang, C.-T., Bostwick, J. B., Daniel, S. & Steen, P. H. 2015 Dynamics of sessile drops. Part 2. Experiment. J. Fluid Mech. 768, 442–467.CrossRefGoogle Scholar
Chang, C.-T., Bostwick, J. B., Steen, P. H. & Daniel, S. 2013 Substrate constraint modifies the Rayleigh spectrum of vibrating sessile drops. Phys. Rev. E 88, 23015.Google ScholarPubMed
Cheng, N.-S. 2008 Formula for the viscosity of a glycerol–water mixture. Ind. Engng Chem. Res. 47 (9), 3285–3288.CrossRefGoogle Scholar
Davis, S. H. 1980 Moving contact lines and rivulet instabilities. Part 1. The static rivulet. J. Fluid Mech. 98, 225–242.CrossRefGoogle Scholar
Davis, S. H. 1983 Contact-line problems in fluid mechanics. J. Appl. Mech. 50, 977–982.CrossRefGoogle Scholar
Decker, E. L. & Garoff, S. 1996 Using vibrational noise to probe energy barriers producing contact angle hysteresis. Langmuir 12 (8), 2100–2110.CrossRefGoogle Scholar
Diez, J. A., Kondic, L. & Bertozzi, A. L. 2000 Global models for moving contact lines. Phys. Rev. E 63 (1), 011208.Google ScholarPubMed
Dussan, V. E. B. 1979 On the spreading of liquids on solid surfaces: static and dynamic contact lines. Annu. Rev. Fluid Mech. 11 (1968), 371–400.CrossRefGoogle Scholar
Elliott, G. E. P. & Riddiford, A. C. 1967 Dynamic contact angles I. The effect of impressed motion. J. Colloid Interface Sci. 23, 389–398.CrossRefGoogle Scholar
Fayzrakhmanova, I. S. & Straube, A. V. 2009 Stick-slip dynamics of an oscillated sessile drop. Phys. Fluids 21 (7), 072104.CrossRefGoogle Scholar
Glycerine Producers’ Association 1963 Physical Properties of Glycerine and its Solutions. New York.Google Scholar
Gnanappa, A. K., Gogolides, E., Evangelista, F. & Riepen, M. 2011 Contact line dynamics of a superhydrophobic surface: application for immersion lithography. Microfluid. Nanofluid. 10 (6), 1351–1357.CrossRefGoogle Scholar
Hocking, L. M. 1987 The damping of capillary-gravity waves at a rigid boundary. J. Fluid Mech. 179, 253–266.CrossRefGoogle Scholar
Hodges, S. R., Jensen, O. E. & Rallison, J. M. 2004 Sliding, slipping and rolling: the sedimentation of a viscous drop down a gently inclined plane. J. Fluid Mech. 512, 95–131.CrossRefGoogle Scholar
Jiang, L., Perlin, M. & Schultz, W. W. 2004 Contact-line dynamics and damping for oscillating free surface flows. Phys. Fluids 16 (3), 748–758.CrossRefGoogle Scholar
Kocourek, V., Karcher, Ch., Conrath, M. & Schulze, D. 2006 Stability of liquid metal drops affected by a high-frequency magnetic field. Phys. Rev. E 74 (2), 026303.Google ScholarPubMed
Korenchenko, A. E. & Malkova, J. P. 2015 Numerical investigation of phase relationships in an oscillating sessile drop. Phys. Fluids 27 (10), 102104.CrossRefGoogle Scholar
Krumpfer, J. W. & McCarthy, T. J. 2010 Contact angle hysteresis: a different view and a trivial recipe for low hysteresis hydrophobic surfaces. Faraday Discuss. 146, 103–111.CrossRefGoogle Scholar
Krupenkin, T. & Taylor, J. A. 2011 Reverse electrowetting as a new approach to high-power energy harvesting. Nat. Commun. 2, 448.CrossRefGoogle ScholarPubMed
Kumar, G. & Prabhu, K. N. 2007 Review of non-reactive and reactive wetting of liquids on surfaces. Adv. Colloid Interface Sci. 133 (2), 61–89.CrossRefGoogle ScholarPubMed
Lyubimov, D. V., Lyubimova, T. P. & Shklyaev, S. V. 2004 Non-axisymmetric oscillations of a hemispherical drop. Fluid Dyn. 39 (6), 851–862.CrossRefGoogle Scholar
Lyubimov, D. V., Lyubimova, T. P. & Shklyaev, S. V. 2006 Behavior of a drop on an oscillating solid plate. Phys. Fluids 18 (1), 012101.CrossRefGoogle Scholar
Mahadevan, L. & Pomeau, Y. 1999 Rolling droplets. Phys. Fluids 11 (9), 2449–2453.CrossRefGoogle Scholar
Moffat, J. R., Sefiane, K. & Shanahan, M. E. R. 2009 Effect of TiO2 nanoparticles on contact line stick-slip behavior of volatile drops. J. Phys. Chem. B 113 (26), 8860–8866.CrossRefGoogle ScholarPubMed
Noblin, X., Buguin, A. & Brochard-Wyart, F. 2004 Vibrated sessile drops: transition between pinned and mobile contact line oscillations. Eur. Phys. J. E 14 (4), 395–404.Google ScholarPubMed
Noblin, X., Buguin, A. & Brochard-Wyart, F. 2009 Vibrations of sessile drops. Eur. Phys. J. 166 (1), 7–10.Google Scholar
Oh, J. M., Ko, S. H. & Kang, K. H. 2008 Shape oscillation of a drop in AC electrowetting. Langmuir 24 (15), 8379–8386.CrossRefGoogle ScholarPubMed
Perlin, M., Schultz, W. W. & Liu, Z. 2004 High Reynolds number oscillating contact lines. Wave Motion 40 (1), 41–56.CrossRefGoogle Scholar
Pomeau, Y. 2001 Moving contact line. Le Journal de Physique IV 11 (PR6), Pr6–199–Pr6–212.Google Scholar
Savva, N. & Kalliadasis, S. 2011 Dynamics of moving contact lines: a comparison between slip and precursor film models. Europhys. Lett. 94 (6), 64004.CrossRefGoogle Scholar
Schweizer, P. M. & Kistler, S. F. 2012 Liquid Film Coating: Scientific Principles and their Technological Implications. Springer Science & Business Media.Google Scholar
Sen, P. & Kim, C. J. 2009 A fast liquid-metal droplet microswitch using EWOD-driven contact-line sliding. J. Microelectromech. Syst. 18 (1), 174–185.CrossRefGoogle Scholar
Shen, C. & Ruth, D. W. 1998 Experimental and numerical investigations of the interface profile close to a moving contact line. Phys. Fluids 10 (4), 789–799.CrossRefGoogle Scholar
Snoeijer, J. H. & Andreotti, B. 2013 Moving contact lines: scales, regimes, and dynamical transitions. Annu. Rev. Fluid Mech. 45 (1), 269–292.CrossRefGoogle Scholar
Srinivasan, R. 2003 Estimating zero-g flow rates in open channels having capillary pumping vanes. Intl J. Numer. Meth. Fluids 41 (4), 389–417.CrossRefGoogle Scholar
Tanner, L. H. 1979 The spreading of silicone oil drops on horizontal surfaces. J. Phys. D 12 (9), 1473.CrossRefGoogle Scholar
Ting, C.-L. & Perlin, M. 1995 Boundary conditions in the vicinity of the contact line at a vertically oscillating upright plate: an experimental investigation. J. Fluid Mech. 295, 263–300.CrossRefGoogle Scholar
Vukasinovic, B., Smith, M. K. & Glezer, A. 2007 Dynamics of a sessile drop in forced vibration. J. Fluid Mech. 587, 395–423.CrossRefGoogle Scholar
Whitehill, J. D., Neild, A. & Stokes, M. H. 2012 Forced spreading behavior of droplets undergoing low frequency vibration. Colloids Surf. A 393, 144–152.CrossRefGoogle Scholar
Winkels, K. G., Weijs, J. H., Eddi, A. & Snoeijer, J. H. 2012 Initial spreading of low-viscosity drops on partially wetting surfaces. Phys. Rev. E 85 (5), 055301.Google ScholarPubMed
Xia, Y., Daniel, S. & Steen, P. 2017 Rapidly moving contact lines and damping contributions. Bull. Am. Phys. Soc. 62 (14), 177.Google Scholar
Yokoi, K., Vadillo, D., Hinch, J. & Hutchings, I. 2009 Numerical studies of the influence of the dynamic contact angle on a droplet impacting on a dry surface. Phys. Fluids 21 (7), 72102.CrossRefGoogle Scholar
Supplementary material: File

Xia and Steen supplementary material

Xia and Steen supplementary material 1

Download Xia and Steen supplementary material(File)
File 444.9 KB