Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-26T15:30:07.017Z Has data issue: false hasContentIssue false
  • English
  • Français

Moisture transfer by turbulent natural convection

Published online by Cambridge University Press:  15 July 2019

Lu Zhang Open the ORCID record for Lu Zhang [Opens in a new window] ,
Kai Leong Chong  and
Ke-Qing Xia Open the ORCID record for Ke-Qing Xia [Opens in a new window]
Lu Zhang
Affiliation:
Department of Physics, The Chinese University of Hong Kong, Shatin, Hong Kong, China Center for Complex Flows and Soft Matter Research and Department of Mechanics and Aerospace Engineering, Southern University of Science and Technology, Shenzhen, 518055, China
Kai Leong Chong
Affiliation:
Department of Physics, The Chinese University of Hong Kong, Shatin, Hong Kong, China
Ke-Qing Xia*
Affiliation:
Department of Physics, The Chinese University of Hong Kong, Shatin, Hong Kong, China Center for Complex Flows and Soft Matter Research and Department of Mechanics and Aerospace Engineering, Southern University of Science and Technology, Shenzhen, 518055, China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We present an experimental and numerical study of natural convection with moist air as convecting fluid. By simplifying the system as two-component convection, an experimental method is proposed for indirectly measuring the moisture transfer rates in buoyancy-driven flows. We verify the results using direct numerical simulations. It is found that the non-dimensionalized transfer rates for both sensible heat (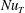$Nu_{T}$) and water vapour (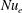$Nu_{e}$) are essentially determined by a generalized Grashof number 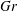$Gr$ (the ratio of combined buoyancy generated by the imposed temperature and vapour pressure gradients to viscous force), and are only weakly dependent on the buoyancy ratio 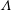$\unicode[STIX]{x1D6EC}$ (the ratio of buoyancy induced by temperature variation to that due to vapour pressure variation). Moreover, we show that the full set of control parameters 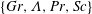$\{Gr,\unicode[STIX]{x1D6EC},Pr,Sc\}$ is more suitable than other choices for characterizing the two-component system under investigation. As a special case, the Schmidt number dependence for passive scalar transport rates in buoyancy-driven flows is also deduced.

JFM classification

Phase change: Condensation/evaporation Convection: Moist convection
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 874 , 10 September 2019 , pp. 1041 - 1056
Check for updates
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81 (2), 503–537.Google Scholar
Andrews, D. G. 2000 An Introduction to Atmospheric Physics. Cambridge University Press.Google Scholar
Arya, S. P. 1999 Air Pollution Meteorology and Dispersion. Oxford University Press.Google Scholar
Bergman, T. L., Incropera, F. P., DeWitt, D. P. & Lavine, A. S. 2011 Fundamentals of Heat and Mass Transfer. Wiley.Google Scholar
Bodenschatz, E., Malinowski, S. P., Shaw, R. A. & Stratmann, F. 2010 Can we understand clouds without turbulence? Science 327 (5968), 970–971.Google Scholar
Bretherton, C. S. 1987 A theory for nonprecipitating moist convection between two parallel plates. Part I. Thermodynamics and linear solutions. J. Atmos. Sci. 44 (14), 1809–1827.Google Scholar
Bretherton, C. S. 1988 A theory for nonprecipitating convection between two parallel plates. Part II. Nonlinear theory and cloud field organization. J. Atmos. Sci. 45 (17), 2391–2415.Google Scholar
Cane, M. A., Clement, A. C., Kaplan, A., Kushnir, Y., Pozdnyakov, D., Seager, R., Zebiak, S. E. & Murtugudde, R. 1997 Twentieth-century sea surface temperature trends. Science 275 (5302), 957–960.Google Scholar
Chandrakar, K. K., Cantrell, W., Chang, K., Ciochetto, D., Niedermeier, D., Ovchinnikov, M., Shaw, R. A. & Yang, F. 2016 Aerosol indirect effect from turbulence-induced broadening of cloud-droplet size distributions. Proc. Natl Acad. Sci. USA 113 (50), 14243–14248.Google Scholar
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35 (7), 58.Google Scholar
Chong, K.-L., Ding, G.-Y. & Xia, K.-Q. 2018 Multiple-resolution scheme in finite-volume code for active or passive scalar turbulence. J. Comput. Phys. 375, 1045–1058.Google Scholar
Durack, P. J., Wijffels, S. E. & Matear, R. J. 2012 Ocean salinities reveal strong global water cycle intensification during 1950 to 2000. Science 336, 455–458.Google Scholar
Emanuel, K. A. 1994 Atmospheric Convection. Oxford University Press.Google Scholar
Fairall, C. W., Bradley, E. F., Hare, J. E., Grachev, A. A. & Edson, J. B. 2003 Bulk parameterization of air-sea fluxes updates and verification for the COARE algorithm. J. Clim. 16 (4), 571–591.Google Scholar
Fairall, C. W., Bradley, E. F., Rogers, D. P., Edson, J. B. & Young, G. S. 1996 Bulk parameterization of air-sea fluxes for tropical ocean global atmosphere coupled ocean atmosphere response experiment. J. Geophys. Res. 101 (c2), 3747–3764.Google Scholar
Gent, P. R. & Mcwilliams, J. C. 1990 Isopycnal mixing in ocean circulation models. J. Phys. Oceanogr. 20 (1), 150–155.Google Scholar
Grossmann, S. & Lohse, D. 2001 Thermal convection for large Prandtl numbers. Phys. Rev. Lett. 86, 3316–3319.Google Scholar
Grossmann, S. & Lohse, D. 2002 Prandtl and Rayleigh number dependence of the Reynolds number in turbulent thermal convection. Phys. Rev. E 66, 016305.Google Scholar
Hernandez-Duenas, G., Majda, A. J., Smith, L. M. & Stechmann, S. N. 2013 Minimal models for precipitating turbulent convection. J. Fluid Mech. 717, 576–611.Google Scholar
Huppert, H. E. & Turner, J. S. 1981 Double-diffusive convection. J. Fluid Mech. 106, 299–329.Google Scholar
Landau, L. D. & Lifshitz, E. M. 2013 Fluid Mechanics. Elsevier Science.Google Scholar
Levitus, S., Antonov, J. I., Boyer, T. P. & Stephens, C. 2000 Warming of the world ocean. Science 287, 2225–2229.Google Scholar
Liu, W. T. 1979 Bulk parameterization of air-sea exchanges of heat and water vapor including the molecular constraints at the interface. J. Atmos. Sci. 36 (9), 1722–1735.Google Scholar
Lohse, D. & Xia, K.-Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 42 (1), 335–364.Google Scholar
Maidment, D. R. 1993 Handbook of Hydrology. McGraw Hill Professional.Google Scholar
Mangarella, P. A., Chambers, A. J., Street, R. L. & Hsu, E. Y. 1973 Laboratory studies of evaporation and energy transfer through a wavy air water interface. J. Phys. Oceanogr. 3 (1), 93–101.Google Scholar
Murray, F. W. 1967 On the computation of saturation vapor pressure. J. Appl. Meteorol. 6 (1), 203–204.Google Scholar
Pauluis, O. & Schumacher, J. 2011 Self-aggregation of clouds in conditionally unstable moist convection. Proc. Natl Acad. Sci. USA 108 (31), 12623–12628.Google Scholar
Prabhakaran, P., Weiss, S., Krekhov, A., Pumir, A. & Bodenschatz, E. 2017 Can hail and rain nucleate cloud droplets? Phys. Rev. Lett. 119 (12), 128701.Google Scholar
Radko, T. 2013 Double-Diffusive Convection. Cambridge University Press.Google Scholar
Sanders, C. J. & Holman, J. P. 1972 Franz Grashof and the Grashof number. Intl J. Heat Mass Transfer. 15 (3), 562–563.Google Scholar
Schmitt, R. W. 1994 Double diffusion in oceanography. Annu. Rev. Fluid Mech. 26 (1), 255–285.Google Scholar
Schumacher, J. & Pauluis, O. 2010 Buoyancy statistics in moist turbulent Rayleigh–Bénard convection. J. Fluid Mech. 648, 509–519.Google Scholar
Shishkina, O., Stevens, R. J. A. M., Grossmann, S. & Lohse, D. 2010 Boundary layer structure in turbulent thermal convection and its consequences for the required numerical resolution. New J. Phys. 12 (7), 075022.Google Scholar
Sini, J. F., Anquetin, S. & Mestayer, P. G. 1996 Pollutant dispersion and thermal effects in urban street canyons. Atmos. Environ. 30 (15), 2659–2677.Google Scholar
Stevens, R. J. A. M., Lohse, D. & Verzicco, R. 2014 Sidewall effects in Rayleigh–Bénard convection. J. Fluid Mech. 741, 1–27.Google Scholar
Stevens, R. J. A. M., van der Poel, E. P., Grossmann, S. & Lohse, D. 2013 The unifying theory of scaling in thermal convection: the updated prefactors. J. Fluid Mech. 730, 295–308.Google Scholar
Vallis, G. K., Parker, D. J. & Tobias, S. M. 2019 A simple system for moist convection: the Rainy–Bénard model. J. Fluid Mech. 862, 162–199.Google Scholar
Wei, P., Chan, T.-S., Ni, R., Zhao, X.-Z. & Xia, K.-Q. 2014 Heat transport properties of plates with smooth and rough surfaces in turbulent thermal convection. J. Fluid Mech. 740, 28–46.Google Scholar
Weidauer, T., Pauluis, O. & Schumacher, J. 2010 Cloud patterns and mixing properties in shallow moist Rayleigh–Bénard convection. New J. Phys. 12, 105002.Google Scholar
Xia, K.-Q. 2013 Current trends and future directions in turbulent thermal convection. Theor. Appl. Mech. Lett. 3 (5), 052001.Google Scholar
Yang, Y.-T., Verzicco, R. & Lohse, D. 2018 Two-scalar turbulent Rayleigh–Bénard convection: numerical simulations and unifying theory. J. Fluid Mech. 848, 648–659.Google Scholar
Zhong, J. Q., Funfschilling, D. & Ahlers, G. 2009 Enhanced heat transport by turbulent two-phase Rayleigh–Bénard convection. Phys. Rev. Lett. 102 (12), 124501.Google Scholar