Published online by Cambridge University Press: 08 March 2016
The modal instability encountered by the incompressible flow inside a toroidal pipe is studied, for the first time, by means of linear stability analysis and direct numerical simulation (DNS). In addition to the unquestionable aesthetic appeal, the torus represents the smallest departure from the canonical straight pipe flow, at least for low curvatures. The flow is governed by only two parameters: the Reynolds number 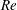 $\mathit{Re}$ and the curvature of the torus
$\mathit{Re}$ and the curvature of the torus 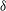 ${\it\delta}$, i.e. the ratio between pipe radius and torus radius. The absence of additional features, such as torsion in the case of a helical pipe, allows us to isolate the effect that the curvature has on the onset of the instability. Results show that the flow is linearly unstable for all curvatures investigated between 0.002 and unity, and undergoes a Hopf bifurcation at
${\it\delta}$, i.e. the ratio between pipe radius and torus radius. The absence of additional features, such as torsion in the case of a helical pipe, allows us to isolate the effect that the curvature has on the onset of the instability. Results show that the flow is linearly unstable for all curvatures investigated between 0.002 and unity, and undergoes a Hopf bifurcation at 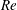 $\mathit{Re}$ of about 4000. The bifurcation is followed by the onset of a periodic regime, characterised by travelling waves with wavelength
$\mathit{Re}$ of about 4000. The bifurcation is followed by the onset of a periodic regime, characterised by travelling waves with wavelength 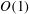 $\mathit{O}(1)$ pipe diameters. The neutral curve associated with the instability is traced in parameter space by means of a novel continuation algorithm. Tracking the bifurcation provides a complete description of the modal onset of instability as a function of the two governing parameters, and allows a precise calculation of the critical values of
$\mathit{O}(1)$ pipe diameters. The neutral curve associated with the instability is traced in parameter space by means of a novel continuation algorithm. Tracking the bifurcation provides a complete description of the modal onset of instability as a function of the two governing parameters, and allows a precise calculation of the critical values of 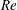 $\mathit{Re}$ and
$\mathit{Re}$ and 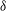 ${\it\delta}$. Several different modes are found, with differing properties and eigenfunction shapes. Some eigenmodes are observed to belong to groups with a set of common characteristics, deemed ‘families’, while others appear as ‘isolated’. Comparison with nonlinear DNS shows excellent agreement, confirming every aspect of the linear analysis, its accuracy, and proving its significance for the nonlinear flow. Experimental data from the literature are also shown to be in considerable agreement with the present results.
${\it\delta}$. Several different modes are found, with differing properties and eigenfunction shapes. Some eigenmodes are observed to belong to groups with a set of common characteristics, deemed ‘families’, while others appear as ‘isolated’. Comparison with nonlinear DNS shows excellent agreement, confirming every aspect of the linear analysis, its accuracy, and proving its significance for the nonlinear flow. Experimental data from the literature are also shown to be in considerable agreement with the present results.