Article contents
Mixing enhancement in binary fluids using optimised stirring strategies
Published online by Cambridge University Press: 24 July 2020
Abstract
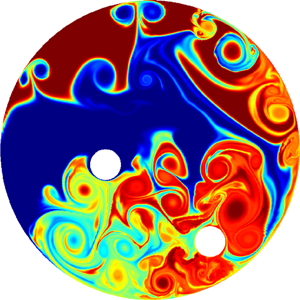
Mixing of binary fluids by moving stirrers is a commonplace process in many industrial applications, where even modest improvements in mixing efficiency could translate into considerable power savings or enhanced product quality. We propose a gradient-based nonlinear optimisation scheme to minimise the mix-norm of a passive scalar. The velocities of two cylindrical stirrers, moving on concentric circular paths inside a circular container, represent the control variables, and an iterative direct–adjoint algorithm is employed to arrive at enhanced mixing results. The associated stirring protocol is characterised by a complex interplay of vortical structures, generated and promoted by the stirrers’ action. Full convergence of the optimisation process requires constraints that penalise the acceleration of the moving bodies. Under these conditions, considerable mixing enhancement can be accomplished, even though an optimum cannot be guaranteed due to the non-convex nature of the optimisation problem. Various challenges and extensions of our approach are discussed.
JFM classification
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
REFERENCES
Eggl and Schmid supplementary movie 1
Mixing optimisation based on only energy constraints for the stirrers. The time horizon for applying control is Tcontrol = 1: Shown are iso-contours of the passive scalar. The optimisation algorithm includes information over a time window of Tinfo = 8:
Eggl and Schmid supplementary movie 2
Mixing optimisation based on only energy constraints for the stirrers. The time horizon for applying control is Tcontrol = 8: Shown are iso-contours of the passive scalar. The optimisation algorithm includes information over a time window of Tinfo = 8:
Eggl and Schmid supplementary movie 3
Mixing optimisation based on energy and velocity constraints for the stir- rers. The time horizon for applying control is Tcontrol = 1: Shown are iso-contours of the passive scalar. The optimisation algorithm includes information over a time window of Tinfo = 8:
Eggl and Schmid supplementary movie 4
Mixing optimisation based on energy and velocity constraints for the stir- rers. The time horizon for applying control is Tcontrol = 8: Shown are iso-contours of the passive scalar. The optimisation algorithm includes information over a time window of Tinfo = 8:
Eggl and Schmid supplementary movie 5
Mixing optimisation based on energy, velocity and acceleration constraints for the stirrers. The time horizon for applying control is Tcontrol = 1: Shown are iso-contours of the passive scalar. The optimisation algorithm includes information over a time window of Tinfo = 8:
Eggl and Schmid supplementary movie 6
Mixing optimisation based on energy, velocity and acceleration constraints for the stirrers. The time horizon for applying control is Tcontrol = 8: Shown are iso-contours of the passive scalar. The optimisation algorithm includes information over a time window of Tinfo = 8:
- 14
- Cited by


