Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-23T08:42:31.576Z Has data issue: false hasContentIssue false
  • English
  • Français

Longitudinal–transverse aerodynamic force in viscous compressible complex flow

Published online by Cambridge University Press:  01 September 2014

L. Q. Liu ,
Y. P. Shi ,
J. Y. Zhu ,
W. D. Su ,
S. F. Zou  and
J. Z. Wu
L. Q. Liu
Affiliation:
State Key Laboratory of Turbulence and Complex System, College of Engineering, Peking University, Beijing 100871, PR China
Y. P. Shi*
Affiliation:
State Key Laboratory of Turbulence and Complex System, College of Engineering, Peking University, Beijing 100871, PR China
J. Y. Zhu
Affiliation:
State Key Laboratory of Turbulence and Complex System, College of Engineering, Peking University, Beijing 100871, PR China
W. D. Su
Affiliation:
State Key Laboratory of Turbulence and Complex System, College of Engineering, Peking University, Beijing 100871, PR China
S. F. Zou
Affiliation:
State Key Laboratory of Turbulence and Complex System, College of Engineering, Peking University, Beijing 100871, PR China
J. Z. Wu
Affiliation:
State Key Laboratory of Turbulence and Complex System, College of Engineering, Peking University, Beijing 100871, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We report our systematic development of a general and exact theory for diagnosis of total force and moment exerted on a generic body moving and deforming in a calorically perfect gas. The total force and moment consist of a longitudinal part (L-force) due to compressibility and irreversible thermodynamics, and a transverse part (T-force) due to shearing. The latter exists in incompressible flow but is now modulated by the former. The theory represents a full extension of a unified incompressible diagnosis theory of the same type developed by J. Z. Wu and coworkers to compressible flow, with Mach number ranging from low-subsonic to moderate-supersonic flows. Combined with computational fluid dynamics (CFD) simulation, the theory permits quantitative identification of various complex flow structures and processes responsible for the forces, and thereby enables rational optimal configuration design and flow control. The theory is confirmed by a numerical simulation of circular-cylinder flow in the range of free-stream Mach number $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}M_{\infty }$ between 0.2 and 2.0. The L-drag and T-drag of the cylinder vary with 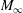$M_{\infty }$ in different ways, the underlying physical mechanisms of which are analysed. Moreover, each L-force and T-force integrand contains a universal factor of local Mach number 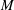$M$. Our preliminary tests suggest that the possibility of finding new similarity rules for each force constituent could be quite promising.

JFM classification

Aerodynamics: Aerodynamics Compressible Flows: Compressible Flows Vortex Flows: Vortex Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 756 , 10 October 2014 , pp. 226 - 251
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Burgers, J. M. 1921 On the resistance of fluids and vortex motion. Koninklijke Nederlandse Akademie van Wetenschappen Proceedings Series B Physical Sciences 23, 774–782.Google Scholar
Chang, C. C. & Lei, S. Y. 1996 On the sources of aerodynamic forces: steady flow around a cylinder or a sphere. Proc. R. Soc. Lond. A 452, 2369–2395.Google Scholar
Chang, C. C., Su, J. Y. & Lei, S. Y. 1998 On aerodynamic forces for viscous compressible flow. Theor. Comput. Fluid Dyn. 10, 71–90.Google Scholar
Chu, B. T. & Kovásznay, L. S. G. 1958 Non-linear interactions in a viscous heat conducting compressible gas. J. Fluid Mech. 3, 494–514.Google Scholar
Fiabane, L., Gohlke, M. M. & Cadot, O. 2011 Characterization of flow contributions to drag and lift of a circular cylinder using a volume expression of the fluid force. Eur. J. Mech. (B/Fluids) 30, 311–315.Google Scholar
Gilbarg, D. & Paolucci, D. 1953 The structure of shock waves in the continuum theory of fluids. J. Rat. Mech. Anal. 2, 617–642.Google Scholar
Huang, G. C. 1994 Unsteady Vortical Aerodynamics: Theory and Applications. Shanghai Jiaotong University Press, (in Chinese).Google Scholar
von Kármán, Th. 1941 Compressibility effects in aerodynamics. J. Aero. Sci. 8, 337–356.Google Scholar
von Kármán, Th. & Sears, W. R. 1938 Airfoil theory for non-uniform motion. J. Aeronaut. Sci. 5, 379–390.Google Scholar
Kovásznay, L. S. G. 1953 Turbulence in supersonic flow. J. Aero. Sci. 20, 657–674.Google Scholar
Lagerstrom, P. A. 1964 Laminar Flow Theory. Princeton University Press.Google Scholar
Lagerstrom, P. A., Cole, J. D. & Trilling, L.1948 Problems in the theory of viscous compressible fluids. GALCIT Tech Rep. 6.Google Scholar
Li, G. J. & Lu, X. Y. 2012 Force and power of flapping plates in a fluid. J. Fluid Mech. 712, 598–613.Google Scholar
Liu, L. Q., Wu, J. Z., Shi, Y. P. & Zhu, J. Y. 2014 A dynamic counterpart of Lamb vector in viscous compressible aerodynamics. Fluid Dyn. Res. (in press).Google Scholar
Luo, Y. B.2004 Boundary vorticity dynamics, derivative moment theory, and their applications in flow diagnosis and control. Master thesis, Peking University.Google Scholar
Mao, F., Shi, Y. P. & Wu, J. Z. 2010 On a general theory for compressing process and aeroacoustics: linear analysis. Acta Mechanica Sin. 26, 355–364.CrossRefGoogle Scholar
Mao, F., Shi, Y. P., Xuan, L. J., Su, W. D. & Wu, J. Z. 2011 On the governing equations for the compressing process and its coupling with other processes. Sci. China. Phys. Mech. Astron. 54, 1154–1167.Google Scholar
Marongiu, C. & Tognaccini, R. 2010 Far-field analysis of the aerodynamic force by Lamb vector integrals. AIAA J. 48, 2543–2555.Google Scholar
Marongiu, C., Tognaccini, R. & Ueno, M. 2013 Lift and lift-induced drag computation by Lamb vector integration. AIAA J. 51, 1420–1430.Google Scholar
McCune, J. E. & Tavares, T. S. 1993 Perspective: unsteady wing theory—the Kármán/Sears legacy. J. Fluids Engng 115, 548–560.Google Scholar
Mele, B. & Tognaccini, R. 2014 Aerodynamic force by Lamb vector integrals in compressible flow. Phys. Fluids 26, 056104.CrossRefGoogle Scholar
Oswatitsch, K. 1956 Gas Dynamics. Academic.Google Scholar
Pierce, A. D. 1989 Acoustics: An Introduction to its Physical Principles and Applications. Acoustical Society of America.Google Scholar
Prandtl, L.1918 Nachrichten von der Gesellschaft der Wissenschaften zu Göttingen, Mathematisch-Physikalische Klasse. Tragflügeltheorie. I. Mitteilung, pp. 451–477.Google Scholar
Saffman, P. G. 1992 Vortex Dynamics. Cambridge University Press.Google Scholar
Sherman, F. S.1955 A low-density wind-tunnel study of shock structure and relaxation phenomena in gases. NACA Tech Rep. 3298.Google Scholar
Wu, J. C. 1981 Theory for aerodynamic force and moment in viscous flows. AIAA J. 19, 432–441.Google Scholar
Wu, J. Z., Lu, X. Y., Yang, Y. T. & Zhang, R. K. 2010 Vorticity dynamics in complex flow diagnosis and management. Pei-Yuan Chou memorial lecture. In Proceedings 13th Asian Congr. Fluid Mech. Bangladesh Society of Mechanical Engineering, Dhaka (ed. Sadrul Islam, A. K. M.), pp. 19–38.Google Scholar
Wu, J. Z., Lu, X. Y. & Zhuang, L. X. 2007 Integral force acting on a body due to local flow structures. J. Fluid Mech. 576, 265–286.CrossRefGoogle Scholar
Wu, J. Z., Ma, H. Y. & Zhou, M. D. 2006 Vorticity and Vortex Dynamics. Springer.Google Scholar
Wu, J. Z. & Wu, J. M. 1993 Interactions between a solid surface and a viscous compressible flow field. J. Fluid Mech. 254, 183–211.Google Scholar
Wu, J. Z. & Wu, J. M. 1996 Vorticity dynamics on boundaries. Adv. Appl. Mech. 32, 19–275.Google Scholar
Wu, J. Z., Wu, H. & Li, Q. S. 2009 Boundary vorticity flux and engineering flow management. Adv. Appl. Math. Mech. 1, 353–366.Google Scholar
Wu, J. Z., Wu, J. M. & Wu, C. J. 1988 A viscous compressible flow theory on the interaction between moving bodies and flow field in the $(\boldsymbol{\omega}, \vartheta )$ framework. Fluid Dyn. Res. 3, 203–208.Google Scholar
Wu, T. Y. 1956 Small perturbations in the unsteady flow of a compressible, viscous and heat-conducting fluid. J. Math. Phys. 35, 13–27.Google Scholar
Wu, T. Y. 2007 A nonlinear theory for a flexible unsteady wing. J. Engng Maths 58, 279–287.Google Scholar
Xu, C. Y., Chen, L. W. & Lu, X. Y. 2009 Effect of mach number on transonic flow past a circular cylinder. Chin. Sci. Bull. 54, 1886–1893.Google Scholar
Xu, C. Y., Chen, L. W. & Lu, X. Y. 2010 Large-eddy simulation of the compressible flow past a wavy cylinder. J. Fluid Mech. 665, 238–273.Google Scholar
Yang, Y. T., Zhang, R. K., An, Y. R. & Wu, J. Z. 2007 Steady vortex force theory and slender-wing flow diagnosis. Acta Mechanica Sin. 23, 609–619.Google Scholar