Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-09T20:35:34.322Z Has data issue: false hasContentIssue false
  • English
  • Français

Large eddy simulation of the velocity-intermittency structure for flow over a field of symmetric dunes

Published online by Cambridge University Press:  23 September 2016

Christopher J. Keylock ,
Kyoungsik S. Chang  and
George S. Constantinescu
Christopher J. Keylock*
Affiliation:
Sheffield Fluid Mechanics Group and Department of Civil and Structural Engineering, University of Sheffield, Mappin Street, Sheffield S1 3JD, UK
Kyoungsik S. Chang
Affiliation:
Department of Aeromechanical Engineering, Hanseo University, South Korea
George S. Constantinescu
Affiliation:
Department of Civil and Environmental Engineering, IIHR-Hydroscience and Engineering, University of Iowa, Stanley Hydraulics Laboratory, Iowa City, IA 52242, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Owing to their frequent occurrence in the natural environment, there has been significant interest in refining our understanding of flow over dunes and other bedforms. Recent work in this area has focused, in particular, on their shear-layer characteristics and the manner by which flow structures are generated. However, field-based studies, are reliant on single-, or multi-point measurements, rather than delimiting flow structures from the velocity gradient tensor, as is possible in numerical work. Here, we extract pointwise time series from a well-resolved large eddy simulation as a means to connect these two approaches. The at-a-point analysis technique is termed the velocity-intermittency quadrant method and relates the fluctuating, longitudinal velocity, $u_{1}^{\prime }(t)$, to its fluctuating pointwise Hölder regularity, 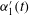$\unicode[STIX]{x1D6FC}_{1}^{\prime }(t)$. Despite the difference in boundary conditions, our results agree very well with previous experiments that show the importance, in the region above the dunes, of a quadrant 3 (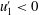$u_{1}^{\prime }<0$, 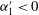$\unicode[STIX]{x1D6FC}_{1}^{\prime }<0$) flow configuration. Our higher density of sampling beneath the shear layer and close to the bedforms relative to experimental work reveals a negative correlation between 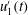$u_{1}^{\prime }(t)$ and 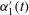$\unicode[STIX]{x1D6FC}_{1}^{\prime }(t)$ in this region. 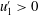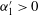This consists of two distinct layers, with quadrant 4 ($u_{1}^{\prime }>0$, $\unicode[STIX]{x1D6FC}_{1}^{\prime }<0$) dominant near the wall and quadrant 2 ($u_{1}^{\prime }<0$, $\unicode[STIX]{x1D6FC}_{1}^{\prime }>0$) dominant close to the lower part of the separated shear layer. These results are consistent with a near-wall advection of vorticity into a region downstream of a temporarily foreshortened reattachment region, and the entrainment of slow moving and quiescent fluid into a faster, more turbulent shear layer. A comparison of instantaneous vorticity fields to the velocity-intermittency analysis shows how the pointwise results reflect larger-scale organisation of the flow. We illustrate this using results from two instantaneous datasets. In the former, extreme velocity-intermittency events corresponding to a foreshortened recirculation region (and high pressures on the stoss slope of the dune immediately downstream) arise, and the development of intense flow structures occurs as a consequence. In the other case, development of a ‘skimming flow’ with relatively little exchange between the inner and outer regions results in exceedances because of the coherence associated with this high velocity, high turbulence outer region. Thus, our results shed further light on the characteristics of dune flow in the near-wall region and, importantly for field-based research, show that useful information on flow structure can be obtained from single-point single velocity component measurements.

JFM classification

Turbulent Flows: Intermittency Geophysical and Geological Flows: River dynamics Vortex Flows: Vortex dynamics
Type
Papers
Information
Journal of Fluid Mechanics , Volume 805 , 25 October 2016 , pp. 656 - 685
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Arnéodo, A., Manneville, S., Muzy, J. F. & Roux, S. G. 1999 Revealing a lognormal cascading process in turbulent velocity statistics with wavelet analysis. Phil. Trans. R. Soc. Lond. A 357, 2415–2438.CrossRefGoogle Scholar
Babakaiff, S. C. & Hickin, E. J. 1996 Coherent flow structures in Squamish River Estuary, British Columbia, Canada. In Coherent Flow Structures in Open Channels (ed. Ashworth, P., Bennett, S., Best, J. L. & McLelland, S. J.), pp. 321–342. Wiley.Google Scholar
Basu, S., Foufoula-Georgiou, E. & Porte-Agel, F. 2004 Synthetic turbulence, fractal interpolation, and large-eddy simulation. Phys. Rev. E 70 (2), 026310.Google Scholar
Bennett, S. J. & Best, J. L. 1995 Mean flow and turbulence structure over fixed, two-dimensional dunes: implications for sediment transport and dune stability. Sedimentology 42, 491–513.CrossRefGoogle Scholar
Best, J. 2005 The fluid dynamics of river dunes: a review and some future research directions. J. Geophys. Res. 110, F04S02.Google Scholar
Bogard, D. G. & Tiederman, W. G. 1986 Burst detection with single-point velocity measurements. J. Fluid Mech. 162, 389–413.Google Scholar
Castro, I. P. & Haque, A. 1987 The structure of a turbulent shear layer bounding a separation region. J. Fluid Mech. 179, 439–468.CrossRefGoogle Scholar
Chang, K. & Constantinescu, G. 2013 Coherent structures in flow over two-dimensional dunes. Water Resour. Res. 49, 2446–2460.Google Scholar
Chang, K. & Constantinescu, G. 2015 Numerical investigation of flow and turbulence structure through and around a circular array of rigid cylinders. J. Fluid Mech. 776, 161–199.CrossRefGoogle Scholar
Chang, K., Constantinescu, G. & Park, S. O. 2006 Analysis of the flow and mass transfer process for the incompressible flow past an open cavity with a laminar and a fully turbulent incoming boundary layer. J. Fluid Mech. 561, 113–145.CrossRefGoogle Scholar
Chang, K., Constantinescu, G. & Park, S. O. 2007 The purging of a neutrally buoyant or a dense miscible contaminant from a rectangular cavity. Part II. The case of an incoming fully turbulent overflow. J. Hydraul. Engng 133, 373–385.CrossRefGoogle Scholar
Cherukat, P., Na, Y. & Hanratty, T. J. 1998 Direct numerical simulation of a fully developed turbulent flow over a wavy wall. Theor. Comput. Fluid Dyn. 11, 109–134.Google Scholar
Christensen, K. T. & Adrian, R. J. 2001 Statistical evidence of hairpin vortex packets in wall turbulence. J. Fluid Mech. 431, 433–443.Google Scholar
Dumas, S., Arnott, R. W. C. & Southard, J. B. 2005 Experiments on oscillatory-flow and combined-flow bed forms: implications for interpreting parts of the shallow-marine sedimentary record. J. Sedim. Res. 75, 501–513.Google Scholar
Fourrière, A., Claudin, P. & Andreotti, B. 2010 Bedforms in a turbulent stream: formation of ripples by primary linear instability and of dunes by nonlinear pattern coarsening. J. Fluid Mech. 649, 287–328.Google Scholar
Frisch, U., Bec, J. & Aurell, F. 2005 ‘Locally homogeneous turbulence’: is it an inconsistent framework? Phys. Fluids 17, 081706.CrossRefGoogle Scholar
Frisch, U. & Parisi, G. 1985 The singularity structure of fully developed turbulence. In Turbulence and Predictability in Geophysical Fluid Dynamics and Climate Dynamics (ed. Ghil, M., Benzi, R. & Parisi, G.), pp. 84–88. North Holland.Google Scholar
Frisch, U., Sulem, P. L. & Nelkin, M. 1978 Simple dynamical model of intermittent fully developed turbulence. J. Fluid Mech. 87, 719–736.Google Scholar
Furuichi, N., Hachiga, T. & Kumada, M. 2004 An experimental investigation of a large-scale structure of a two-dimensional backward-facing step by using advanced multipoint LDV. Exp. Fluids 36, 274–281.CrossRefGoogle Scholar
Ganapathisubramani, B., Longmire, E. K. & Marusic, I. 2003 Characteristics of vortex packets in turbulent boundary layers. J. Fluid Mech. 478, 35–46.Google Scholar
Germano, M. 1992 Turbulence: the filtering approach. J. Fluid Mech. 238, 325–336.Google Scholar
Geurts, B. J. 2003 Elements of Direct and Large-eddy Simulation, R. T. Edwards.Google Scholar
Grigoriadis, D. G. E., Balaras, E. & Dimas, A. A. 2009 Large-eddy simulation of unidirectional water flow over dunes. J. Geophys. Res. 114, F02022.Google Scholar
Günther, A. & von Rohr, P. R. 2003 Large scale structures in a developed flow over a wavy wall. J. Fluid Mech. 478, 257–285.Google Scholar
Horiuti, K., Yanagihara, S. & Tamaki, T. 2016 Nonequilibrium state in energy spectra and transfer with implications for topological transition and SGS modelling. Fluid Dyn. Res 48 (2), 021409.Google Scholar
Hosokawa, I. 2007 A paradox concerning the refined similarity hypothesis of Kolmogorov for isotropic turbulence. Progr. Theor. Phys. 118, 169–173.Google Scholar
Hudson, J. D., Dykhno, L. & Hanratty, T. J. 1996 Turbulence production in flow over a wavy wall. Exp. Fluids 20, 257–265.Google Scholar
Hunt, J. C. R., Wray, A. A. & Moin, P.1988 Eddies, stream, and convergence zones in turbulent flows. Report No. CTR-S88. Center for Turbulence Research, Stanford University, USA.Google Scholar
Hurther, D., Lemmin, U. & Terray, E. A. 2007 Turbulent transport in the outer region of rough-wall open-channel flows: the contribution of large coherent shear stress structures (LC3S). J. Fluid Mech. 574, 465–493.Google Scholar
Jackson, R. G. 1976 Sedimentological and fluid-dynamic implications of the turbulent bursting phenomenon in geophysical flows. J. Fluid Mech. 77, 531–560.Google Scholar
Jaffard, S. 1997 Multifractal formalism for functions. 1. Results valid for all functions. SIAM J. Math. Anal. 28, 944–970.CrossRefGoogle Scholar
Keylock, C. J. 2008 A criterion for delimiting active periods within turbulent flows. Geophys. Res. Lett. 35, L11804.Google Scholar
Keylock, C. J. 2010 Characterizing the structure of nonlinear systems using gradual wavelet reconstruction. Nonlinear Process. Geophys. 17, 615–632.Google Scholar
Keylock, C. J. 2015 Flow resistance in natural, turbulent channel flows: the need for a fluvial fluid mechanics. Water Resour. Res. 51, 4374–4390.Google Scholar
Keylock, C. J., Ganapathasubramani, B., Monty, J., Hutchins, N. & Marusic, I. 2016a The coupling between inner and outer scales in a zero pressure boundary layer evaluated using a Hölder exponent framework. Fluid Dyn. Res. 48 (2), 021405.Google Scholar
Keylock, C. J., Kida, S. & Peters, N. 2016b JSPS supported symposium on interscale transfers and flow topology in equilibrium and non-equilibrium turbulence (Sheffield, UK, September 2014). Fluid Dyn. Res. 48 (2), 020001.Google Scholar
Keylock, C. J., Lane, S. N. & Richards, K. S. 2014a Quadrant/octant sequencing and the role of coherent structures in bed load sediment entrainment. J. Geophys. Res. 119, 264–286.Google Scholar
Keylock, C. J., Nishimura, K. & Peinke, J. 2012 A classification scheme for turbulence based on the velocity-intermittency structure with an application to near-wall flow and with implications for bedload transport. J. Geophys. Res. 117, F01037.Google Scholar
Keylock, C. J., Singh, A. & Foufoula-Georgiou, E. 2013 The influence of bedforms on the velocity-intermittency structure of turbulent flow over a gravel bed. Geophys. Res. Lett. 40 (1–5), 1351–1355.Google Scholar
Keylock, C. J., Singh, A., Venditti, J. G. & Foufoula-Georgiou, E. 2014b Robust classification for the joint velocity-intermittency structure of turbulent flow over fixed and mobile bedforms. Earth Surf. Process. Landf. 39, 1717–1728.Google Scholar
Keylock, C. J., Stresing, R. & Peinke, J. 2015 Gradual wavelet reconstruction of the velocity increments for turbulent wakes. Phys. Fluids 27, 025104.Google Scholar
Kolmogorov, A. N. 1941 The local structure of turbulence in incompressible viscous fluid for very large Reynolds numbers. Dokl. Akad. Nauk SSSR 30, 299–303.Google Scholar
Kolmogorov, A. N. 1962 A refinement of previous hypotheses concerning the local structure of turbulence in a viscous, incompressible fluid at high Reynolds number. J. Fluid Mech. 13, 82–85.Google Scholar
Kolwankar, K. M. & Lévy Véhel, J. 2002 A time domain characterisation of the fine local regularity of functions. J. Fourier Anal. Applics. 8, 319–334.Google Scholar
Kostaschuk, R. 2000 A field study of turbulence and sediment dynamics over subaqueous dunes with flow separation. Sedimentology 47, 519–531.CrossRefGoogle Scholar
Kostaschuk, R. & Villard, P. 1996 Flow and sediment transport over large subaqueous dunes: Fraser River, Canada. Sedimentology 43, 849–863.Google Scholar
Kruse, N., Günther, A. & von Rohr, P. R. 2003 Dynamics of large-scale structures in turbulent flow over a wavy wall. J. Fluid Mech. 485, 87–96.Google Scholar
Lancaster, N., Nickling, W. G., Neuman, C. K. M. & Wyatt, V. E. 1996 Sediment flux and airflow on the stoss slope of a barchan dune. Geomorphology 17, 55–62.Google Scholar
Lu, S. S & Willmarth, W. W. 1973 Measurements of the structure of the Reynolds stress in a turbulent boundary layer. J. Fluid Mech. 60, 481–511.Google Scholar
Mahesh, K., Constantinescu, S. G. & Moin, P. 2004 A numerical method for large eddy simulation in complex geometries. J. Comput. Phys. 197, 215–240.Google Scholar
Meneveau, C. 2012 Germano identity-based subgrid scale modeling: a brief survey of variations on a fertile theme. Phys. Fluids 24, 121301.Google Scholar
Meneveau, C. & Sreenivasan, K. 1991 The multifractal nature of turbulent energy-dissipation. J. Fluid Mech. 224, 429–484.Google Scholar
Muzy, J. F., Bacry, E. & Arnéodo, A. 1991 Wavelets and multifractal formalism for singular signals: application to turbulence data. Phys. Rev. Lett. 67, 3515–3518.Google Scholar
Nakagawa, H. & Nezu, I. 1977 Prediction of the contributions to the Reynolds stress from bursting events in open channel flows. J. Fluid Mech. 80, 99–128.Google Scholar
Nelson, J. M., McLean, S. R. & Wolfe, S. R. 1993 Mean flow and turbulence fields over 2-dimensional bed forms. Water Resour. Res. 29, 3935–3953.Google Scholar
Omidyeganeh, M. & Piomelli, U. 2011 Large-eddy simulation of two-dimensional dunes in a steady, unidirectional flow. J. Turbul. 12 (42), 1–31.Google Scholar
Omidyeganeh, M. & Piomelli, U. 2013a Large-eddy simulation of three-dimensional dunes in a steady, unidirectional flow. Part 1. Turbulence statistics. J. Fluid Mech. 721, 454–483.Google Scholar
Omidyeganeh, M. & Piomelli, U. 2013b Large-eddy simulation of three-dimensional dunes in a steady, unidirectional flow. Part 2. Flow structures. J. Fluid Mech. 734, 509–534.Google Scholar
Osborne, P. D. & Rooker, G. A. 1999 Sand re-suspension events in a high energy infragravity swash zone. J. Coast. Res. 15, 74–86.Google Scholar
Parsons, D. R., Best, J. L., Orfeo, O., Hardy, R. J., Kostaschuk, R. & Lane, S. N. 2005 Morphology and flow fields of three-dimensional dunes, Rio Parana, Argentina: results from simultaneous multibeam echo sounding and acoustic Doppler current profiling. J. Geophys. Res. 110, F04S03.Google Scholar
Parsons, D. R., Walker, I. J. & Wiggs, G. F. S. 2004 Numerical modelling of flow structures over idealized transverse aeolian dunes of varying geometry. Geomorphology 59, 149–164.Google Scholar
Pierce, C. D. & Moin, P.2001 Progress-variable approach for large eddy simulation of turbulent combustion. Tech. Rep. TF-80. Mech. Eng. Dep., Stanford University, USA.Google Scholar
Praskovsky, A. A., Gledzer, E. B., Karyakin, M. Y. & Zhou, Y. 1993 The sweeping decorrelation hypothesis and energy-inertial scale interaction in high Reynolds number flow. J. Fluid Mech. 248, 493–511.Google Scholar
Renner, C., Peinke, J. & Friedrich, R. 2001 Experimental indications for Markov properties of small-scale turbulence. J. Fluid Mech. 433, 383–409.Google Scholar
Richardson, L. F. 1922 Weather Prediction by Numerical Process. Cambridge University Press.Google Scholar
Robinson, S. K. 1991 Coherent motions in the turbulent boundary layer. Annu. Rev. Fluid Mech. 23, 601–639.Google Scholar
Sagaut, P. 2002 Large Eddy Simulation for Incompressible Flows. Springer.Google Scholar
Salvetti, M. V., Damiani, R. & Beuxm, F. 2001 Three-dimensional, coarse large-eddy simulations of the flow above two-dimensional sinusoidal waves. Intl J. Numer. Meth. Fluids 35, 617–642.Google Scholar
Scotti, A. & Meneveau, C. 1999 A fractal model for large eddy simulation of turbulent flow. Physica D 127, 198–232.Google Scholar
Seuret, S. & Lévy Véhel, J. 2003 A time domain characterization of 2-microlocal spaces. J. Fourier Anal. Applics. 9, 473–495.Google Scholar
She, Z. S. & Leveque, E. 1994 Universal scaling laws in fully developed turbulence. Phys. Rev. Lett. 72, 336–339.Google Scholar
Shugar, D. H., Kostaschuk, R., Best, J. L., Parsons, D. R., Lane, S. N., Orfeo, O. & Hardy, R. J. 2010 On the relationship between flow and suspended sediment transport over the crest of a sand dune, Rio Parana, Argentina. Sedimentology 57, 252–272.Google Scholar
Singh, A., Fienberg, K., Jerolmack, D., Marr, J. & Foufoula-Georgiou, E. 2009 Experimental evidence for statistical scaling and intermittency in sediment transport rates. J. Geophys. Res. 114, F01025.Google Scholar
Singh, A., Porté-Agel, F. & Foufoula-Georgiou, E. 2010 On the influence of gravel bed dynamics on velocity power spectra. Water Resour. Res. 46, W04509.Google Scholar
Stoesser, T., Braun, C., Garcia-Villalba, M. & Rodi, W. 2008 Turbulent structures in flow over two dimensional dunes. J. Hydraul. Engng 143, 42–55.Google Scholar
Stresing, R. & Peinke, J. 2010 Towards a stochastic multi-point description of turbulence. New J. Phys. 12, 103046.Google Scholar
Stresing, R., Peinke, J., Seoud, S. & Vassilicos, J. 2010 Defining a new class of turbulent flows. Phys. Rev. Lett. 104, 194501.Google Scholar
Titus, T., Zimbelman, J. & Radebaugh, J. 2015 The importance of dunes on a variety of planetary surfaces. Eos 96 (19), 4.Google Scholar
Vassilicos, J. C. 2015 Dissipation in turbulent flows. Annu. Rev. Fluid Mech. 47, 95–114.Google Scholar
Venditti, J. G. & Bennett, S. J. 2000 Spectral analysis of turbulent flow and suspended sediment transport over fixed dunes. J. Geophys. Res. 105 (C9), 22035–22047.Google Scholar
Wiggs, G. F. S., Livingstone, I. & Warren, A. 1996 The role of streamline curvature in sand dune dynamics: evidence from field and wind tunnel measurements. Geomorphology 17, 29–46.CrossRefGoogle Scholar
Zedler, E. A. & Street, R. L. 2001 Large-eddy simulation of sediment transport: currents over ripples. J. Hydraul. Engng 127, 444–452.Google Scholar