Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-16T15:21:07.036Z Has data issue: false hasContentIssue false
  • English
  • Français

Improved scaling laws for the shock-induced dispersal of a dense particle curtain

Published online by Cambridge University Press:  08 August 2019

Edward P. DeMauro Open the ORCID record for Edward P. DeMauro [Opens in a new window] ,
Justin L. Wagner Open the ORCID record for Justin L. Wagner [Opens in a new window] ,
Lawrence J. DeChant ,
Steven J. Beresh  and
Aaron M. Turpin
Edward P. DeMauro*
Affiliation:
Rutgers, The State University of New Jersey, Department of Mechanical and Aerospace Engineering, 98 Brett Road, Room D102, Piscataway, NJ 08854, USA
Justin L. Wagner
Affiliation:
Sandia National Laboratories, Engineering Sciences Center, P.O. Box 5800, MS-0825, Albuquerque, NM 87185, USA
Lawrence J. DeChant
Affiliation:
Sandia National Laboratories, Engineering Sciences Center, P.O. Box 5800, MS-0825, Albuquerque, NM 87185, USA
Steven J. Beresh
Affiliation:
Sandia National Laboratories, Engineering Sciences Center, P.O. Box 5800, MS-0825, Albuquerque, NM 87185, USA
Aaron M. Turpin
Affiliation:
North Carolina State University, Department of Mechanical and Aerospace Engineering, Raleigh, NC 27695, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Experiments were performed within Sandia National Labs’ Multiphase Shock Tube to measure and quantify the shock-induced dispersal of a shock/dense particle curtain interaction. Following interaction with a planar travelling shock wave, schlieren imaging at 75 kHz was used to track the upstream and downstream edges of the curtain. Data were obtained for two particle diameter ranges (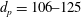$d_{p}=106{-}125$, 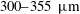$300{-}355~\unicode[STIX]{x03BC}\text{m}$) across Mach numbers ranging from 1.24 to 2.02. Using these data, along with data compiled from the literature, the dispersion of a dense curtain was studied for multiple Mach numbers (1.2–2.6), particle sizes (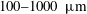$100{-}1000~\unicode[STIX]{x03BC}\text{m}$) and volume fractions (9–32 %). Data were non-dimensionalized according to two different scaling methods found within the literature, with time scales defined based on either particle propagation time or pressure ratio across a reflected shock. The data show that spreading of the particle curtain is a function of the volume fraction, with the effectiveness of each time scale based on the proximity of a given curtain’s volume fraction to the dilute mixture regime. It is seen that volume fraction corrections applied to a traditional particle propagation time scale result in the best collapse of the data between the two time scales tested here. In addition, a constant-thickness regime has been identified, which has not been noted within previous literature.

JFM classification

Granular media: Granular media Compressible Flows: Shock waves Multiphase and Particle-laden Flows: Particle/fluid flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 876 , 10 October 2019 , pp. 881 - 895
Creative Commons
This is a work of the U.S. Government and is not subject to copyright protection in the United States.
Copyright
© 2019 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Akiki, G., Jackson, T. L. & Balachandar, S. 2017 Pairwise interaction extended point-particle model for a random array of monodisperse spheres. J. Fluid Mech. 813, 882–928.Google Scholar
Boiko, V. M., Kiselev, V. P., Kiselev, S. P., Papyrin, A. N., Poplavsky, S. V. & Formin, V. M. 1997 Shock wave interaction with a cloud of particles. Shock Waves 7, 275–285.Google Scholar
Chang, E. J. & Kailasanath, K. 2003 Shock wave interactions with particles and liquid fuel droplets. Shock Waves 12, 333–341.Google Scholar
Davis, S. L., Dittmann, T. B., Jacobs, G. B. & Don, W. S. 2013 Dispersion of a cloud of particles by a moving shock: effects of the shape, angle of rotation, and aspect ratio. J. Appl. Mech. Tech. Phys. 54 (4), 900–912.Google Scholar
DeMauro, E. P., Wagner, J. L., Beresh, S. J. & Farias, P. A. 2017 Unsteady drag following shock wave impingement on a particle curtain measured using pulse-burst PIV. Phys. Rev. Fluids 2, 064301.Google Scholar
Goetsch, R. J. & Regele, J. D. 2015 Discrete element method prediction of particle curtain properties. Chem. Engng Sci. 137, 852–861.Google Scholar
Houim, R. W. & Oran, E. S. 2016 A multiphase model for compressible granular-gaseous flows: formulation and initial tests. J. Fluid Mech. 789, 166–220.Google Scholar
Kellenberger, M., Johansen, C., Ciccarelli, G. & Zhang, F. 2013 Dense particle cloud dispersion by a shock wave. Shock Waves 23 (5), 415–430.Google Scholar
Kosinski, P. 2008 Numerical investigation of explosion suppression by inert particles in straight ducts. J. Hazard. Mater. 154, 981–991.Google Scholar
Ling, Y., Wagner, J. L., Beresh, S. J., Kearney, S. P. & Balachandar, S. 2012 Interaction of a planar shock wave with a dense particle curtain: modeling and experiments. Phys. Fluids 24, 113301.Google Scholar
Lv, H., Wang, Z., Zhang, Y. & Li, J. 2018 Shock attenuation by densely packed micro-particle wall. Exp. Fluids 59, 140–148.Google Scholar
McFarland, J. A., Black, W. J., Dahal, J. & Morgan, B. E. 2016 Computational study of the shock driven instability of a multiphase particle-gas system. Phys. Fluids 28, 024105.Google Scholar
Merzkirch, W. & Bracht, K. 1978 The erosion of dust by a shock wave in air: initial stages with laminar flow. Intl J. Multiphase Flow 41 (1), 89–95.Google Scholar
Pinker, R. A. & Herbert, M. V. 1967 Pressure loss associated with compressible flow through square-mesh wire gauzes. J. Mech. Engng Sci. 9 (1), 11–23.Google Scholar
Regele, J. D., Rabinovitch, J., Colonius, T. & Blanquart, G. 2014 Unsteady effects in dense, high speed, particle laden flows. Multiphase Flow 61, 1–13.Google Scholar
Rogue, X., Rodriguez, G., Haas, J. F. & Saurel, R. 1998 Experimental and numerical investigation of the shock-induced fluidization of a particles bed. Shock Waves 8 (1), 29–45.Google Scholar
Sen, O., Gaul, N. J., Choi, K. K., Jacobs, G. & Udaykumar, H. S. 2017 Evaluation of kriging based surrogate models constructed from mesoscale computations of shock interaction with particles. J. Comput. Phys. 336, 235–260.Google Scholar
Sen, O., Gaul, N. J., Choi, K. K., Jacobs, G. & Udaykumar, H. S. 2018 Evaluation of multifidelity surrogate modeling techniques to construct closure laws for drag in shock-particle interactions. J. Comput. Phys. 371, 434–451.Google Scholar
Sweeney, M. R. & Valentine, G. A. 2017 Impact zone dynamics of dilute mono- and polydisperse jets and their implications for the initial conditions of pyroclastic density currents. Phys. Fluids 29, 093304.Google Scholar
Theofanous, T. G., Mitkin, V. & Chang, C. H. 2016 The dynamics of dense particle clouds subjected to shock waves. Part 1. Experiments and scaling laws. J. Fluid Mech. 792, 658–681.Google Scholar
Theofanous, T. G., Mitkin, V. & Chang, C. H. 2018 Shock dispersal of dilute particle clouds. J. Fluid Mech. 841, 732–745.Google Scholar
Vessiere, B. 2006 Detonations in gas-particle mixtures. J. Propul. Power 22 (6), 1269–1288.Google Scholar
Vorobieff, P., Anderson, M., Conroy, J., White, R., Truman, C. R. & Kumar, S. 2011 Vortex formation in a shock-accelerated gas induced by particle seeding. Phys. Rev. Lett. 106, 184503.Google Scholar
Wagner, J. L., Beresh, S. J., Kearney, S. P., Trott, W. M., Castaneda, J. N., Pruett, B. O. & Baer, M. R. 2012 A multiphase shock tube for shock wave interactions with dense particle fields. Exp. Fluids 52 (6), 1507–1517.Google Scholar
Wagner, J. L., DeMauro, E. P., Casper, K. M., Beresh, S. J., Lynch, K. P. & Pruett, B. O. 2018 Pulse-burst PIV of an impulsively started cylinder in a shock tube for Re > 105 . Exp. Fluids 59 (6), 2, 106–121.+105+.+Exp.+Fluids+59+(6),+2,+106–121.>Google Scholar
Wagner, J. L., Kearney, S. P., Beresh, S. J., DeMauro, E. P. & Pruett, B. O. 2015 Flash X-ray measurements on the shock-induced dispersal of a dense particle curtain. Exp. Fluids 56 (213), 1–12.Google Scholar
Zhang, F., Frost, D. L., Thibault, P. A. & Murray, S. B. 2001 Explosive dispersal of solid particles. Shock Waves 10, 431–443.Google Scholar