Published online by Cambridge University Press: 05 August 2014
Consider the space of sequences of 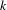 $k$ letters ordered lexicographically. We study the set
$k$ letters ordered lexicographically. We study the set 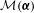 ${\mathcal{M}}(\boldsymbol{{\it\alpha}})$ of all maximal sequences for which the asymptotic proportions
${\mathcal{M}}(\boldsymbol{{\it\alpha}})$ of all maximal sequences for which the asymptotic proportions  $\boldsymbol{{\it\alpha}}$ of the letters are prescribed, where a sequence is said to be maximal if it is at least as great as all of its tails. The infimum of
$\boldsymbol{{\it\alpha}}$ of the letters are prescribed, where a sequence is said to be maximal if it is at least as great as all of its tails. The infimum of 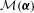 ${\mathcal{M}}(\boldsymbol{{\it\alpha}})$ is called the
${\mathcal{M}}(\boldsymbol{{\it\alpha}})$ is called the  $\boldsymbol{{\it\alpha}}$-infimax sequence, or the
$\boldsymbol{{\it\alpha}}$-infimax sequence, or the  $\boldsymbol{{\it\alpha}}$-minimax sequence if the infimum is a minimum. We give an algorithm which yields all infimax sequences, and show that the infimax is not a minimax if and only if it is the
$\boldsymbol{{\it\alpha}}$-minimax sequence if the infimum is a minimum. We give an algorithm which yields all infimax sequences, and show that the infimax is not a minimax if and only if it is the  $\boldsymbol{{\it\alpha}}$-infimax for every
$\boldsymbol{{\it\alpha}}$-infimax for every  $\boldsymbol{{\it\alpha}}$ in a simplex of dimension 1 or greater. These results have applications to the theory of rotation sets of beta-shifts and torus homeomorphisms.
$\boldsymbol{{\it\alpha}}$ in a simplex of dimension 1 or greater. These results have applications to the theory of rotation sets of beta-shifts and torus homeomorphisms.