Published online by Cambridge University Press: 30 June 2016
We give a method for constructing a Legendrian representative of a knot in 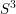 $S^{3}$ which realizes its maximal Thurston–Bennequin number under a certain condition. The method utilizes Stein handle decompositions of
$S^{3}$ which realizes its maximal Thurston–Bennequin number under a certain condition. The method utilizes Stein handle decompositions of  $D^{4}$ , and the resulting Legendrian representative is often very complicated (relative to the complexity of the topological knot type). As an application, we construct infinitely many knots in
$D^{4}$ , and the resulting Legendrian representative is often very complicated (relative to the complexity of the topological knot type). As an application, we construct infinitely many knots in 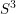 $S^{3}$ each of which yields a reducible 3-manifold by a Legendrian surgery in the standard tight contact structure. This disproves a conjecture of Lidman and Sivek.
$S^{3}$ each of which yields a reducible 3-manifold by a Legendrian surgery in the standard tight contact structure. This disproves a conjecture of Lidman and Sivek.