Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Levin, Aaron
and
Yasufuku, Yu
2018.
Integral points and orbits of endomorphisms on the projective plane.
Transactions of the American Mathematical Society,
Vol. 371,
Issue. 2,
p.
971.
Ghioca, Dragos
and
Satriano, Matthew
2018.
Density of orbits of dominant regular self-maps of semiabelian varieties.
Transactions of the American Mathematical Society,
Vol. 371,
Issue. 9,
p.
6341.
Ghioca, Dragos
and
Xie, Junyi
2018.
Algebraic dynamics of skew-linear self-maps.
Proceedings of the American Mathematical Society,
Vol. 146,
Issue. 10,
p.
4369.
Benedetto, Robert
Ingram, Patrick
Jones, Rafe
Manes, Michelle
Silverman, Joseph
and
Tucker, Thomas
2019.
Current trends and open problems in arithmetic dynamics.
Bulletin of the American Mathematical Society,
Vol. 56,
Issue. 4,
p.
611.
Javanpeykar, Ariyan
and
Xie, Junyi
2022.
Finiteness Properties of Pseudo-Hyperbolic Varieties.
International Mathematics Research Notices,
Vol. 2022,
Issue. 3,
p.
1601.
Xie, Junyi
2022.
The existence of Zariski dense orbits for endomorphisms of projective surfaces.
Journal of the American Mathematical Society,
Vol. 38,
Issue. 1,
p.
1.
Jia, Jia
Xie, Junyi
and
Zhang, De-Qi
2023.
Surjective endomorphisms of projective surfaces: the existence of infinitely many dense orbits.
Mathematische Zeitschrift,
Vol. 303,
Issue. 2,
Xie, Junyi
2023.
Remarks on algebraic dynamics in positive characteristic.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 0,
Issue. 0,
Bell, Jason
Ghioca, Dragos
and
Reichstein, Zinovy
2024.
Rational self-maps with a regular iterate on a semiabelian variety.
Journal of Number Theory,
Vol. 260,
Issue. ,
p.
103.
Xie, Junyi
2024.
Algebraicity Criteria, Invariant Subvarieties and Transcendence Problems from Arithmetic Dynamics.
Peking Mathematical Journal,
Vol. 7,
Issue. 1,
p.
345.
WANG, Long
2024.
Periodic points and arithmetic degrees of certain rational self-maps.
Journal of the Mathematical Society of Japan,
Vol. 76,
Issue. 3,
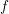 $f$ be a dominant polynomial endomorphism of the affine plane defined over an algebraically closed field of characteristic
$f$ be a dominant polynomial endomorphism of the affine plane defined over an algebraically closed field of characteristic 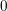 $0$. If there is no nonconstant invariant rational function under
$0$. If there is no nonconstant invariant rational function under  $f$, then there exists a closed point in the plane whose orbit under
$f$, then there exists a closed point in the plane whose orbit under  $f$ is Zariski dense. This result gives us a positive answer to a conjecture proposed by Medvedev and Scanlon, by Amerik, Bogomolov and Rovinsky, and by Zhang for polynomial endomorphisms of the affine plane.
$f$ is Zariski dense. This result gives us a positive answer to a conjecture proposed by Medvedev and Scanlon, by Amerik, Bogomolov and Rovinsky, and by Zhang for polynomial endomorphisms of the affine plane.