Published online by Cambridge University Press: 15 May 2020
Soient 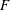 $F$
un corps global, et
$F$
un corps global, et 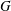 $G$
un groupe réductif connexe défini sur
$G$
un groupe réductif connexe défini sur  $F$
. On prouve que si deux données endoscopiques de
$F$
. On prouve que si deux données endoscopiques de 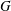 $G$
sont équivalentes en presque toute place de
$G$
sont équivalentes en presque toute place de 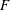 $F$
, alors elles sont équivalentes. Le résultat est encore vrai pour l’endoscopie (ordinaire) avec caractère. On donne aussi, pour
$F$
, alors elles sont équivalentes. Le résultat est encore vrai pour l’endoscopie (ordinaire) avec caractère. On donne aussi, pour  $F$
global ou local et
$F$
global ou local et 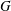 $G$
quasi-simple simplement connexe, une description des données endoscopiques elliptiques de
$G$
quasi-simple simplement connexe, une description des données endoscopiques elliptiques de 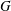 $G$
.
$G$
.
Let 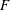 $F$
be a global field, and
$F$
be a global field, and 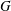 $G$
a connected reductive group defined over
$G$
a connected reductive group defined over 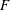 $F$
. We prove that two endoscopic data of
$F$
. We prove that two endoscopic data of 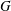 $G$
which are equivalent almost everywhere, are equivalent. The result remains true for (non-twisted) endoscopy with character. We also give, for
$G$
which are equivalent almost everywhere, are equivalent. The result remains true for (non-twisted) endoscopy with character. We also give, for 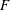 $F$
global or local and
$F$
global or local and 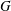 $G$
quasi-simple simply connected, a description of the elliptic endoscopic data of
$G$
quasi-simple simply connected, a description of the elliptic endoscopic data of 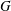 $G$
.
$G$
.